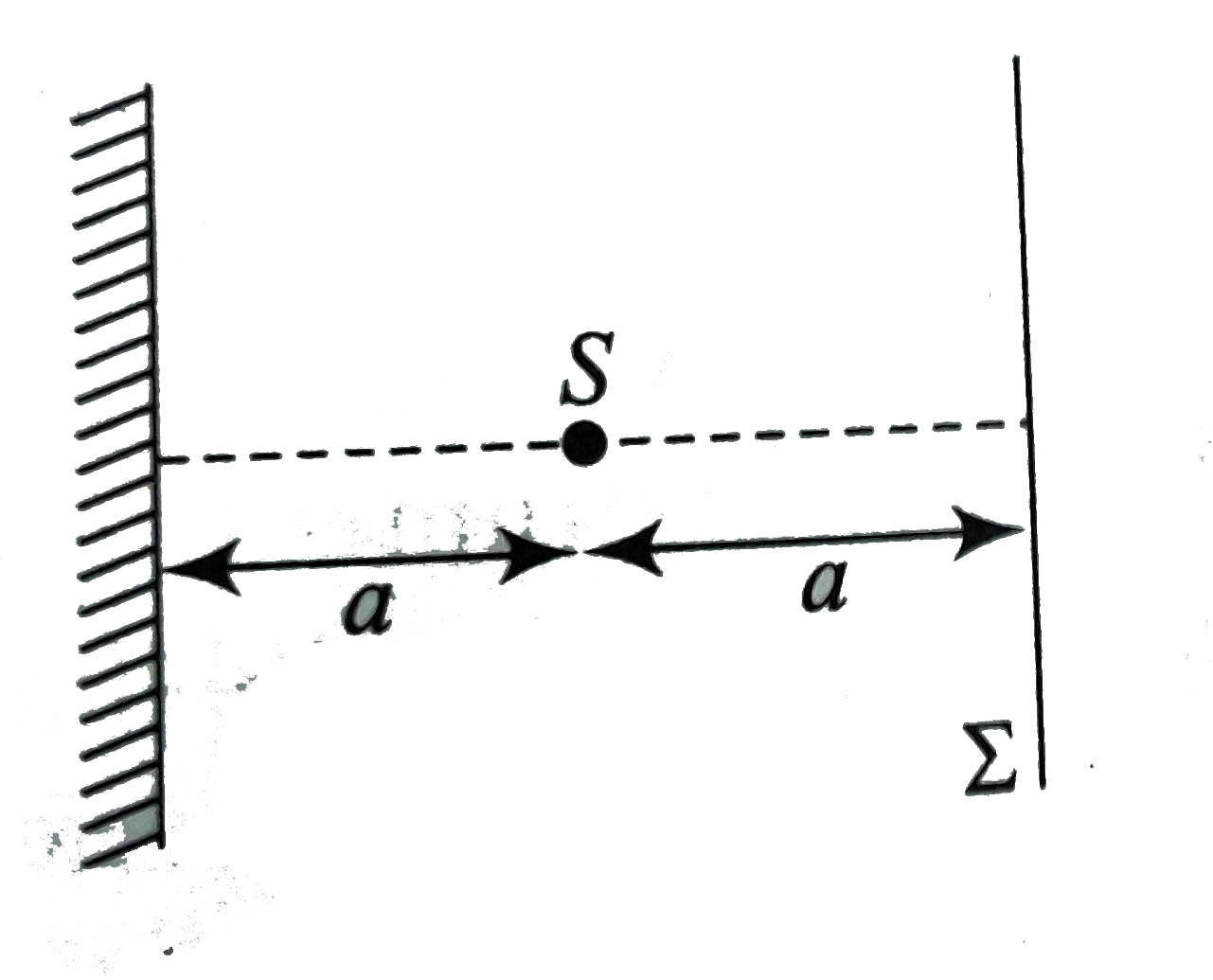
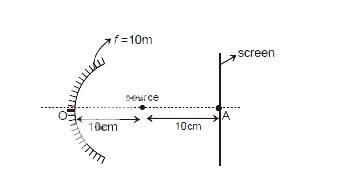A point source of light S is placed in front of a perfectly reflecting mirror as shown in Figure. Σ is a screen. The intensity at the center of screen is found to be I.
If the mirror is removed, then the intensity at the center of screen would be
I
10I/9
9I/10
2I
The correct Answer is:c.
Let poewr of light source be P, then intensity at any point on the screen it due to light rays directly received from source and that due to lilght rays after reflection from the mirror.
I=P4πa2+P4π×(3a)2
When mirror is taken away,
I1=P4πa2=9I10
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig2videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension51videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective26videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK1videos
FRICTION
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank39videos
GRAVITATION
Similar Questions
Knowledge Check
- Question 1 - Select One
A point source of light is placed in front of a plane mirror.
AAll the reflected rays meets at a point when produced backwardBony the reflected rays close to the normal meet at a point when produced backwardCony the reflected rays making a smal angle with the mirror meet at point when prodced backward.Dlight of different colours make different images - Question 2 - Select One
A point source of light is placed in front of a plane mirror as shown in Figure.
Determine the length of reflected path of light on the screen Σ
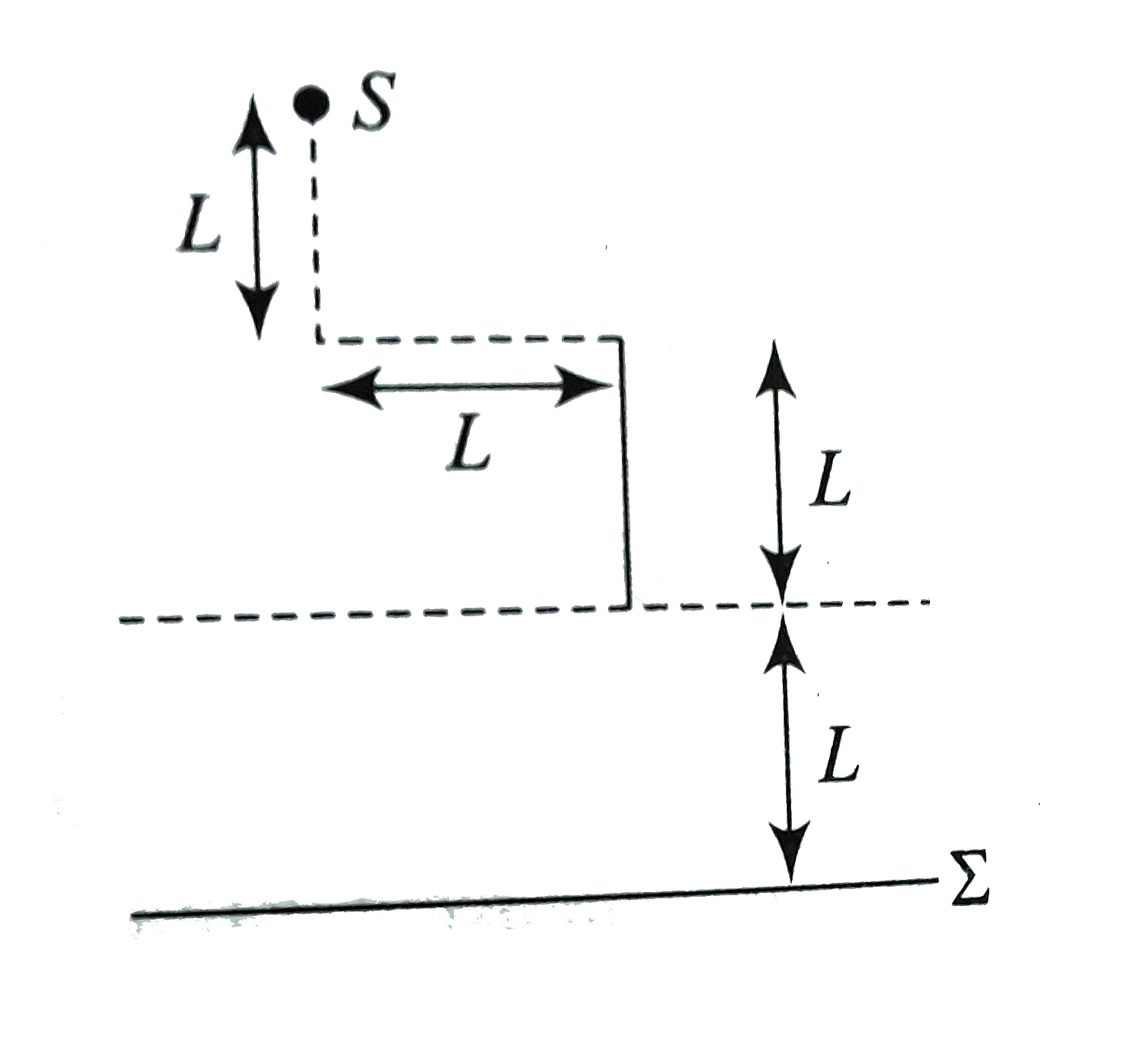 ALB2LC3L/2DL/2
ALB2LC3L/2DL/2 - Question 3 - Select One
Light from two coherent sources of the same amplitude A and wavelength λ illuminates the screen. The intensity of the central maximum is I0. If the sources were incoherent, the intensity at the same point will be
A4I0B2I0CI0DI02
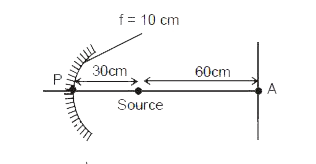
Intensity at A due to source is I. Without concave mirror, then find out the intensity of A after placing concave mirror.
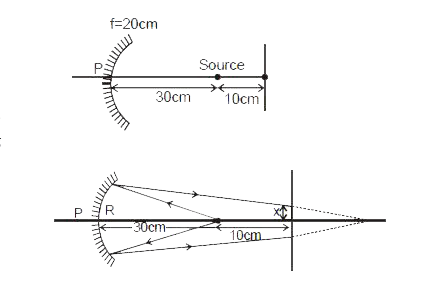 View Solution
View SolutionIn standard Young's double-slit experiment when sources of light are coherent, then intensity at the centre of the screen is found to be I1 and when sources are incoherent, then intensity at the centre is found to be I2. Calculate I1/I2.
0123456789View SolutionLight from two coherent sources of the same amplitude A and wavelength λ illuminates the screen. The intensity of the central maximum is I0. If the sources were incoherent, the intensity at the same point will be
View SolutionA point source of light 'S' at a distance d from the screen A produces light intensity I0 at the centre of the screen. If a completely reflecting mirror M is placed at a distance d behind the source as shown in the figure, find the intensity at the centre of the screen
 View Solution
View SolutionIf the distance between a point source and screen is doubled, then intensity of light on the screen will become
View Solution