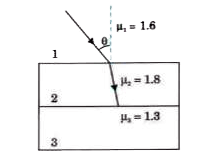
A ray of light refracts from medium 1 into a thin layer of medium 2, crosses the layer and is incident at the critical angle on the interface between the medium 2 and 3 as shown in the figure. If the angle of incidence of ray is θ, the values of θ is
sin−1(89)
sin−1(1318)
sin−1(1316)
sin−1(813)
The correct Answer is:C
Light enters from medium 1 to medium 2
sinθsinr=1μ2=μ2μ1 ...(A)
then again light falls from medium 2 to medium 3 at critical angle
sinrsin90∘=2μ3=μ3μ2
sinr=1.31.8
Put the value in equation (A) we get,
sinθ=1.81.6×1.31.8
⇒θ=sin−1(1316)
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:MODERN PUBLICATIONChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:OBJECTIVE TYPE QUESTIONS (JEE (ADVANCED) FOR IIT ENTRANCE)
Explore 20 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:MODERN PUBLICATIONChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:OBJECTIVE TYPE QUESTIONS (C. MULTIPLE CHOICE QUESTIONS)
Explore 25 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:MODERN PUBLICATIONChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:OBJECTIVE TYPE QUESTIONS (B. MULTIPLE CHOICE QUESTIONS)
Explore 21 Videos
- NUCLEIBook:MODERN PUBLICATIONChapter:NUCLEIExercise:CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
Explore 15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS Book:MODERN PUBLICATIONChapter:SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS Exercise:CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
Explore 12 Videos
Similar Questions
In figure, light refracts from material 1 into a thin layer of material 2, crosses that layer, and then is incident at the critical angle on the interface between materials 2 and 3.
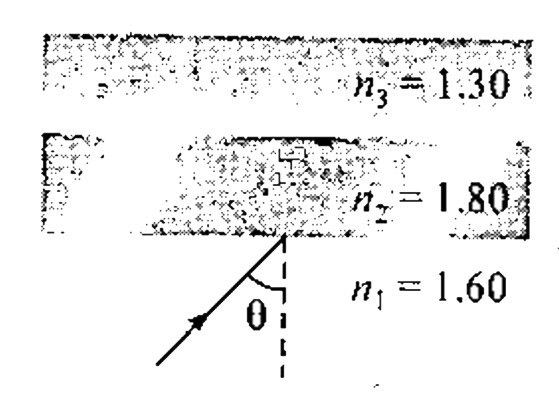
(a) What is the angle θ?
(b) If θ is decreased, is there refraction of light into material 3 ?
View SolutionIn figure, light refracts from material 1 to a thin layer of material 2, crosses that layer, and then is incident at the critical angle on the interface between materials 2 and 3.
(a) What is the angle θ?
(b) if θ is decreased, is there refraction of light into material 3?

View Solution
Knowledge Check
A ray of light passes from vaccum into a medium of refractive index n. If the angle of incidence is twice the angle of refraction, then the angle of incidence is
Acos−1(n/2)Bsin−1(n/2)C2cos−1(n/2)D2sin−1(n/2)A ray of light passes from vaccume into a medium of refractive index n. if the angle of incidence is twice the angle of refraction, then the angle of incidence is
Acos−1(n/2)Bsin−1(n/2)C2cos−1(n/2)D2sin−1(n/2)A ray of light passes from vacuum into a medium of refractive index n . If the angle of incidence is twice the angle of refraction , then the relation between the angle of incidence and the refractive index is
An=2sin(i2)Bn=2cos(i2)Cn=2tan(i2)Dn=12[cos(i2)]
A ray of light is incident as a normal ray on the surface of separation of two different mediums. What is the value of the angle of incidence in this case ?
View SolutionA ray of light passes from vaccum into a medium of refractive index n. If the angle of incidence is twice the angle of refraction, then the angle of incidence is
View SolutionA ray of light from a denser medium strikes a rarer medium at an angle of incidence i. if the reflected and the refracted rays are mutually perpendicular to each other, what is the value of the critical angle ?
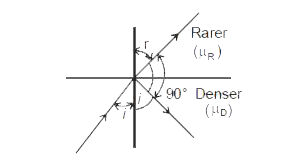
View SolutionA ray of light incident on a plane mirror at an angle θ. If the angle between the incident and reflected rays is 80∘, what is the value of θ?
View SolutionThe ray of light travels from raer medium to denser medium of refractive index μ. The angle of incidence of twice the angle of refraction. The angle of incidence is given by
View Solution
