A ray of light strikes a plane boundary separating two media, medium 1 and medium 2, at an angle of 45∘. Calculate the angle of refraction if the ray of light travels from medium 1 to medium 2. Given, refractive indices of medium 1 and 2 are 1.5 and 1.33 respectively.
To solve the problem of finding the angle of refraction when a ray of light travels from medium 1 to medium 2, we will use Snell's Law. Snell's Law states that:
μ1sin(i)=μ2sin(r)
Where:
- μ1 = refractive index of medium 1
- μ2 = refractive index of medium 2
- i = angle of incidence
- r = angle of refraction
1. Identify the Given Values:
- Refractive index of medium 1 (μ1) = 1.5
- Refractive index of medium 2 (μ2) = 1.33
- Angle of incidence (i) = 45 degrees
2. Convert the Angle of Incidence to Radians (if necessary):
- In this case, we can work directly with degrees since we will be using the sine function.
3. Apply Snell's Law:
- According to Snell's Law:
μ1sin(i)=μ2sin(r)
- Substitute the known values into the equation:
1.5sin(45∘)=1.33sin(r)
4. Calculate sin(45∘):
- sin(45∘)=√22≈0.7071
5. Substitute sin(45∘) into the Equation:
1.5×0.7071=1.33sin(r)
- This simplifies to:
1.06065≈1.33sin(r)
6. Solve for sin(r):
sin(r)=1.060651.33≈0.797
7. Calculate the Angle of Refraction r:
- To find r, take the inverse sine:
r=sin−1(0.797)≈53.13∘
Final Answer:
The angle of refraction r when the ray of light travels from medium 1 to medium 2 is approximately 53.13∘.
- μ1 = refractive index of medium 1
- μ2 = refractive index of medium 2
- i = angle of incidence
- r = angle of refraction
- Refractive index of medium 1 (μ1) = 1.5
- Refractive index of medium 2 (μ2) = 1.33
- Angle of incidence (i) = 45 degrees
- In this case, we can work directly with degrees since we will be using the sine function.
- According to Snell's Law:
μ1sin(i)=μ2sin(r)
- Substitute the known values into the equation:
1.5sin(45∘)=1.33sin(r)
- sin(45∘)=√22≈0.7071
1.5×0.7071=1.33sin(r)
- This simplifies to:
1.06065≈1.33sin(r)
sin(r)=1.060651.33≈0.797
- To find r, take the inverse sine:
r=sin−1(0.797)≈53.13∘
The angle of refraction r when the ray of light travels from medium 1 to medium 2 is approximately 53.13∘.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (1)
13 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (2)
9 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
Two different transparent media of different refractive indices are placed together as shown in the figure given below. A ray of light enters the medium settled above at an angle of 45∘. Calculate the angle of refraction of light in the medium settled below. The refractive indices of upper and lower media are 1.22 and 1.44, respectively.

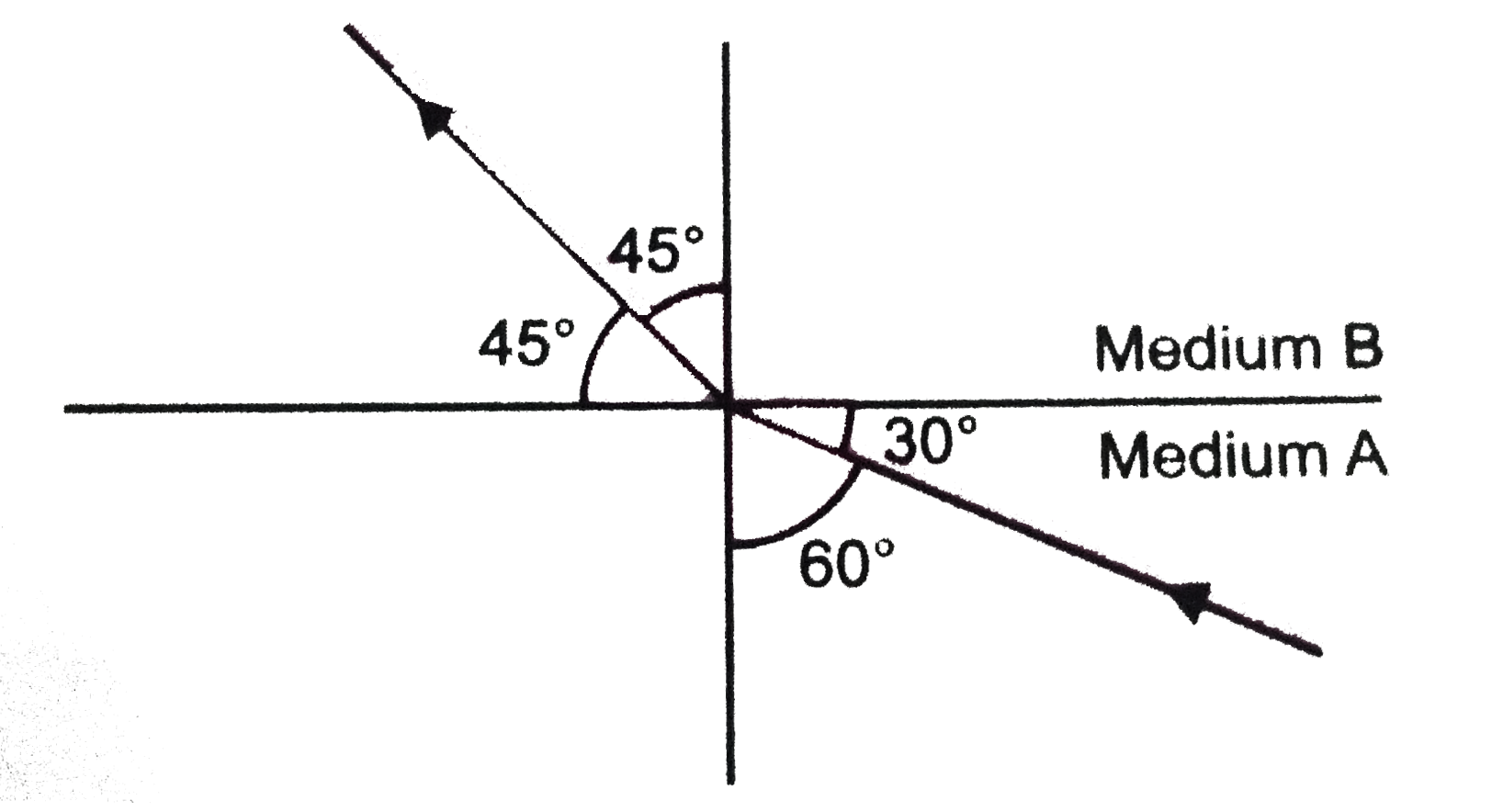
View SolutionFigure shows a ray of light as it travels from medium A to medium B. Refractive index of the medium B relative to medium A is
View Solution
Knowledge Check
A ray of light is travelling from medium A to medium B . The refractive index of the medium B does not depend upon
Anature of the mediumBwavelength of the light usedCnature of the medium ADangle of incidenceFigure shows a ray of light as it travels from medium A to medium B. Refractive index of the medium B relative to medium A is
 A√3/√2B√2/√3C1/√2D√2
A√3/√2B√2/√3C1/√2D√2Figure shows a ray of light as it travels from medium A to medium B. Refractive index of the medium B relative to medium A is
 A√3√2B√2√3C1√2D√2
A√3√2B√2√3C1√2D√2
When a ray of light travels from a denser medium to a rarer medium, the ray will :
View SolutionIf the ray of light travels from medium 1 to medium 2 and ultimately reverses its path then the phenomenon is _____
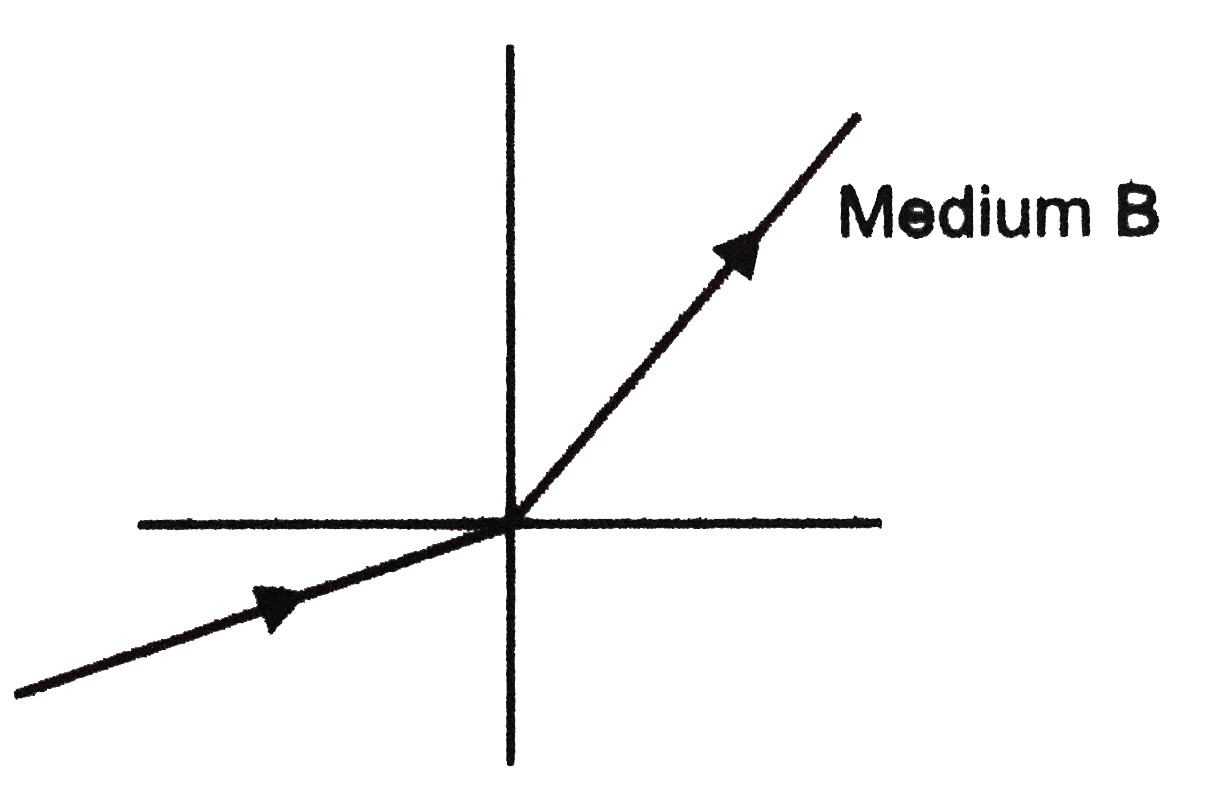
View SolutionA light ray enters from medium A to medium B as shown in Figure. The refractive index of medium A relative to B will be
View SolutionA light ray enters from medium A to medium B as shown in Figure. The refractive index of medium A relative to B will be

View SolutionA ray of light is incident on the surface of separation of a medium at an angle 45∘ and is refracted in the medium at an angle 30∘. What will be the speed of light in the medium?
View Solution
