A rectangular coil of area 5.0×10−4m2 and 60 turns is pivoted about one of its vertical sides. The coil is in a radial horizontal field of 90 G (radial here means the field liners are in the plane of the coil for any rotation). What is the torsional constant of the hair spring connected to the coil if a current of 2.0 mA produces an angular deflection of 18∘?
To solve the problem step by step, we will use the relationship between the magnetic torque on the coil, the current flowing through it, and the torsional constant of the spring.
Step 1: Identify the given values
- Area of the coil, A=5.0×10−4m2
- Number of turns, N=60
- Magnetic field strength, B=90G=90×10−4T (conversion from Gauss to Tesla)
- Current, I=2.0mA=2.0×10−3A
- Angular deflection, α=18∘
Step 2: Convert the angle from degrees to radians
To use the angle in calculations, we need to convert it to radians:
α=18∘×π180=18π180=π10radians
Step 3: Calculate the magnetic torque
The torque τ on the coil in a magnetic field is given by the formula:
τ=N⋅B⋅A⋅I
Substituting the values:
τ=60⋅(90×10−4)⋅(5.0×10−4)⋅(2.0×10−3)
Step 4: Calculate the torque
Calculating the above expression step by step:
1. Calculate 90×10−4:
90×10−4=0.009T
2. Calculate 60⋅0.009:
60⋅0.009=0.54
3. Calculate 5.0×10−4⋅2.0×10−3:
5.0×10−4⋅2.0×10−3=1.0×10−6
4. Now, multiply the results:
τ=0.54⋅1.0×10−6=5.4×10−7N m
Step 5: Relate torque to angular deflection and torsional constant
The relationship between torque, torsional constant k, and angular deflection α is given by:
τ=k⋅α
Rearranging to find k:
k=τα
Step 6: Substitute values to find the torsional constant
Substituting the values we calculated:
k=5.4×10−7π10
Calculating:
k=5.4×10−7⋅10π=5.4×10−6π≈5.4×10−63.14≈1.72×10−6N m/rad
Step 7: Convert to Newton meter per degree
Since 1rad=180πdegrees:
k≈1.72×10−6⋅π180≈3.0×10−8N m/degree
Final Answer
The torsional constant of the hair spring connected to the coil is approximately:
k≈3.0×10−8N m/degree
- Area of the coil, A=5.0×10−4m2
- Number of turns, N=60
- Magnetic field strength, B=90G=90×10−4T (conversion from Gauss to Tesla)
- Current, I=2.0mA=2.0×10−3A
- Angular deflection, α=18∘
To use the angle in calculations, we need to convert it to radians:
α=18∘×π180=18π180=π10radians
The torque τ on the coil in a magnetic field is given by the formula:
τ=N⋅B⋅A⋅I
Substituting the values:
τ=60⋅(90×10−4)⋅(5.0×10−4)⋅(2.0×10−3)
Calculating the above expression step by step:
1. Calculate 90×10−4:
90×10−4=0.009T
2. Calculate 60⋅0.009:
60⋅0.009=0.54
3. Calculate 5.0×10−4⋅2.0×10−3:
5.0×10−4⋅2.0×10−3=1.0×10−6
4. Now, multiply the results:
τ=0.54⋅1.0×10−6=5.4×10−7N m
The relationship between torque, torsional constant k, and angular deflection α is given by:
τ=k⋅α
Rearranging to find k:
k=τα
Substituting the values we calculated:
k=5.4×10−7π10
Calculating:
k=5.4×10−7⋅10π=5.4×10−6π≈5.4×10−63.14≈1.72×10−6N m/rad
Since 1rad=180πdegrees:
k≈1.72×10−6⋅π180≈3.0×10−8N m/degree
The torsional constant of the hair spring connected to the coil is approximately:
k≈3.0×10−8N m/degree
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Solved Example25videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.122videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based8videos
INDUCTANCE
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False3videos
MISCELLANEOUS VOLUME 3
Similar Questions
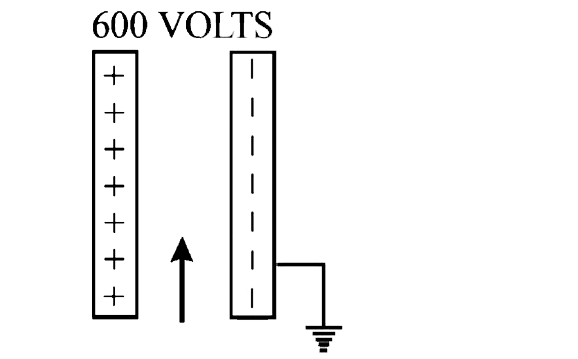
A potential difference of 600vo<s is applied across the plates of a parallel plate consenser . The separation between the plates is 3mm. An electron projected vertically, parallel to the plates , with a velocity of 2×106m/sec moves underflected between the plates. Find the magnitude and direction of the magnetic field in the region between the condenser plates. ( Neglect the edge effects). ( Charge of the electron = -1.6xx10^(-19) coulomb)
 02:33View Solution
02:33View SolutionA charged particle of mass 5 mg and charge q=+2μC has velocity →v=2ˆi−3ˆj+4ˆk. Find out the magnetic force on the charged particle and its acceleration at this instant due to magnetic field →B=3ˆj−2ˆk.→vand→B are in ms−1andWbm−2 , respectively.
02:18View SolutionLight is incident on a glass plate of refractive index 3.0 such that angle of refraction is 60° .Dark band is observed corresponding to the wavelength of 12000 A .If the thickness of glass plate is 1.2x10−3 mm. Calculate the order of the interference band for reflected system.
02:47View SolutionIn an electrolytic solution the electric current is mainly due to the movement of free electrons.
05:29View SolutionA rectangular coil of area 5.0xx10^-4m^2 and 60 turns is pivoted about one of its vertical sides. The coil is in a radial horizontal magnetic field of 9xx10^-3T . What is the torsional constant of the spring connected to the coil if a current of 0.20mA produces an angular diflection of 18^@ ?
05:17View SolutionA rectangular coil of area 5.0×10−4m2 and 60 turns is pivoted about one of its vertical sides. The coil is in a radial horizontal field of 90 G (radial here means the field liners are in the plane of the coil for any rotation). What is the torsional constant of the hair spring connected to the coil if a current of 2.0 mA produces an angular deflection of 18∘?
02:12View SolutionA rectangular coil of area 5.0 xx 10^(-4)m^(2) and 60 turns is pivoted about one of its vertical sides. The coil is in a radial horizontal magnetic field of 9 xx 10^(-3) T . What is the torsional constant of the spring connected to the coil if a current of 0.20mA produces an angular deflection of 180^(@) ?
View SolutionA rectangular coil ABCD which is rotated at a constant angular veolcity about an horizontal as shown in the figure. The axis of rotation of the coil as well as the magnetic field B are horizontal. Maximum current will flow in the circuit when the plane of the coil is
View SolutionA rectangular coil of area 5.0×10−4m2 and 40 turns is pivoted about one of its vertical sides. The coil is in a horizontal field of 80 gauss. If a current of 0.20 mA produces an angular deflection of 20∘, then the torsional constant of the hair spring connected to the coil is (in Nm/degree)
03:54View SolutionA rectangular coil of area 2 xx 10^(-4)m^(2) and 40 turns is pivoted about one of its vertical sides. The coil is in a radial horizontal field of 60G. What is the torsional constant of the hair springs connected to the coil if a current of 4.0 mA produces an angular diflection of 16^(@) ?
03:36View SolutionA moving coil galvanometer has a coil with 175 turns and area 1 cm^(2) . It uses a torsion band of torsion constant 10^(6) N-m/rad. The coil is placed in a magnetic field B parallel to its plane. The coil deflects by 1° for a current of 1 mA. The value of B (in Tesla) is approximately
09:07View SolutionA moving coil galvanometer has a rectangular wire coil of enclosed are 0.001 m2 and 500 turns. The coil operates in a radial magnetic field of 0.2 T and carries a current of 6π×10−810−8A. If the torsional spring constant is 6×10−7 N.m/r the angular deflection of the coil in radians is
02:58View SolutionA moving coil galvanometer has a rectangular wire coil of enclosed area 0.001 m2 and 500 turns. The coil operates in a radial mafnetic field of 0.2T and carries a current of 6π×10−8 A.
If the torsional spring constant is 6×10−7N− m/rad, then angular deflection of the coil in radians isView SolutionA moving coil galvanometer has a rectangular coil of area 10^(-3) m^(2) and 150 turns. The torsional constant of the hair springe connected to the coil is 5 xx 10^(-7) Nm per degree. The maximum angular deflection is 30^(@) . Calculate the maximum current that can be measured by it if its rectangular coil is kept in a radial horizontal magnetic field of 2,000 G .
03:35View Solution5.0xx10^(-4)m^(2) A rectangular coil with area and 60 turns is spun on one of its vertical arms. The radius of the coil is in the horizontal region of 90 G (here the mean of the radius is that the field lines are at the bottom of the coil for any orientation.) What is the torsional constant of the child command attached to the coil if a current of 0.20 A 18^(@) Produce an angular deflection of
02:20View SolutionA moving coil galvanometer has a coil with 175 turns and area 1 cm^(2) . It uses a torsion band of torsion constant 10^(-6) N-m//rad . The coil is placed in a magnetic field B parallel to its plane. The coil deflects by 1^(@) for a current of 1 mA. The value of B (in Tesla) is approximately.
06:27View Solution

