A ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(ii) Magnetic field produced at the centre of ring.
To find the magnetic field produced at the center of a rotating charged ring, we can follow these steps:
Step 1: Understand the problem
We have a ring with mass m, radius R, and charge q that is rotating about its axis with an angular velocity ω. We need to find the magnetic field B at the center of the ring.
Step 2: Use the formula for the magnetic field of a current-carrying loop
The magnetic field B at the center of a circular loop carrying current I is given by the formula:
B=μ0I2R
where μ0 is the permeability of free space and R is the radius of the ring.
Step 3: Determine the current I
Since the ring is charged and rotating, we need to find the equivalent current I. The current can be defined as the charge passing through a point per unit time. The time period T for one complete rotation of the ring is given by:
T=2πω
Thus, the current I can be expressed as:
I=qT=q2πω=qω2π
Step 4: Substitute I into the magnetic field formula
Now that we have the expression for current I, we can substitute it back into the magnetic field formula:
B=μ0I2R=μ0(qω2π)2R
Step 5: Simplify the expression
Now we simplify the expression:
B=μ0qω4πR
Final Result
Thus, the magnetic field produced at the center of the ring is:
B=μ0qω4πR
---
We have a ring with mass m, radius R, and charge q that is rotating about its axis with an angular velocity ω. We need to find the magnetic field B at the center of the ring.
The magnetic field B at the center of a circular loop carrying current I is given by the formula:
B=μ0I2R
where μ0 is the permeability of free space and R is the radius of the ring.
Since the ring is charged and rotating, we need to find the equivalent current I. The current can be defined as the charge passing through a point per unit time. The time period T for one complete rotation of the ring is given by:
T=2πω
Thus, the current I can be expressed as:
I=qT=q2πω=qω2π
Now that we have the expression for current I, we can substitute it back into the magnetic field formula:
B=μ0I2R=μ0(qω2π)2R
Now we simplify the expression:
B=μ0qω4πR
Thus, the magnetic field produced at the center of the ring is:
B=μ0qω4πR
Similar Questions
A ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(iii)Magnetic moment of ring.View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(i)Current produced due to motion of ringView Solution
Knowledge Check
- Question 1 - Select One or More
A non-conducting disc having unifrom positive charge Q, is rotating about its axis with unifrom angular velocity ω.The magnetic field at the centre of the disc is.
 Adirected outwardBhaving magnitude μ0Qω4πRCdirected inwardsDhaving magnitude μ0Qω2πR
Adirected outwardBhaving magnitude μ0Qω4πRCdirected inwardsDhaving magnitude μ0Qω2πR - Question 2 - Select One
A metallic ring of radius r with a uniform metallic spoke of negligible mass and length r is rotated about its axis with angular velocity ω in a perpendicular uniform magnetic field B as shown in Fig. 3.163. The central end of the spoke is connected to the rim of the wheel through a resistor R as shown. The resistor does not rotate, its one end is always at the center of the ring and the other end is always in as shown is needed to maintain constant angular velocity of the wheel. F is equal to (the ring and the spoke has zero resistance)
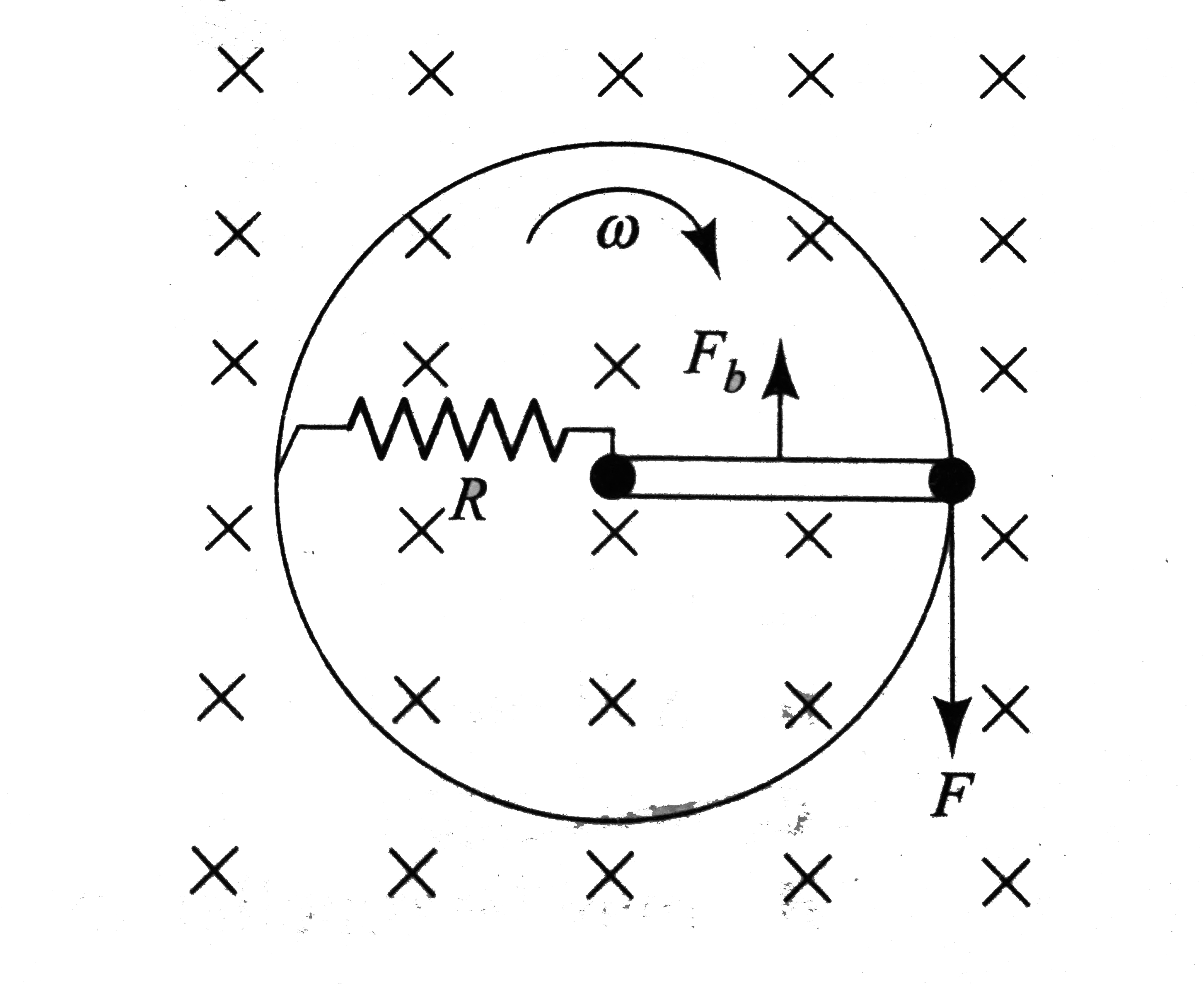 AB2ωr28RBB2ωr22RCB2ωr32RDB2ωr34R
AB2ωr28RBB2ωr22RCB2ωr32RDB2ωr34R - Question 3 - Select One
A thin circular ring of mass M and radius R is rotating about its axis with constant angular velocity ω. The objects each of mass m are attached gently to the ring. The wheel now rotates with an angular velocity.
AωM(m+M)Bω(M−2M)(M+2m)CωM(M+2m)Dω(M+2m)M
A ring of mass m and radius R is being rotated about its axis with angular velocity ω. If a increases then tension in ring
View SolutionA ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.
 View Solution
View SolutionA non-conducting thin disc of radius R and mass m having charge uniformly over one side with surface density σ rotates about its axis with an angular velocity ω. Find :
(a) the magnetic induction at the centre of the disc,
(b) the magnetic moment of the disc.
( c) the ratio of magnetic moment and angular momentum of disc.View SolutionA ring of mass M and radius R is rotating about its axis with angular velocity ω. Two identical bodies each of mass m are now gently attached at the two ends of a diameter of the ring. Because of this, the kinetic energy loss will be :
View Solution

