A ring of radius R is made of a thin wire of material of density rho,

Class 12 PHYSICS ELASTICITY

Topper's Solved these Questions

ELASTICITYBOOK - TARGET PUBLICATIONCHAPTER - ELASTICITYEXERCISE - Competitive Thinking
69 Videos
CURRENT ELECTRICITY BOOK - TARGET PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - Evaluation Test
6 Videos
ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EVALUATION TEST
8 Videos

Knowledge Check

A ring of radius R is made of a thin wire of material of density $ρ$ , having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is $ω$ .
The ratio of kinetic energy to potential energy is
A $\frac{y}{ρ R^{2} ω^{2}}$
B $\frac{2 y}{ρ R^{2} ω^{2}}$
C $\frac{y}{2 ρ R^{2} ω^{2}}$
D $\frac{y}{4 ρ R^{2} ω^{2}}$
A ring of radius $R$ is made of a thin wire of material of density $ρ$ having cross section area $a .$ The ring rotates with angular velocity $ω$ about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length $d l$ of angular width $d θ$ is shown in the figure.

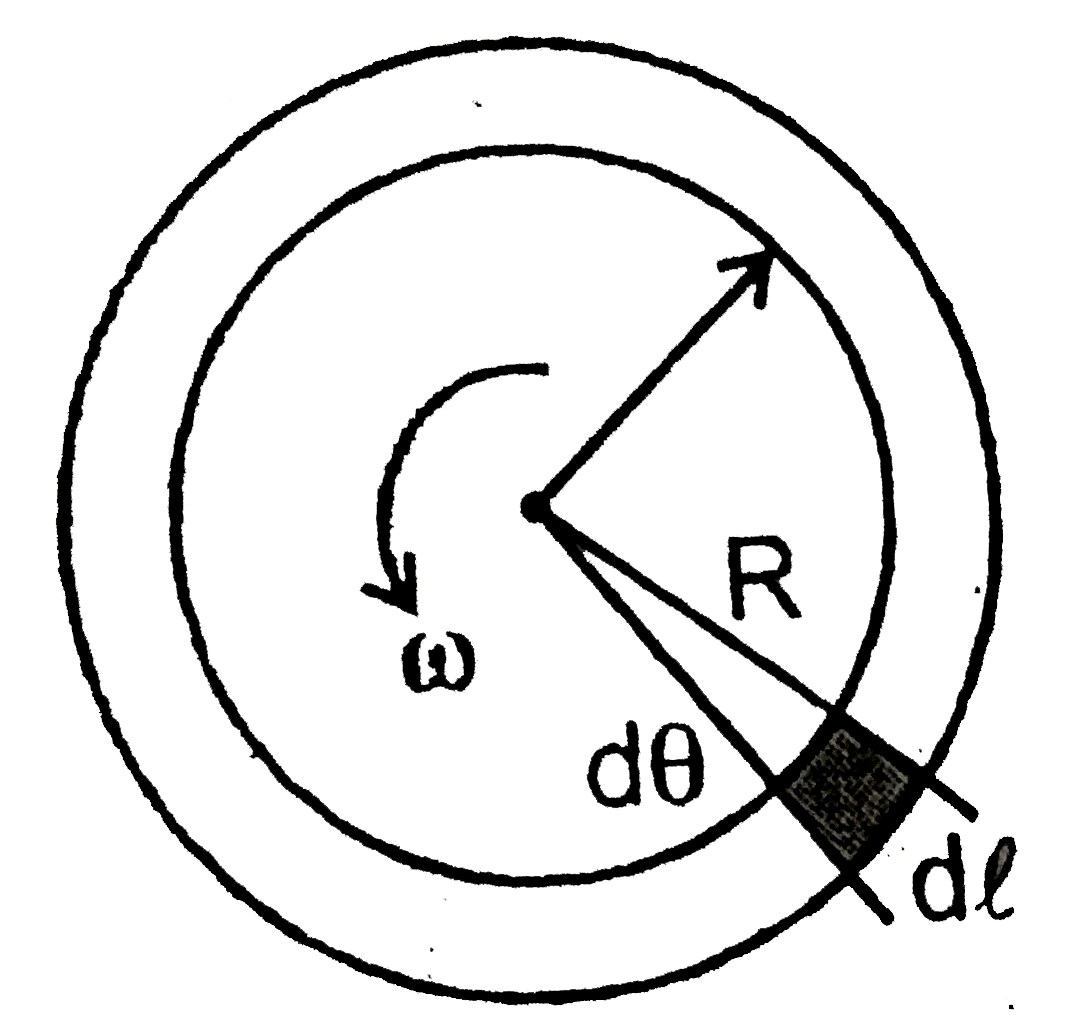
If $T$ is the tension in the ring, then
A $T = \frac{a ρ R^{2} ω^{2}}{2}$
B $T = a ρ R^{2} ω^{2}$
C $a^{2} ρ ω^{2}$
D $T = 2 a ρ R^{2} ω^{2}$
A ring of radius $R$ is made of a thin wire of material of density $ρ$ having cross section area $a .$ The ring rotates with angular velocity $ω$ about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length $d l$ of angular width $d θ$ is shown in the figure.

The centripetal force acting on the element is
A $(a . ρ d l ω^{2} R)$
B $R^{2} d θ . ω^{2}$
C $\frac{1}{2} a ρ d l ω^{2} R$
Dzero

A ring of radius R is made of a thin wire of material of density $ρ$ , having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is $ω$ .
The tension in the ring will be

The correct Answer is:B

Topper's Solved these Questions

Similar Questions

Knowledge Check

A ring of radius R is made of a thin wire of material of density $ρ$ , having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is $ω$ .
The ratio of kinetic energy to potential energy is

TARGET PUBLICATION-ELASTICITY-Evaluation test

A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω. The tension in the ring will be

The correct Answer is:B

Topper's Solved these Questions

Similar Questions

Knowledge Check

A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω. The ratio of kinetic energy to potential energy is

TARGET PUBLICATION-ELASTICITY-Evaluation test

A ring of radius R is made of a thin wire of material of density $ρ$ , having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is $ω$ .
The tension in the ring will be

A ring of radius R is made of a thin wire of material of density $ρ$ , having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is $ω$ .
The ratio of kinetic energy to potential energy is