A rod of mass m spins with an angular speed ω=√gl, Find its a. kinetic energy of rotation. b. kinetic energy of translation c. total kinetic energy.

Video Solution
Text Solution
Kinetic enegy of rotation Krotational=12ICω2
=12(ml212)(√gl)2=124mlgl
b Kinetic energy of translation, Krotational=12mv2C
=12m[(ωl2)2]=12m[√gl×l2]2=13mgl
c. total kinetic energy
Ktotal=Ktranslational+Krotational
=16mgl=12IPω2=12[ml22][√gl]2=16mgl
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.213videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.321videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Solved Examples12videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
Knowledge Check
- Question 1 - Select One
The rotational kinetic energy is -
Amω2B12Iα2C12Iω2D12mv2 - Question 2 - Select One
Rotational kinetic energy is equal to
A1w2BI2w2C32Iw2D12Iw2 - Question 3 - Select One
What fraction of translational kinetic energy of rolling circular disc to total kinetic energy ?
A1/3B1C2/3D1/2
Rotational Kinetic Energy || Examples
View SolutionA rod of mass m and length l is connected with a light rod of length l. The composite rod is made to rotate with angular velocity ω as shown in the figure. Find the
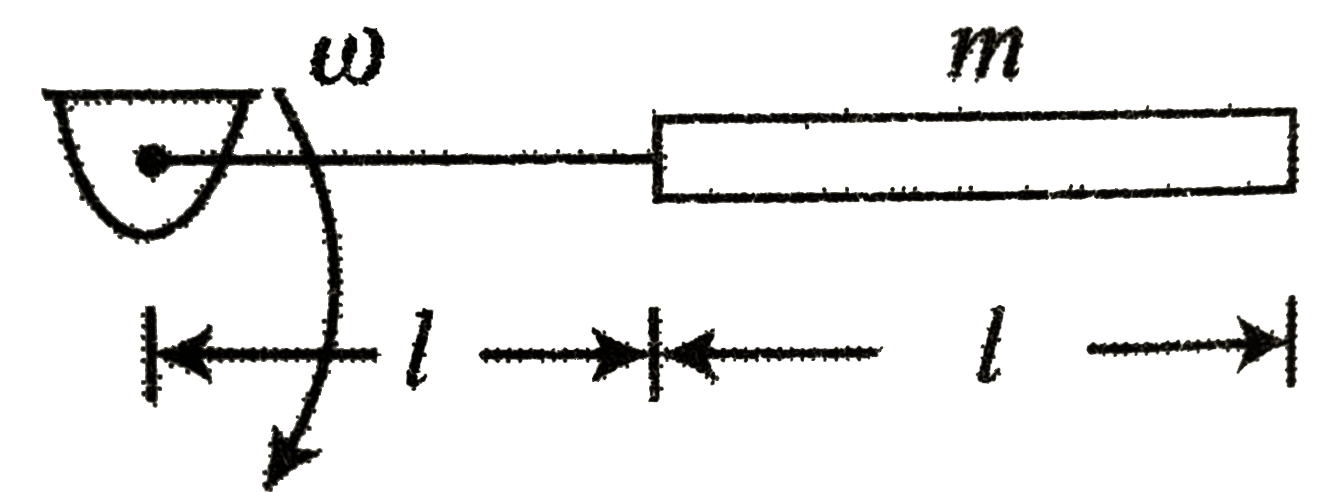
a. translational kinetic energy.
b. rotational kinetic energy.
c. total kinetic energy of rod.View SolutionWhen a sphere rolls without slipping the ratio of its kinetic energy of translation to its total kinetic energy is.
View SolutionA ring is rolling without slipping. Its energy of translation is E. Its total kinetic energy will be :-
View SolutionIf rotational kinetic energy is 50% of total kinetic energy then the body will be
View Solution

