A ship is sailing towards east in a traight line motion, with a speed of 10m/s . A man on the deck is moving across it , towards north, with a speed of 6m/s
calculate the velocity of the man relative to the sea.
To calculate the velocity of the man relative to the sea, we can follow these steps:
Step 1: Identify the velocities
- The velocity of the ship (Vs) is towards the east at 10 m/s. We can represent this as a vector:
→Vs=10^im/s
- The velocity of the man (Vm) is towards the north at 6 m/s. We can represent this as a vector:
→Vm=6^jm/s
Step 2: Determine the velocity of the man relative to the sea
The velocity of the man relative to the sea (V_m,s) can be calculated by adding the velocity of the man and the velocity of the ship:
→Vm,s=→Vm+→Vs
Substituting the values:
→Vm,s=6^j+10^i
Step 3: Calculate the magnitude of the resultant velocity
To find the magnitude of the resultant velocity vector, we use the Pythagorean theorem:
|→Vm,s|=√(10)2+(6)2
Calculating the squares:
|→Vm,s|=√100+36=√136
Calculating the square root:
|→Vm,s|≈11.66m/s
Step 4: Determine the direction of the velocity
To find the direction, we can calculate the angle θ with respect to the east (x-axis) using the tangent function:
tan(θ)=610
Calculating the angle:
θ=tan−1(610)≈31∘
Thus, the direction is 31 degrees north of east.
Final Answer
The velocity of the man relative to the sea is approximately:
11.66m/sat31∘north of east
---
- The velocity of the ship (Vs) is towards the east at 10 m/s. We can represent this as a vector:
→Vs=10^im/s
- The velocity of the man (Vm) is towards the north at 6 m/s. We can represent this as a vector:
→Vm=6^jm/s
The velocity of the man relative to the sea (V_m,s) can be calculated by adding the velocity of the man and the velocity of the ship:
→Vm,s=→Vm+→Vs
Substituting the values:
→Vm,s=6^j+10^i
To find the magnitude of the resultant velocity vector, we use the Pythagorean theorem:
|→Vm,s|=√(10)2+(6)2
Calculating the squares:
|→Vm,s|=√100+36=√136
Calculating the square root:
|→Vm,s|≈11.66m/s
To find the direction, we can calculate the angle θ with respect to the east (x-axis) using the tangent function:
tan(θ)=610
Calculating the angle:
θ=tan−1(610)≈31∘
Thus, the direction is 31 degrees north of east.
The velocity of the man relative to the sea is approximately:
11.66m/sat31∘north of east
Topper's Solved these Questions
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - PRACTICE PROBLEMS (1)
8 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - PRACTICE PROBLEMS (2)
9 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Chapter Practise Test
16 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
Similar Questions
A ship is steaming towards east with a speed of 8 m/s. A women runs across the deck at a speed of 6ms−1 towards north. What is the velocity of the women relative to the sea ?
View SolutionA plice siren emits a sound wave of frequency 440 Hz. The speed of sound is 332 m/s. if the siren is moving away from the listerner with a speed of 18 m/s relative to the air and the listerner is moving towards the siren with a speed of 68 m/s relative to the air, then what frequency does the listern hear?
View Solution
Knowledge Check
A train A is moving towards east with a velocity of 30 km/h and another train B is moving on parallel lines towards west with a speed of 40 km/ h . The velocity of train A relative to train B is
A10 km/hB70 km/h tosards eastC70 km/h towards westDnone of theseA car is going in south with a speed of 5m/s. To a man sitting in car a bus appears to move towards west with a speed of 2√6m/s. What is the actual speed of the bus?
A4ms−1B3ms−1C7ms−1Dnone of theseA man runs towards a mirror at a speed 15m/s. The speed of the image relative to the man is
A15ms−1B30ms−1C35ms−1D20ms−1
A police siren emits a sound wave of frequency 440 Hz. The speed of sound is 332 m/s. if the siren is moving away from the listerner with a speed of 18 m/s relative to the air and the listerner is moving towards the siren with a speed of 68 m/s relative to the air, then what frequency does the listern hear?
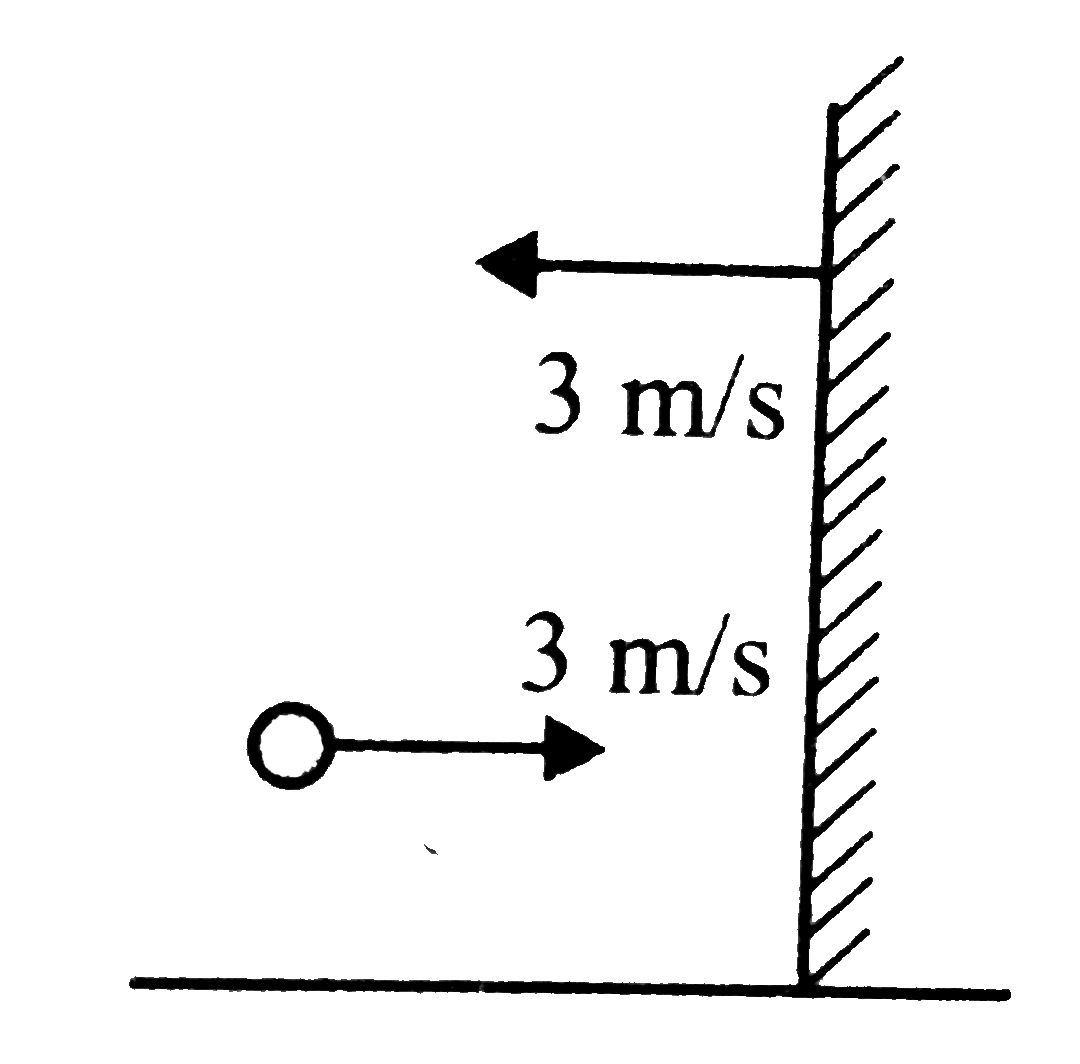
View SolutionA highly elastic ball moving at a speed of 3m/s approaches a wall moving towards it with a speed of 3m/s. After the collision. the speed of the ball will be
View SolutionA car is moving towards east with a speed of 25kmh−1. To the driver of the car, a bus appears to move towards north with a speed of 25√3kmh−1. What is the actual velocity of the bus ?
View SolutionA man in a balloon throws a stone downwards with a speed of 5m/s with respect to balloon. The balloon is moving upwards with a constant acceleration of 5m/s2. Then velocity of the stone relative to the man after 2 seconds is.

View SolutionDaniel walks directly toward a plane mirror at a speed of 0.25ms. Determine the speed of the image relative to him.
View Solution
