A simple pendulum of length 'L' has mass 'M' and it oscillates freely with amplitude energy is
(g = acceleration due to gravity)
MgA2l/(2L)
MgA2L
MgA2L
2MgA2L
The correct Answer is:A
Potential energy =12Mω2A2
=12M.gL.A2(∴ω=√gl)
Topper's Solved these Questions
- MH-CET - 2017 BOOK - NIKITA PUBLICATIONCHAPTER - MH-CET - 2017 EXERCISE - ELASTICITY
1 Videos
- MH-CET - 2017 BOOK - NIKITA PUBLICATIONCHAPTER - MH-CET - 2017 EXERCISE - SURFACE TENSION
2 Videos
- MH-CET - 2017 BOOK - NIKITA PUBLICATIONCHAPTER - MH-CET - 2017 EXERCISE - ROTATIONAL MOTION
3 Videos
- MCQS FROM BOARD EXAMBOOK - NIKITA PUBLICATIONCHAPTER - MCQS FROM BOARD EXAMEXERCISE - COMMUNICATION SYSTEMS
7 Videos
- MHT-CET 2016BOOK - NIKITA PUBLICATIONCHAPTER - MHT-CET 2016EXERCISE - COMMUNICATION SYSTEMS
1 Videos
Similar Questions
A mass of 5 kg is connected to a spring. The potential energy curve of the simple harmonic motion executed by the system is shown in the figure. A simple pendulum of length 4 m has the same period of oscillation as the spring system. What is the value of acceleration due to gravity on the planet where these experiments are performed ?
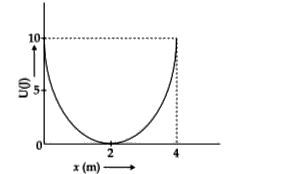
View SolutionA mass of 5 kg is connected to a spring. The potential energy curve of the simple harmonic motion executed by the system is shown in the figure. A simple pendulum of length 4 m has the same period of oscillation as the spring system. What is the value of acceleration due to gravity on the planet where these experiments are performed ?
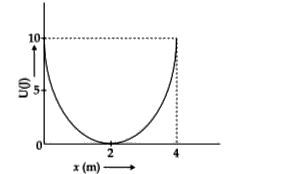
View Solution
Knowledge Check
A simple pendulum of length 'L' has mass 'M' and it oscillates freely with amplitude energy is
(g = acceleration due to gravity)AMgA22LBMgA2LCMgA2LD2MgA2LA simple pendulum of length l is made to oscillate with an amplitude of 45 degrees. The acceleration due to gravity is g. Let T0=2π√l/g . The time period of oscillation of this pendulum will be -
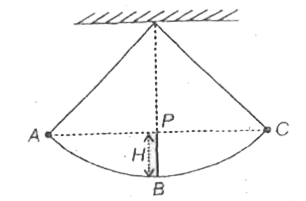
AT0 irrespective of the amplitudeBslightly less than T0Cslightly more than T0Ddependent on whether it swings in a plane aligned with the north-south or east-west directionsA simple pendulum with a bob of mass ‘m’ oscillates from A to C and back to A such that PB is H . If the acceleration due to gravity is ‘g’ then the velcoity of the bob as it passes through B is
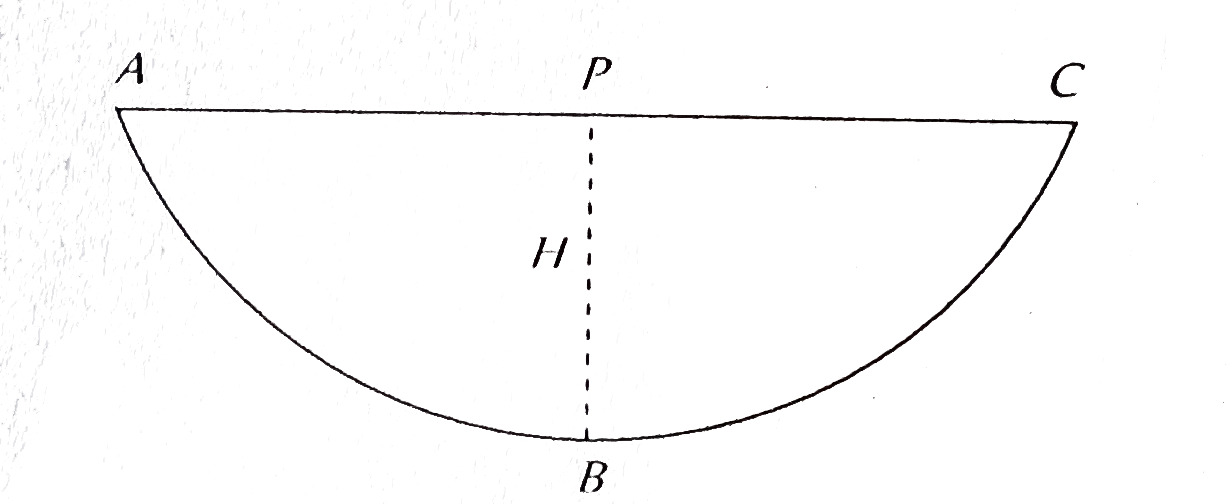 AmgHB√2gHC2ghDzero
AmgHB√2gHC2ghDzero
Attempt any SIX :
A simple pendulum of length 1 m and mass 10 g oscillates freely with amplitude 2 cm. Find its potential energy (P.E.) at the extreme position. (g=9.8m/s2)
View SolutionA simple pendulum with a bob of mass m oscillates from A to C and back to A, such that PB=h,
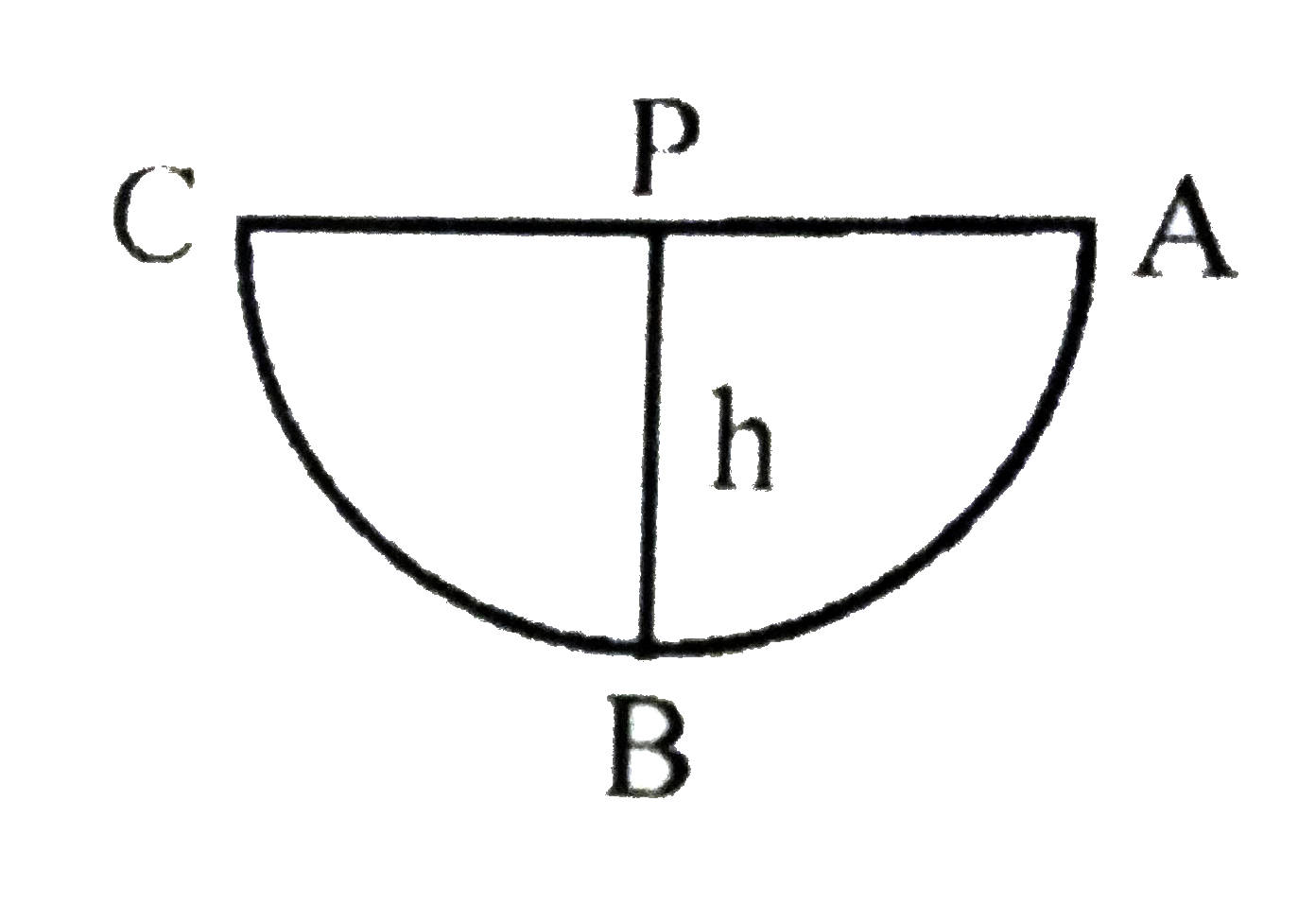
If the acceleration due to gravity is 'g', then the velocity of the bob as it passes through B is
View SolutionA simple pendulum with a bob of mass m oscillates from A to C and back to A such that PB is H. If the acceleration due to gravity is g, then the velocity of the bob as it passes through B is
View SolutionA simple pendulum of length 1 m, and energy 0.2 J, oscillates with an amplitude 4 cm. When its length is doubled then the energy of oscillation will be
View SolutionA simple pendulum of length 1 m with a bob of mass m swings with an angular amplitude 30°. Then (g = 9.8 ms−2)
View Solution
