A simple pendulum of length l is suspended from the celing of a cart which is sliding without friction on as inclined plane of inclination theta . What will be the time period of the pendulum?
To find the time period of a simple pendulum suspended from a cart sliding down an inclined plane, we can follow these steps:
1. Identify the Formula for Time Period:
The time period T of a simple pendulum is given by the formula:
T=2π√lgeffective
where l is the length of the pendulum and geffective is the effective acceleration due to gravity acting on the pendulum.
2. Determine the Effective Gravity:
In this scenario, the cart is sliding down an inclined plane with an angle θ. The effective acceleration due to gravity acting on the pendulum will be influenced by the inclination of the plane.
The gravitational force acting on the pendulum is mg (where m is the mass of the pendulum and g is the acceleration due to gravity). The components of this force can be resolved into two directions:
- Perpendicular to the incline: mgcosθ
- Parallel to the incline: mgsinθ
However, for the oscillation of the pendulum, we are interested in the component of gravity that acts perpendicular to the direction of the pendulum's swing. This component is given by:
geffective=gcosθ
3. Substitute geffective into the Time Period Formula:
Now, substituting geffective into the time period formula, we have:
T=2π√lgcosθ
4. Final Expression for the Time Period:
Therefore, the time period T of the pendulum when it is suspended from a cart sliding down an inclined plane is:
T=2π√lgcosθ
The time period T of a simple pendulum is given by the formula:
T=2π√lgeffective
where l is the length of the pendulum and geffective is the effective acceleration due to gravity acting on the pendulum.
In this scenario, the cart is sliding down an inclined plane with an angle θ. The effective acceleration due to gravity acting on the pendulum will be influenced by the inclination of the plane.
- Perpendicular to the incline: mgcosθ
- Parallel to the incline: mgsinθ
geffective=gcosθ
Now, substituting geffective into the time period formula, we have:
T=2π√lgcosθ
Therefore, the time period T of the pendulum when it is suspended from a cart sliding down an inclined plane is:
T=2π√lgcosθ
Similar Questions
A simple pendulum of length 5 m is suspended from the ceiling of a cart. Cart is sliding down on a frictionless surface having angle of inclination 60°. The time period of the pendulum is
View SolutionA simple pendulum is suspended from the ceiling of trolley which is sliding down on a inclined plane of inclination θ Find the angle made by the string with normal to the trolley a) when trolley slides down with uniform velocity b) when the plane is smooth.
View Solution
Knowledge Check
A simple pendulum of length I has been set up inside a railway wagon sliding down a frictionless inclined plane having an angle of inclination 0 with the horizontal. What will be its period of oscillation is recorded by an observer inside the wagon ? [Fig. 15.12.4]
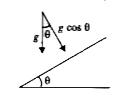 A2π√(lgcosθ)B2π√(lgsinθ)C2π√(lg)D2π√(lcosθg)
A2π√(lgcosθ)B2π√(lgsinθ)C2π√(lg)D2π√(lcosθg)A body of mass 100 g is sliding from an inclined plane of inclination 30∘. What is the frictional force experienced if μ=1.7 ?
A1.7×√2×(1/√3)NB1.7×√3×(1/2)NC1.7×√3 ND1.7×√2×(1/3) N
The period of oscillation of a simple pendulum of length L suspended from the roof of a vehicle which moves without frication down on inclined plane of inclination α=60∘ is given by π√XLg then find X.
View SolutionThe period of oscillation of a simple pendulum of length (L) suspended from the roof of a vehicle which moves without friction down an inclined plane of inclination (prop), is given by.
View SolutionA pendulum of mass m and length l is suspended from the ceiling of a trolley which has a constant acceleration a in the maximum deflection θ of the pendulum from the vertical.
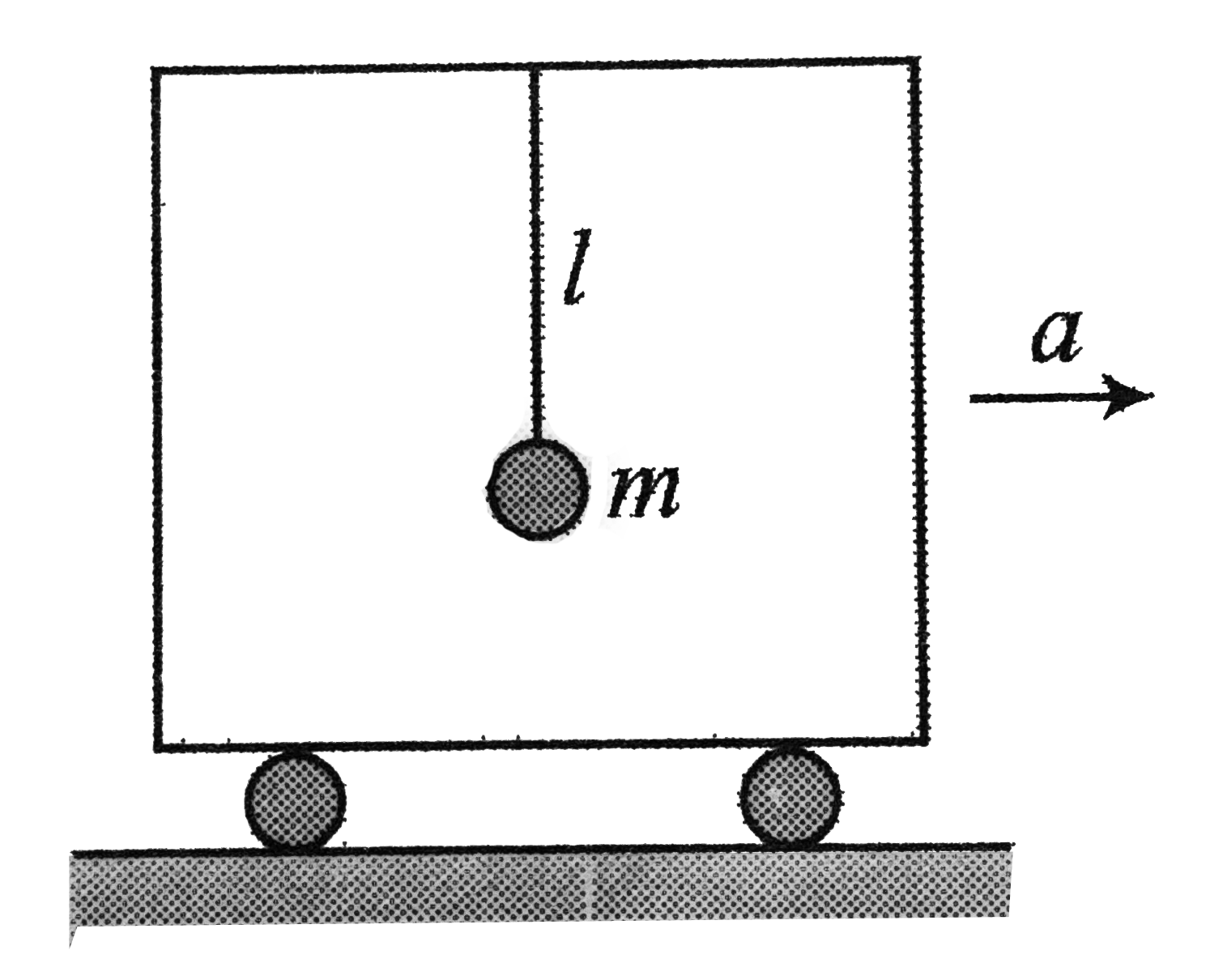
View SolutionWhat is the minimum coefficient of friction for a solid sphere to roll without slipping on an inclined plane of inclination θ ?
View SolutionA pendulum is hanging from the ceiling of a trolley which moves down a smooth inclined plane. Then
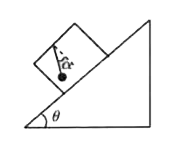
View SolutionA simple pendulum of length l and mass m is suspended in a car that is moving with constant speed v around a circle of radius r. Find the period of oscillation and equilibrium position of the pendulum.
View Solution
