A sinusoidal wave is propagating in negative x-direction in a string stretched along x-axis. A particle of string at x=2 cm is found at its mean position and it is moving in positive y-direction at t=1 s. the amplitude of the wave, the wavelength and the angular frequency of the wave are 0.1m,π/4m and 4πrad/s, respectively.
The speed of particle at x=2m and t=1s is
0.2πm/s
0.6πm/s
0.4πm/s
0
The correct Answer is:c
To solve the problem, we will follow these steps:
Step 1: Identify the given parameters
We have the following parameters from the problem statement:
- Amplitude (A) = 0.1 m
- Wavelength (λ) = π/4 m
- Angular frequency (ω) = 4π rad/s
- The position of the particle (x) = 2 cm = 0.02 m (converted to meters)
- Time (t) = 1 s
Step 2: Calculate the wave number (k)
The wave number (k) can be calculated using the formula:
k=2πλ
Substituting the value of λ:
k=2ππ4=8m−1
Step 3: Calculate the frequency (f)
The frequency (f) can be calculated using the relationship between angular frequency and frequency:
ω=2πf
Rearranging gives:
f=ω2π
Substituting the value of ω:
f=4π2π=2Hz
Step 4: Calculate the speed of the wave (v)
The speed of the wave can be calculated using the formula:
v=f⋅λ
Substituting the values of f and λ:
v=2⋅π4=π2m/s
Step 5: Determine the velocity of the particle at x = 2 m and t = 1 s
Since the particle at x = 2 cm (0.02 m) is at its mean position, we can conclude that the particle at x = 2 m is also at its mean position (as it is an integral multiple of the wavelength). At the mean position, the particle has maximum velocity, which is given by:
vmax=A⋅ω
Substituting the values of A and ω:
vmax=0.1⋅4π=0.4πm/s
Final Answer
The speed of the particle at x = 2 m and t = 1 s is:
v=0.4πm/s
---
We have the following parameters from the problem statement:
- Amplitude (A) = 0.1 m
- Wavelength (λ) = π/4 m
- Angular frequency (ω) = 4π rad/s
- The position of the particle (x) = 2 cm = 0.02 m (converted to meters)
- Time (t) = 1 s
The wave number (k) can be calculated using the formula:
k=2πλ
Substituting the value of λ:
k=2ππ4=8m−1
The frequency (f) can be calculated using the relationship between angular frequency and frequency:
ω=2πf
Rearranging gives:
f=ω2π
Substituting the value of ω:
f=4π2π=2Hz
The speed of the wave can be calculated using the formula:
v=f⋅λ
Substituting the values of f and λ:
v=2⋅π4=π2m/s
Since the particle at x = 2 cm (0.02 m) is at its mean position, we can conclude that the particle at x = 2 m is also at its mean position (as it is an integral multiple of the wavelength). At the mean position, the particle has maximum velocity, which is given by:
vmax=A⋅ω
Substituting the values of A and ω:
vmax=0.1⋅4π=0.4πm/s
The speed of the particle at x = 2 m and t = 1 s is:
v=0.4πm/s
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning
6 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A sinusoidal wave is propagating in negative x-direction in a string stretched along x-axis. A particle of string at x=2 cm is found at its mean position and it is moving in positive y-direction at t=1 s. the amplitude of the wave, the wavelength and the angular frequency of the wave are 0.1m,π/4m and 4πrad/s, respectively.
The equation of the wave is
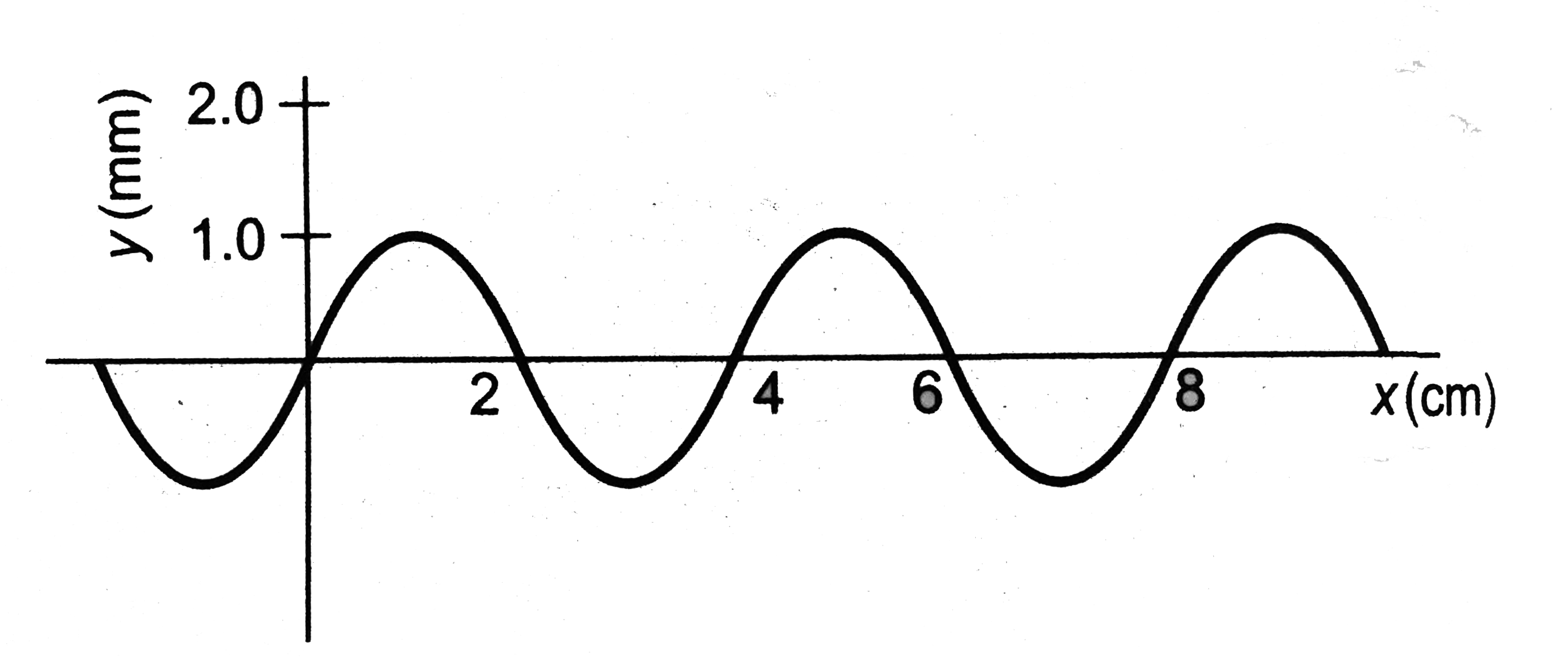
View SolutionFigure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is 20cms−1. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .
.
View SolutionFigure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
View SolutionThe position of a particle moving along x-axis varies eith time t as x=4t−t2+1. Find the time interval(s) during which the particle is moving along positive x-direction.
View SolutionA sinusoidal wave trsvelling in the positive direction on a stretched string has amplitude 2.0cm, wavelength 1.0m and velocity 5.0m/s. At x=0 and t=0 it is given that y=0 and ∂y∂t<0. Find the wave function y(x,t).
View SolutionA sinusoidal wave travels along a taut string of linear mass density 0.1g/cm. The particles oscillate along y− direction and wave moves in the positive x− direction . The amplitude and frequency of oscillation are 2mm and 50Hz respectively. The minimum distance between two particles oscillating in the same phase is4m.
If at x=2m and t=2s, the particle is at y=1mm and its velocity is in positive y− direction, then the equation of this travelling wave is :(y is in mm,t is in seconds and x is in metres )
View SolutionA progressive wave having amplitude 5m and wavelength 3m. If the maximum average velocity of particle in half time period is 5m/s and waves is moving in the positive x-direction then find which may be the correct equation (s) of the wave ? [wave x in meter].
View SolutionThe position of a transverse wave travelling in medium along positive x-axis is shown in figure at time t=0. Speed of wave is v=200 m/s
Frequency of the wave is

View SolutionA transverse wave of amplitude 2 m, wavelength 4 m and frequency 6 Hz is propagating in a string toward positive y-direction. The equation of the wave may be (y is in m and t is in s)
View SolutionThe displacement of A particle at x = 0 of a stretched string carrying a wave in the positive X-direction is given by f(t)=Ae−t2. The wave speed is V. Write equation of the wave.
View Solution
