A small ball of mass m and density ρ is dropped in a viscous liquid of density σ. Ather some time the ball falls with a constant velocity. Calculate the viscous force acting on the ball.
To solve the problem of calculating the viscous force acting on a small ball of mass m and density ρ that is dropped in a viscous liquid of density σ, we follow these steps:
Step 1: Understand the Forces Acting on the Ball
When the ball is dropped, it experiences two main forces:
1. The gravitational force (weight) acting downwards, given by Fg=mg.
2. The viscous force acting upwards, which we need to calculate.
Step 2: Establish Terminal Velocity Condition
When the ball reaches a constant velocity (terminal velocity), the net force acting on it becomes zero. This means that the downward gravitational force is balanced by the upward viscous force:
Fg=Fviscous
Step 3: Write the Equation for Forces
At terminal velocity:
mg=Fviscous
Step 4: Calculate the Volume of the Ball
The volume V of the ball can be expressed in terms of its mass m and density ρ:
V=mρ
Step 5: Calculate the Weight of the Liquid Displaced
According to Archimedes' principle, the weight of the liquid displaced by the ball is equal to the weight of the volume of liquid that the ball displaces. The mass of the liquid displaced M′ is given by:
M′=σV=σ(mρ)
Thus, the weight of the displaced liquid is:
Fb=M′g=σ(mρ)g
Step 6: Set Up the Equation for Viscous Force
Using the relationship established in Step 2, we can equate the two forces:
mg=σ(mρ)g+Fviscous
Rearranging gives us:
Fviscous=mg−σ(mρ)g
Step 7: Factor Out Common Terms
Factoring out mg from the right side:
Fviscous=mg(1−σρ)
Conclusion
Thus, the viscous force acting on the ball when it reaches terminal velocity is:
Fviscous=mg(1−σρ)
When the ball is dropped, it experiences two main forces:
1. The gravitational force (weight) acting downwards, given by Fg=mg.
2. The viscous force acting upwards, which we need to calculate.
When the ball reaches a constant velocity (terminal velocity), the net force acting on it becomes zero. This means that the downward gravitational force is balanced by the upward viscous force:
Fg=Fviscous
At terminal velocity:
mg=Fviscous
The volume V of the ball can be expressed in terms of its mass m and density ρ:
V=mρ
According to Archimedes' principle, the weight of the liquid displaced by the ball is equal to the weight of the volume of liquid that the ball displaces. The mass of the liquid displaced M′ is given by:
M′=σV=σ(mρ)
Thus, the weight of the displaced liquid is:
Fb=M′g=σ(mρ)g
Using the relationship established in Step 2, we can equate the two forces:
mg=σ(mρ)g+Fviscous
Rearranging gives us:
Fviscous=mg−σ(mρ)g
Factoring out mg from the right side:
Fviscous=mg(1−σρ)
Thus, the viscous force acting on the ball when it reaches terminal velocity is:
Fviscous=mg(1−σρ)
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Long Answer questions
33 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Solved Examples
159 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Very short answer questions
163 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
Similar Questions
The velocity of a small ball of mass M and density d when dropped in a container filled with glycerine becomes constant after sometime. If the density of glycerine is 'd/2' then the viscous force acting on the ball will be
View SolutionA spherical ball of radius r and density d is dropped from rest in a viscous fluid having density ρ and coefficient of viscosity η.
(a) Calculate the power (P1) of gravitational force acting on the ball at a time t after it is dropped. (b) Calculate the rate of heat generation (P2) due to rubbing of fluid molecules with the ball, at time t after it is dropped. (c) How do P1 and P2 change if the radius of the ball were doubled? (d) Find P1 and P2 when both become equal.
View Solution
Knowledge Check
A tiny sphere of mass m and density x is dropped in a jar of glycerine of density y. When the sphere aquires terminal velocity, the magnitude of the viscous force acting on it is :
AmgxyBmgyxCmg[1−yx]Dmg[1+yx]The velocity of small ball of mass M and density d1 when dropped in a container filled with glycerin becomes constant after sometime. If the density glycerin of is d2, the viscous force acting on ball is
AMd1gd2BMg(1−d2d1)CM(d1+d2)gDMd1d2A spherical ball of density σ is freely falling in a viscous liquid of density p(p<σ). The initial acceleration of the ball will be
A(p−σp)gB(σ−pσ)gC(p−σσ)gD(p+σσ)g
A ball of density ρ is released from deep inside of a liquid of density 2ρ. It will move up
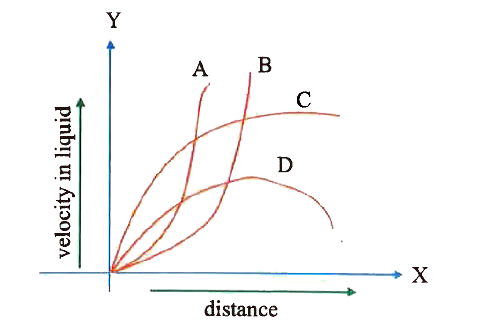
View SolutionA small ball is dropped in a viscous liquid. Its fall in the liquid is best described by the figure
View SolutionA ball of density ρ is dropped from a height on the suraca of a non-visous liquid of dinsity 2ρ. Choose the correct options.

View SolutionStatement -1 : A tiny shere of mass m and density ρ is dropped in a tall jar of glycerine of density ρ0 . When the sphere acquires terminal velocity v, the magnitude of viscous force is mg(1−ρ0/ρ).
Statement -2 : Viscous force on the body falling in a medium is F=6πηrv , where η is the coeff. of viscosity of medium and r is the radius of body
View SolutionThe velocity of small ball of mass M and density d1 when dropped a container filled with glycerine becomes constant after some time. If the density of glycerine is d2, the viscous force acting on ball is
View Solution
