A small object, which has a charge q=7.5μC and mass m=9.0×10−5kg, is placed in a constant electric field. Starting from rest, the object accelerates to a speed of 2.0×103m/s in a time of 0.96 s. Determine the magnitude of the electric field.
2.5×104N/C
4.5×104N/C
3.4×104N/C
1.7×104N/C
The correct Answer is:A
To determine the magnitude of the electric field acting on the charged object, we can follow these steps:
Step 1: Calculate the acceleration of the object
The object starts from rest and reaches a speed of v=2.0×103m/s in a time t=0.96s. The formula for acceleration a is given by:
a=v−ut
where u is the initial velocity (which is 0 since it starts from rest).
Substituting the values:
a=2.0×103m/s−00.96s=2.0×1030.96≈2083.33m/s2
Step 2: Relate the acceleration to the electric field
The only force acting on the charged object is due to the electric field. According to Newton's second law, the force F acting on the object can be expressed as:
F=m⋅a
where m is the mass of the object. The force due to the electric field E is given by:
F=q⋅E
where q is the charge of the object. Setting these two expressions for force equal gives:
m⋅a=q⋅E
Step 3: Solve for the electric field E
Rearranging the equation to solve for E:
E=m⋅aq
Step 4: Substitute the known values
The mass m=9.0×10−5kg and the charge q=7.5μC=7.5×10−6C. Now substituting the values into the equation:
E=(9.0×10−5kg)⋅(2083.33m/s2)7.5×10−6C
Calculating the numerator:
9.0×10−5⋅2083.33≈0.1875N
Now substituting this value into the equation for E:
E=0.1875N7.5×10−6C≈25000N/C=2.5×104N/C
Final Result
The magnitude of the electric field is approximately:
E≈2.5×104N/C
---
The object starts from rest and reaches a speed of v=2.0×103m/s in a time t=0.96s. The formula for acceleration a is given by:
The only force acting on the charged object is due to the electric field. According to Newton's second law, the force F acting on the object can be expressed as:
Rearranging the equation to solve for E:
The mass m=9.0×10−5kg and the charge q=7.5μC=7.5×10−6C. Now substituting the values into the equation:
The magnitude of the electric field is approximately:
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (More than one correct Type )3videos
ELECTRIC CHARGES AND FIELDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Linked Comprehension)5videos
ELECTRIC CHARGES AND FIELDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Problems63videos
ELECTRIC CHARGES AND FIELDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
ELASTICITY
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type1videos
ELECTRIC POTENTIAL
Similar Questions
The electric force experienced by a charge of 1.0xx10^(-6) C is 1.5 xx 10^(-3) N . Find the magnitude of the electric field at the position of the chaerge.
View SolutionAn electron enters an electric field of width 40μ m with a speed of 6×103m/s and takes 6ns to cross it. Find its acceleration and velocity just after it exists the field.
View Solution
Knowledge Check
- Question 1 - Select One
Ratio of electric and magnetic field due to moving point charge if its speed is 4.5×105m/s
A2×1011B3×1011C2×108D3×1012 - Question 2 - Select One
A particle of mass 2×10−3 kg, charge 4×10−3C enters in an electric field of 5V/m, then its kinetic energy after 10 s is
A0.1 JB1 JC10 JD100 J - Question 3 - Select One
A charge q=−2.0μC is placed at origin. Find the electric field at (3m,4m,0).
A−(4.32ˆi+5.76ˆj)×102N/CB−(4.32ˆi−5.76ˆj)×102N/CC−(5.76ˆi+4.32ˆj)×102N/CD(4.32ˆi+5.76ˆj)×102N/C
A particle having a charge+3×10−9C is placed in a uniform electric field directed toward left. It is released from rest and moves a distance of 5 cm after which its kinetic energy is found to be 4.5×10−5J.
(a) Calcnalate the work done by the electrical force on the particle
(b) Calculate the magnitude of the electric field.
(c) Calculate the potential of starting point with respect to the end point of particle's motion.
 View Solution
View SolutionA charged water drop of weight 4×10−18 kg and charge 1.6×10−19 C is stable in an electric field . What is the intensity of electric field
View SolutionFigure shows a point charge of 0.5×10−6 C at the center of the spherical cavity of radius 3 cm of a piece of metal. The electric field at
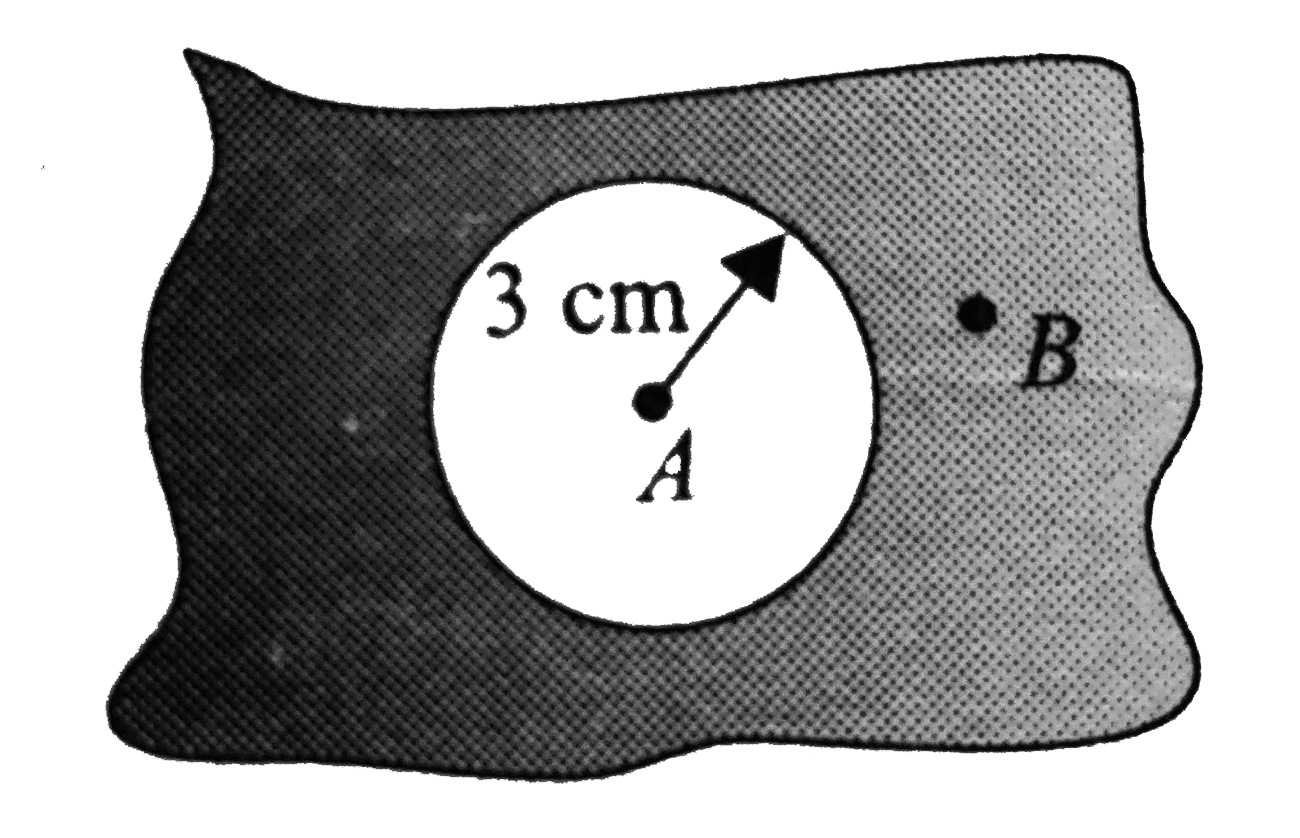 View Solution
View SolutionAn electron has a mass of 9.1×10−31kg. It revolves round the nucleus in a circular orbit of radius 0.529×10−10 metre at a speed of 2.2×106m/s. The magnitude of its linear momentum in this motion is
View SolutionIn Millikan's oil drop experiment, an oil drop mass 60×10−6kg is balanced by an electric field of 106V/m. The charge in coulomb on the drop, assuming g=10m/s2 is
View Solution

