A small spehre of mass m is connected by a stirng to a nail at O and moves in a circle of radius r on the smooth plane inclined at an angle θ with the horizontal. If the sphere has a velocity u at the top position A. Mark the correct options.
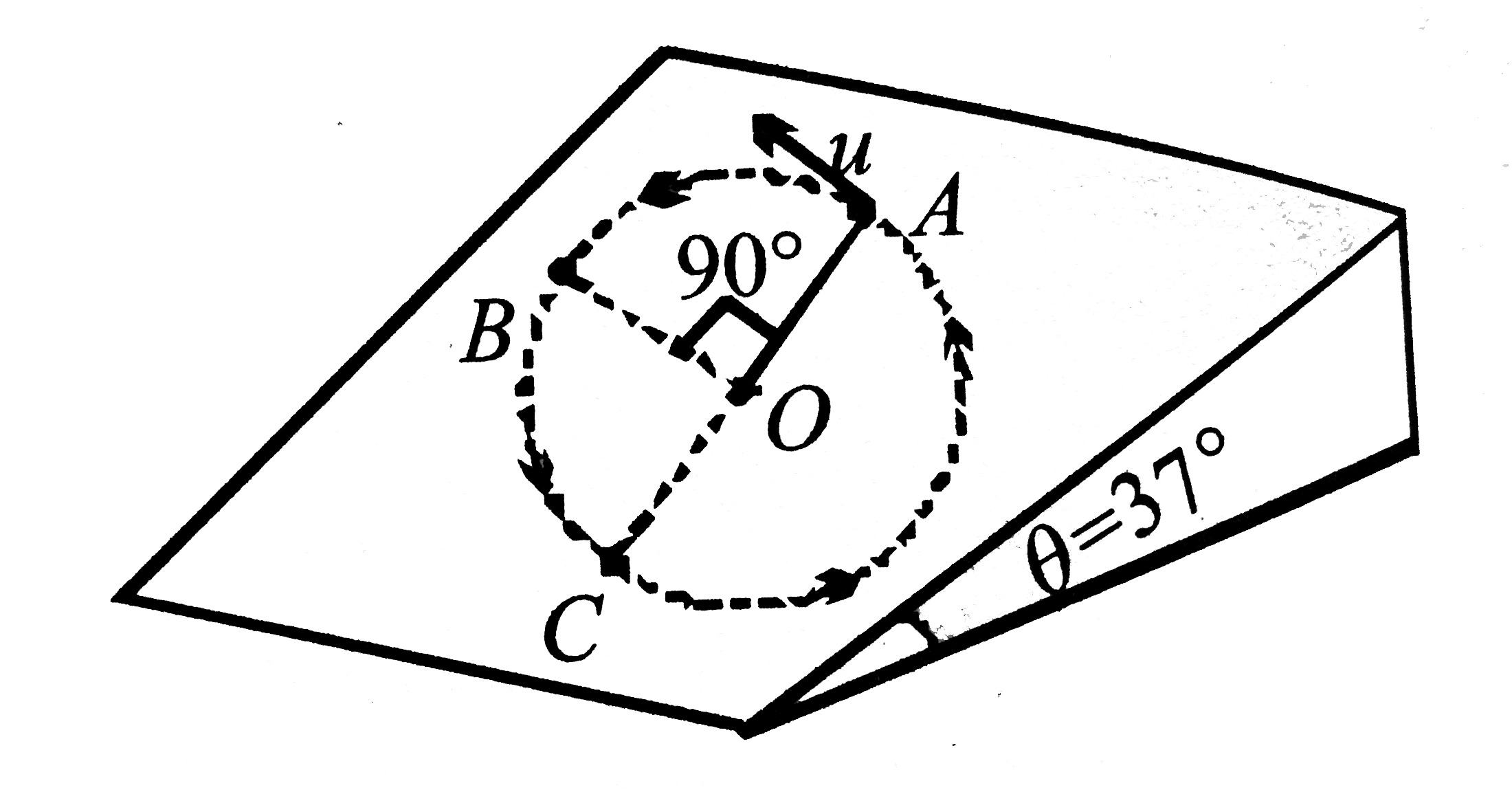
Minimum velocity at A so that the string does not get slack instanntaneously is √35gr
Tension at B is 8mg/5 if the sphere has the required velocity as in option a.
Tension C is 18mg/5 in situation of option b.
none of these
The correct Answer is:A, C, D
Minimum velocity at A should be
vA=√gsin37∘r=√35gr
Velocity at B:v2B=v2A+2grsin37∘
⇒v2B=35gr+65gr⇒vB=√9gr5
TB=mv2Br=9mg5
Velocity at C:v2C=v2A+2g2rsin37∘
⇒v2C=35gr+125gr⇒vC=√3gr
TC−mgsin37∘=mv2Cr
⇒TC−35mg=3mg⇒TC=185mg
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - LC_TYPE
34 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
Similar Questions
A particle of mass m attached to a string of length l is descending circular motion on a smooth plane inclined at an angle α with the horizontal. For the particle to reach the highest point its velocity at the lowest point should exceed.
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View Solution
Knowledge Check
A solid cylinder of mass M and radius R rolls without slipping down an inclined plane making an angle 6 with the horizontal. Then its acceleration is.
A1/3 g sinθB2/3 g sinθC2/5 g sinθD2/7 g sinθ
A block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA solid cylinder of mass M and radius R rolls from rest down a plane inclined at an angle θ to the horizontal. The velocity of the centre of mass of the cylinder after it has rolled down a distance d is :
View SolutionA round uniform body of radius R, mass M and moment of inertia 'I' rolls down (without slipping) and inclined plane making an angle θ with the horizontal. Then its acceleration is.
View SolutionA solid sphere of mass m and radius R is released from top of an incline having co-efficent of friction μ and making an angle of 45∘ with the horizontal. Choose the correct alternative (s)
View SolutionA drum of radius R and mass M, rolls down without slipping along an inclined plane of angle θ. The frictional force-
View SolutionA small block of mass m has speed√gR2 at the top most point of a fixed hemisphere of radius R as shown in figure. The angle θ, when block loses the contact with the hemisphere is
_PHY_E05_149_Q01.png)
View SolutionA sphere of mass 300 grams is rotated at uniform angular velocity 2rads−1 along a circle of radius 1.25 m in horizontal plane with help of light horizontal string. The tension in the string is
View Solution
