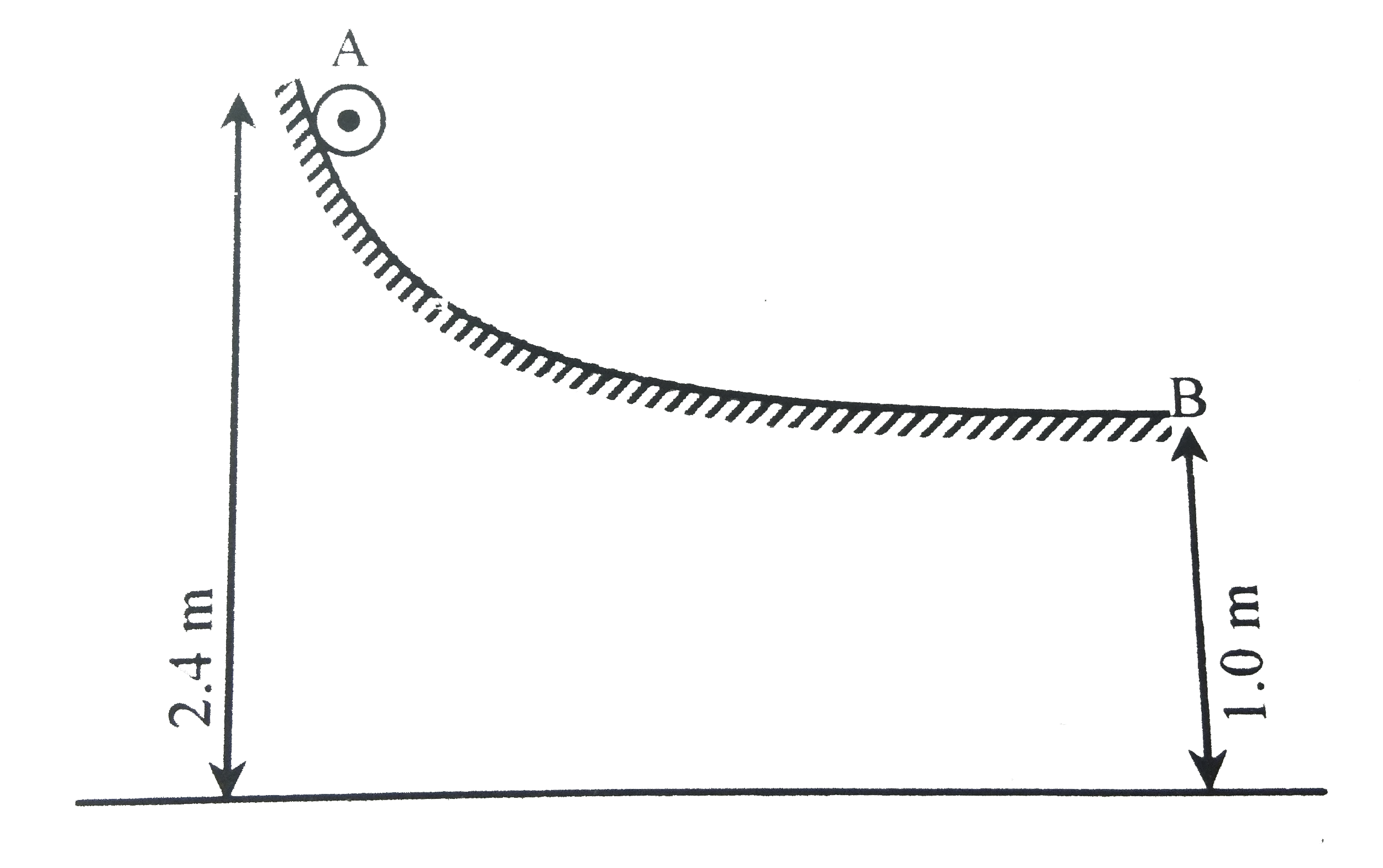
A small sphere rolls down without slipping from the top of a track in a vertical plane. The track has an elevated section and a horizontal part, The horizontal part, is 1.0 metre above the ground lenvel and the top of the track is 2.4 meters above the ground. Find the distance on the ground with respect to the point B (which is vertically below the end of the track as shown i fig.) where the sphere lands. During its flight as a projectlie, does the sphere continue to rotate about its centre of mass? Explain.

Video Solution

Text Solution

Verified by Experts

Applying the energy conservation between $O$ and $A$
$m g (2.4 - 1.0) = \frac{1}{2} m v^{2} (1 + \frac{k^{2}}{R^{2}}) = \frac{1}{2} m v^{2} (1 + \frac{2}{5})$
$m g (1.4) = \frac{7}{10} m v^{2} \Rightarrow v^{2} = 20$
$A$ to $C$ (projective motion)
$y = \frac{g x^{2}}{2 y^{2}} \Rightarrow 1 = \frac{10 x^{2}}{2 \times 20} \Rightarrow x = 2 m$
During the flight, no external torque as weight is passing through the center of mass, hence the angular velocity remains same.
Recall: When a ball is thrown from the top of a tower in a horizontal direction

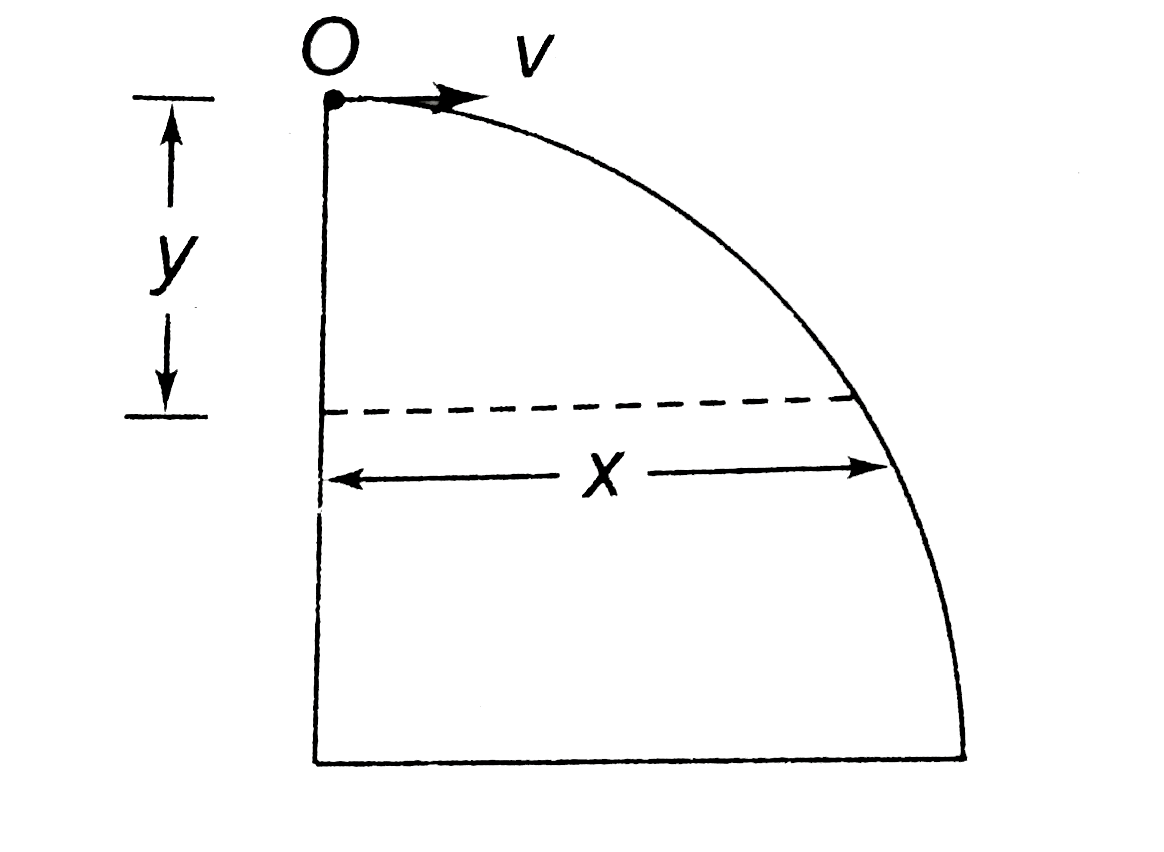
$x = v t, y = \frac{1}{2} {gt}^{2}$
Eliminating $t$ , we get
$y = \frac{g x^{2}}{2 v^{2}}$

Updated on:21/07/2023