A smooth air-tight piston connected to a spring of force constant k and unstretched length l separates two regions of a tube as shown in figure. Region A is evacuted and region B is open to the atmosphere. How will you use this set up to determine the atmospheric pressure ?

Step by step video & image solution for A smooth air-tight piston connected to a spring of force constant k and unstretched length l separates two regions of a tube as shown in figure. Region A is evacuted and region B is open to the atmosphere. How will you use this set up to determine the atmospheric pressure ? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - MCQ
17 Videos
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - Numberical MCQ single
36 Videos
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - Practice exercise 7.3
9 Videos
- GRAVITATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical
94 Videos
Similar Questions
A L Shaped tube containing liquid of density pis accelerated horizontally with acceleration as shown in the figure. The end of tube B is closed while end A is open. If the atmospheric pressure is Po. Then the pressure at B is :
View SolutionPlate A of parallel plate air filled capacitor is connected to a spring having force constant k and plate B is fixed. They are held on a frictionless table top as shown in Fig. If charge +q is placed on plate A and a charge −q on plate A and a charge −q on plate B, by how much does the spring expand ?
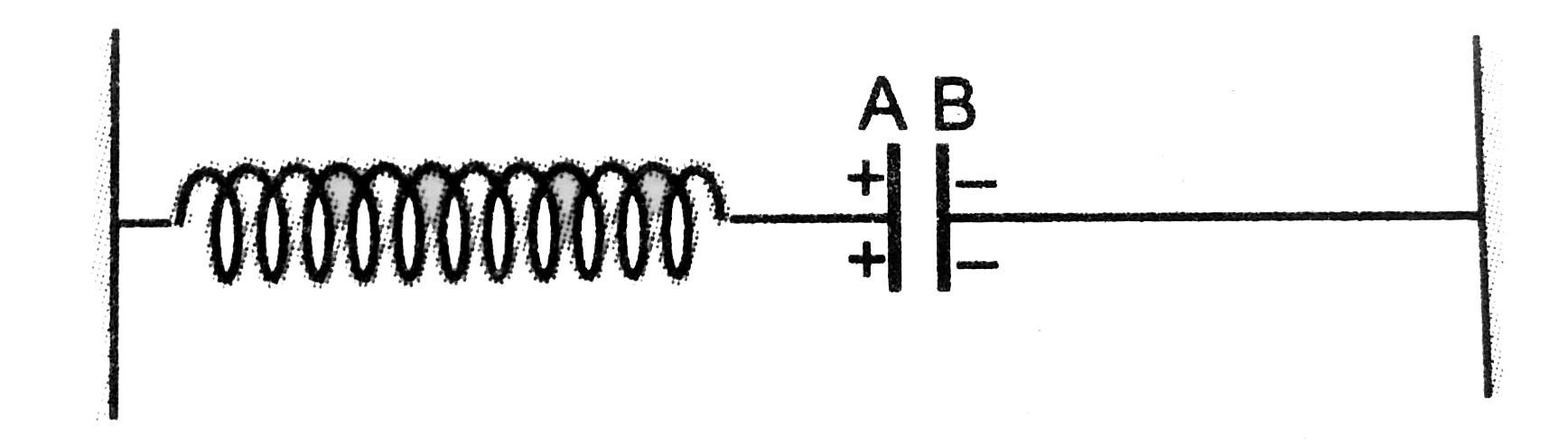
View Solution
Knowledge Check
Two blocks of mass m and 2m are kept on a smooth horizontal surface. Theya are connected by an ideal spring of force constant k. Initially the spring is unstretched. A constant force is applied to the heavier block in the direction shown in figure. Suppose at time t displacement of smaller block is x1 then displacement of the heavier block at this moment would be
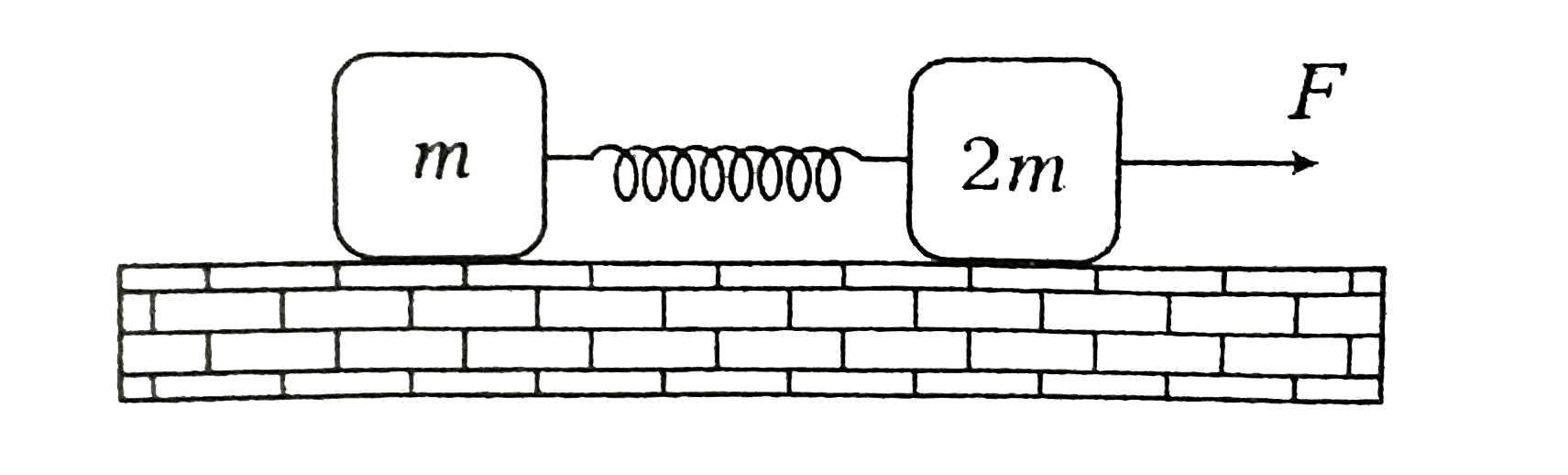 Ax2BFt26m+x3Cx3DFt24m−x2
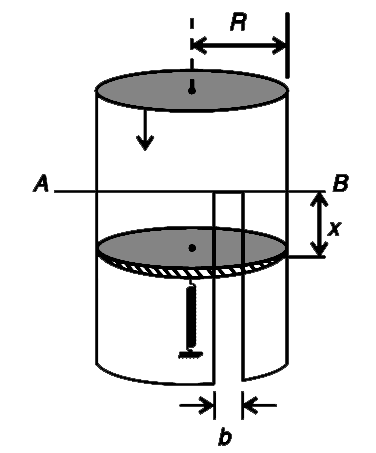
Ax2BFt26m+x3Cx3DFt24m−x2A light cylindrical tube 'T' of length l and radius 'r' containing air is inverted in water (density d). One end of the tube is open and the other is closed A block 'B' of density 2d is kept on the tube as shown in the figure. The tube stays in equilibrium in the position shown. (Assume the atmosphere pressure is to be P0)
Assume that density of air is very small than density of block and water. Pick up the correct statement (s)
 Athe volume of block B is πr2l3Bthe volume of block B is 2πr2l3Cthe pressure of air trapped in the tube is P1+dg(h+l3)Dthe pressure of air trapped in the tube is P0+dg(h+2l3)
Athe volume of block B is πr2l3Bthe volume of block B is 2πr2l3Cthe pressure of air trapped in the tube is P1+dg(h+l3)Dthe pressure of air trapped in the tube is P0+dg(h+2l3)
The device shown in fiugre can be used to measure the pressure and volume flow rate when a person exhales. There is a slit of width b running down th length of the cylinder. Inside the tube there is a light movable piston attached to an ideal spring of force constant K. In equiilibrium position the piston is at a position where the slit starts (shown by line AB in the figure. A person is made to exhale into the cylinder causing the piston to compress the spring. Assume that slit width b is very small and the outflow area is much smaller tan the cros section of the tube, even at the pistons full extension. A person exhales and the spring compresses by x. (Density of air=ρ)
(a) Calculate the gage pressure in the tube.
(b) Calculate the volume flow rate (Q) of the air.
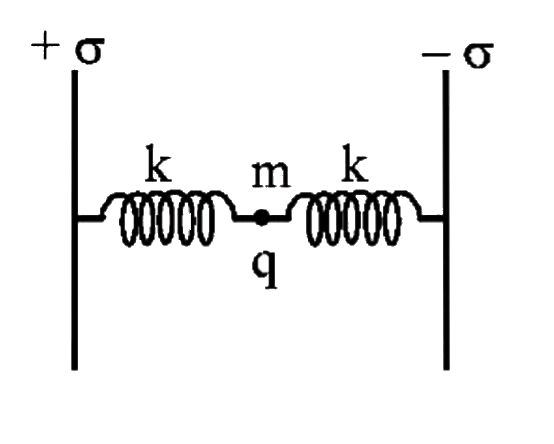
View SolutionTwo large insulating plates having surface charge densities +σ and −σ are fixed some distance apart in a gravity -free region and two ideal insulating springs of force constant k are connected to the plates as shown in the figure. A particle of charge q and mass m which is attached to the junction of the spring is released from rest, then the partice will cross its equilibrium position with a speed
View SolutionTwo blocks A and B of masses m and 2m, respectively , are connected by a massless spring of force constant k and are placed on a smooth horizontal plane. The spring is stretched by an amount x and then released . The relative velocity of the blocks when the spring comes to its natural length is
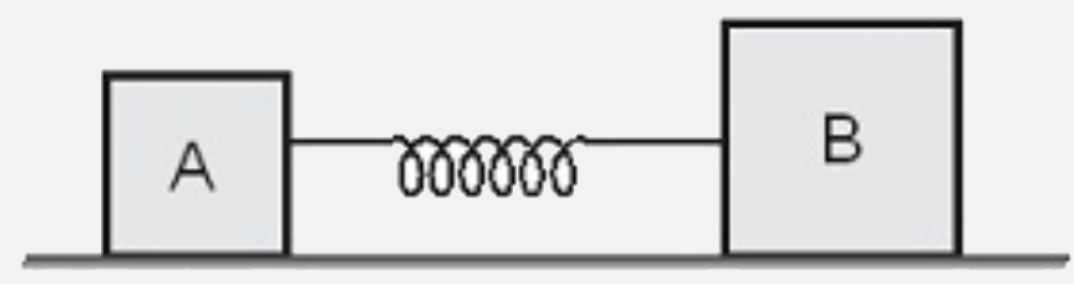
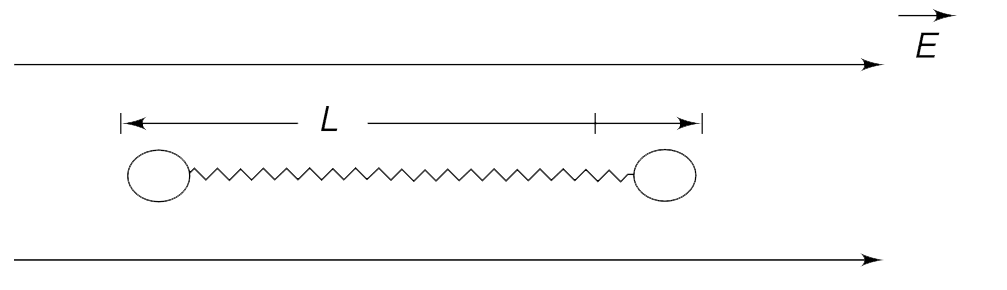
View SolutionTwo small identical conducting balls each of radius r and mass m are placed on a frictionless horizontal table, connected by a light conducting spring of force constant K and un-deformed length L (L gtgt r). A uniform electric field of strength E is switched on in horizontal direction parallel to the spring. (a) How much charge will appear on the two balls when they are at separation L. (b) The system fails to oscillate if K lt K0. Find K0. (c) Assuming K=2K0, find the time period of oscillation after the electric field is switched on.
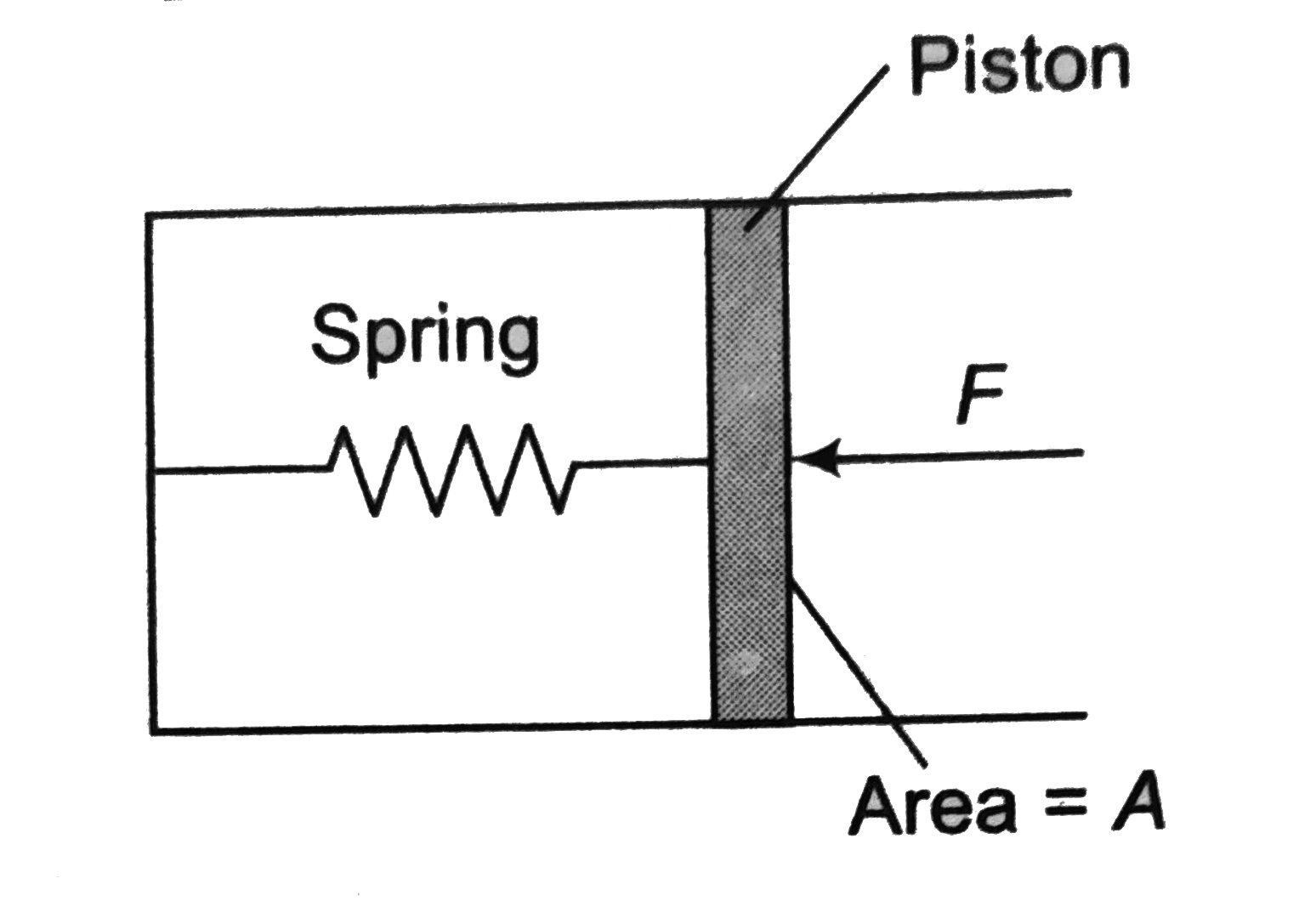
View SolutionThe pressure gauge shown in figure has a spring for which k=60N/m and the area of the piston is 0.50cm2. Its right end is connected to a closed container of gas at a gauge pressure of 30kPa. How far will the spring be compressed if the region containing the spring is (a) in vacuum and (b) open to the atmosphere? Atmospheric pressure is 101kPa.
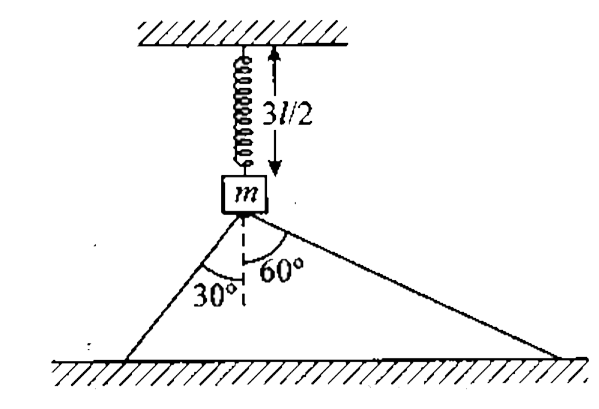
View SolutionFigure-2.107 shows a block of mass m attached to a spring of force constant k and connected to ground by two string of equal lengths making an angle 90∘ with each other. In relaxed state natural length of the spring is l. In the situation shown in figure, find the tensions in the two strings.
View Solution
