A smooth wedge of mass M rests on a smooth horizontal surface. A block of mass m is projected from its lowermost point with velocity v0. What is the maximum height reached by the block?
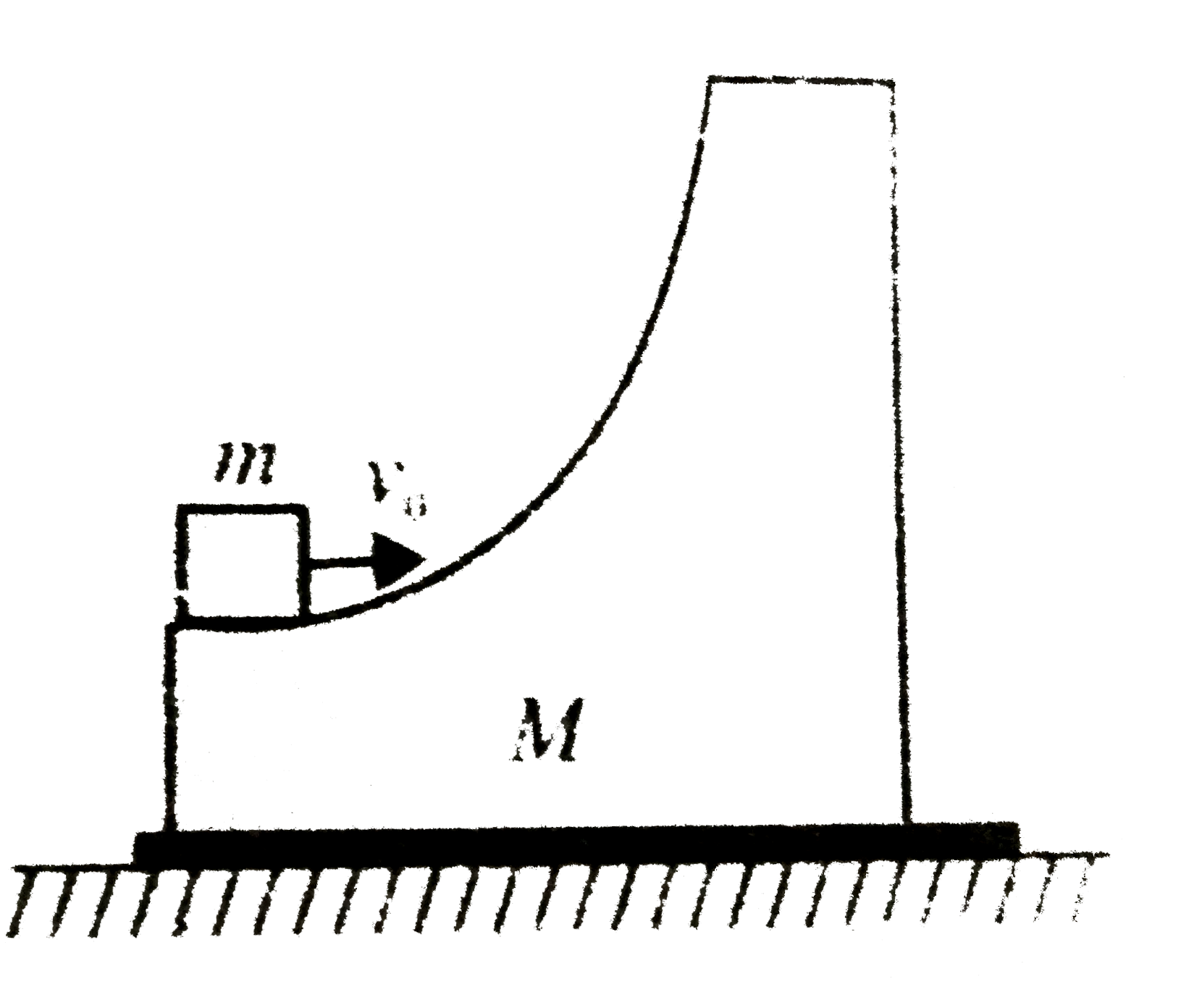
Method 1 (Ground frame method)
At the maximum height, both m1 and m2 have same velocity as the relative sliding between them stops. Since no external force acts horizontally, linear momentum is conserved in horizontal direction
(→px)f=(→px)i
where →pxi=m1v0ˆi and →pxf=(m1+m2)vˆi
Then we have (m1+m2)v=m1v0
WE theorem Wext+Wint=△K
where Wext=−m1gh,Wint=Wcontact=0,
and △K=[12(m1+m2)v2−m1v202]
This gives −m1gh=12(m1+m2)v2−m1v202........ii
Sustituting v from eqn i and eqn ii we have
h=v202(1+m1m2)g
Method 2: (Work energy theorem from centre of mass frame)
The block attains a maximum height when the final relative velociyt vrel between block and wedge becomes zero.
that mean (vref)=0.
The initial relative velocity betwen block and wedge is given as
(vref)i=v0
Substituting (vref_(f)=0 and (vrel)i=v0 in the formula.
△K'=12μ[(vrel_(f)2−(vrel)2i]
we have △K'=−12μv20
while ascending through a height h (say) the external force, that is the earth's gravity does work on the block m1 which can be given as:
Wext(=Wgravity)=−m1gh
The internal (constraint) forces (normal reactions) between m1 and m2 as a whole do not performe work, W∫=0
Finally, putting △K'=−12μv20,Wext=−m1gh and Wint=0 in
We theorem Wext+Wint=△K'
we have −m1gh+0=−12μv20
whereμ=m1m1m1+m2
This gives h=v202(1+m1m2)g
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples
13 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
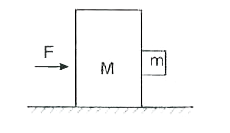
A horizontal force F pushes wedge of mass M on a smooth horizontal surface. A block of mass m is stationary to the wedge, then:
View Solution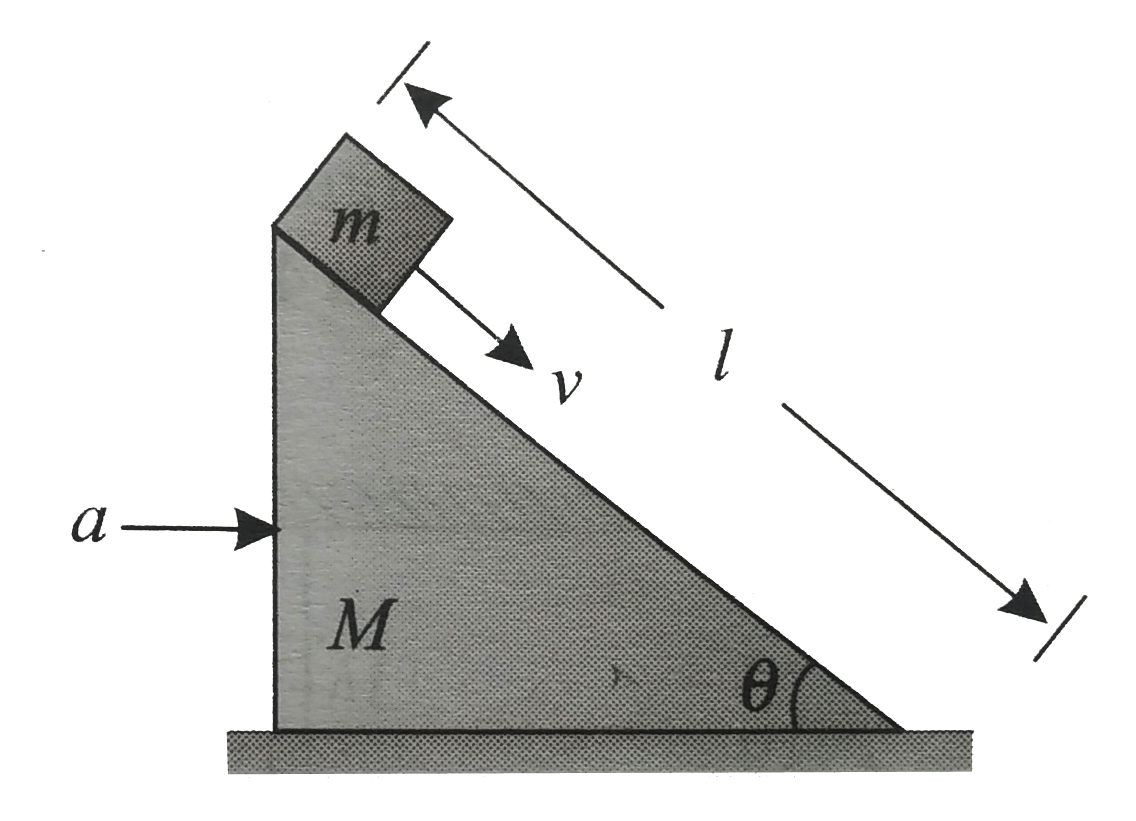
A smooth wedge of mass M is pushed with an acceleration a=>anθ and a block of mass m is projected down the slant with a velocity v relative to the wedge.
Q. The time taken by the block to reach the ground is:
View Solution_2019_CBT4_E01_069_Q01.png)
A wedge of mass 4m is initially at rest on frictionles horizotnal surface. A small block of mass m moving with speed v0 and climbs on wedge. Find maximum height achieved by block
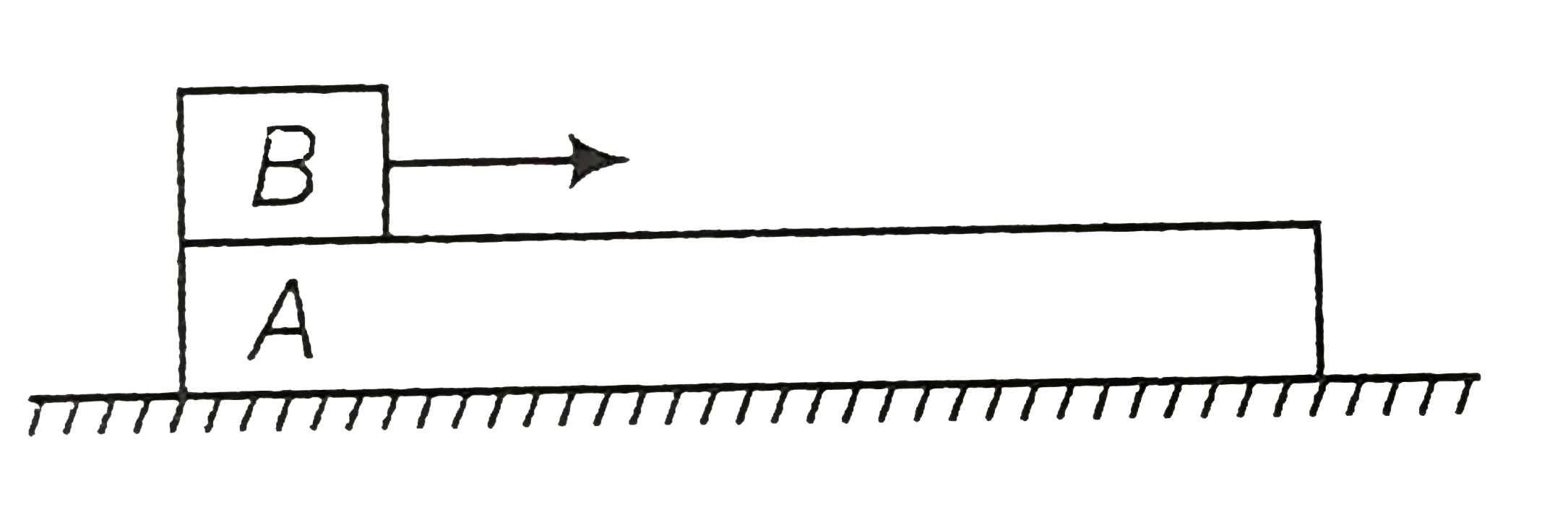
View SolutionA long block A of mass M is at rest on a smooth horizontal surface.A small block B of mass M/2 is placed on A at one end and projected along A with some veklocity v.The coefficient of friction between the block is μ then , the accelerations of blocks A and B before reaching a common velocity will be respectively
View SolutionFigure shows a block A of mass 6m having a smooth semicircular groove of radius a placed on a smooth horizontal surface. A block B of mass m is released from a position in groove where its radius is horizontal. Find the speed of the bigger block when the smaller block reaches its bottom most position.
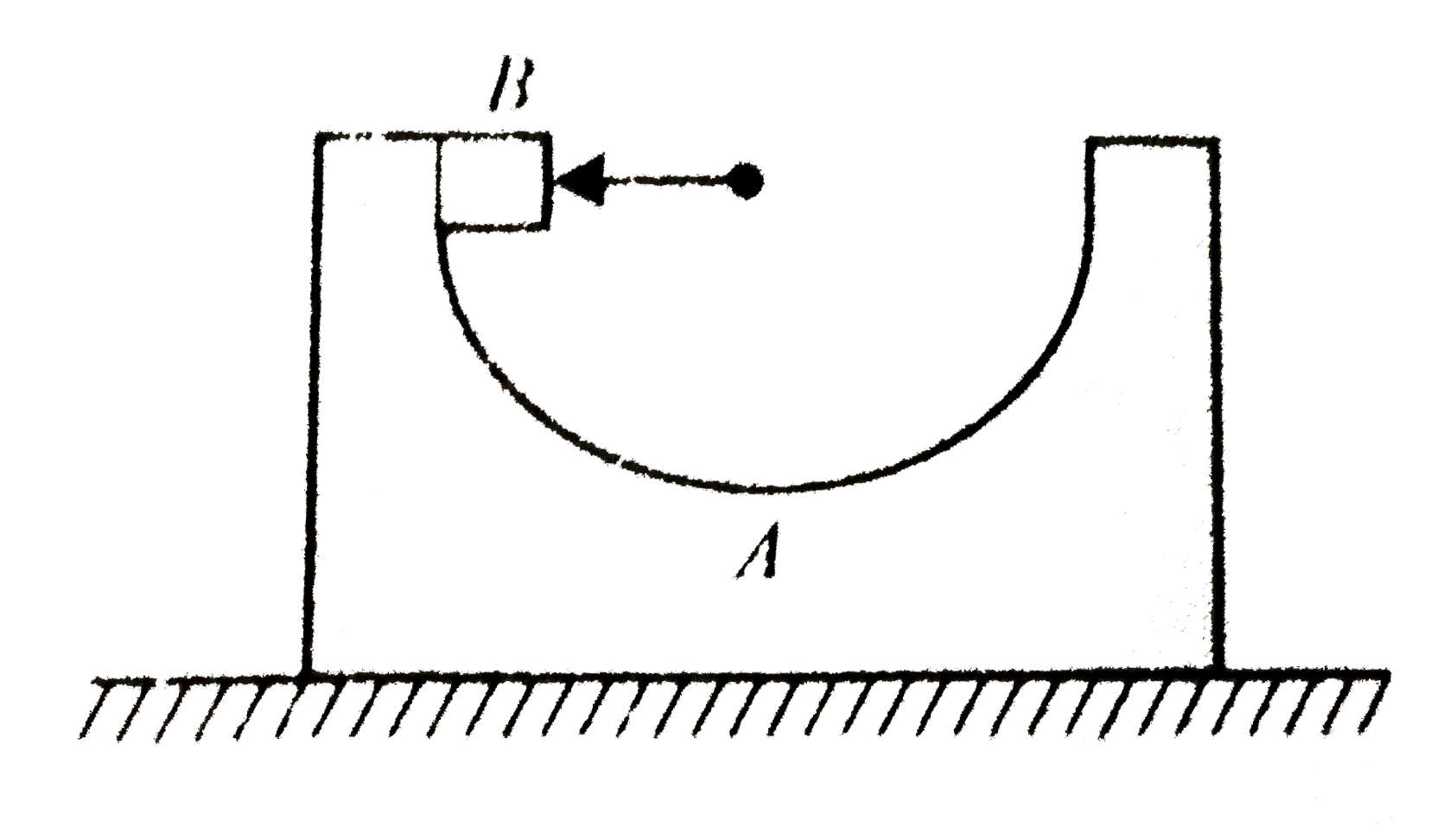
View Solution
A smooth wedge of mass M is pushed with an acceleration a=>anθ and a block of mass m is projected down the slant with a velocity v relative to the wedge.
The horizontal force applied on the wedge is:
View Solution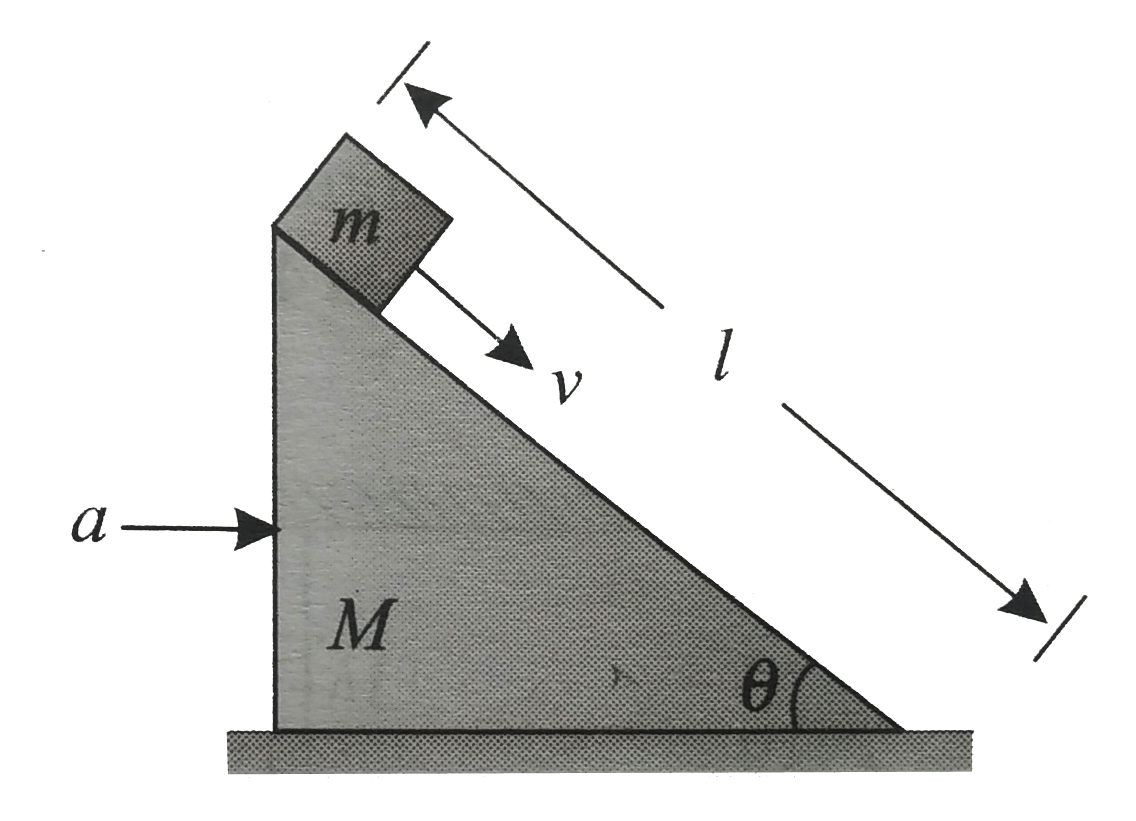
A smooth wedge of mass M is pushed with an acceleration a=gtanθ and a block of mass m is projected down the slant with a velocity v relative to the wedge.
Q. The normal reaction between wedge and block is:
View Solution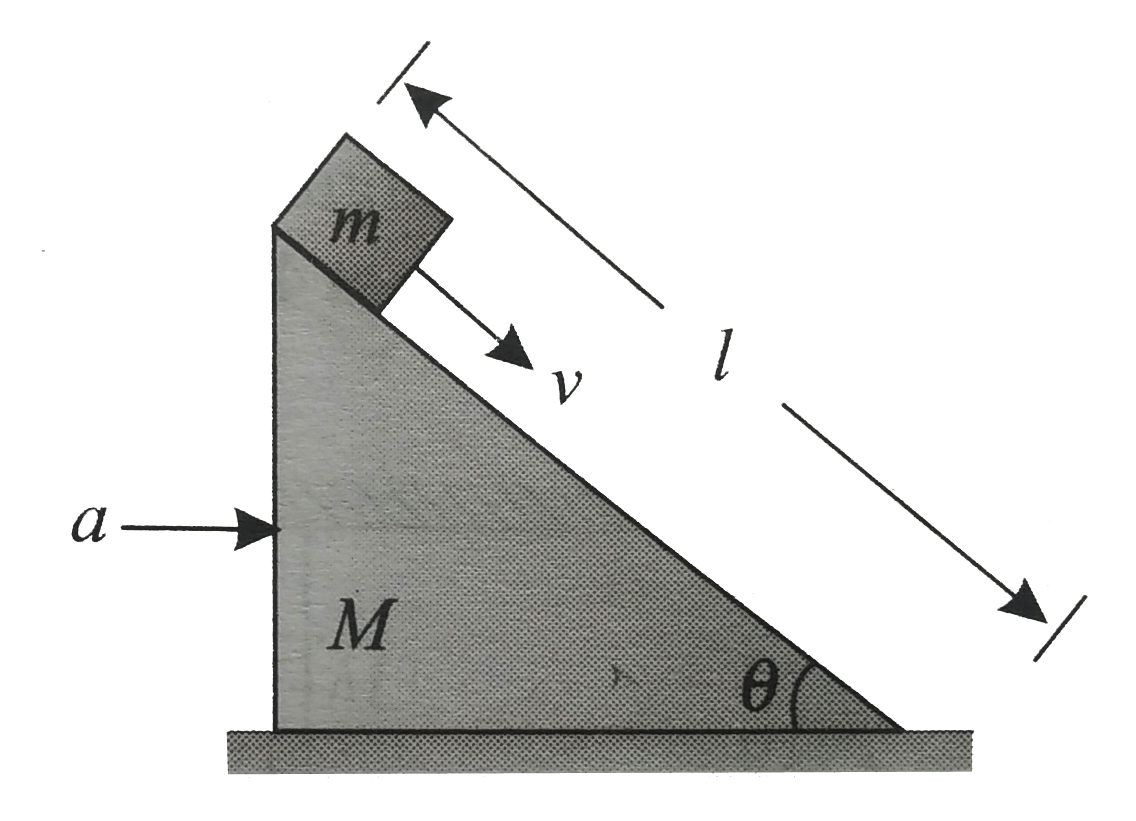
A smooth wedge of mass M is pushed with an acceleration a=>anθ and a block of mass m is projected down the slant with a velocity v relative to the wedge.
Q. The normal reaction offered by ground to the wedge is:
View SolutionA wedge shaped block A' of mass M is at rest on a smooth horizontal surface. A small block 'B' of mass 'm' placed at the top edge of inclined plane of length 'l' as shown in the figure. By the time, the block 'B' reaches the bottom end, the wedge A moves a distance of:

View SolutionA wedge of mass M=2m rests on a smooth horizontal plane. A small block of mass m rests over it at left end A as shown in figure. A sharp impulse is applied on the block, due to which it starts moving to the right with velocity v0=6ms−1. At highest point of its trajectory, the block collides with a particle of same mass m moving vertically downwards with velocity v=2ms−1 and gets stuck with it. If the combined body lands at the end point A of body of mass M, calculate length l. Neglect friction (g=10ms−2).

View Solution
