A soap bubble of radius r is placed on another bubble of radius 2r. The radius of the surface common to both the bubbles is
2r3
3r
2r
r
The correct Answer is:C
To find the radius of the surface common to both bubbles, we can follow these steps:
1. Identify the Radii of the Bubbles:
- Let the radius of the smaller bubble be r.
- Let the radius of the larger bubble be 2r.
2. Define the Radius of the Common Surface:
- Let the radius of the common surface be R.
3. Understand the Pressure Inside the Bubbles:
- The pressure inside a soap bubble is given by the formula:
P=4Tr
- Where T is the surface tension of the soap solution.
4. Calculate the Pressure Inside Each Bubble:
- For the smaller bubble (radius r):
P1=4Tr
- For the larger bubble (radius 2r):
P2=4T2r=2Tr
5. Set Up the Pressure Balance at the Common Surface:
- At the common surface, the pressure inside the smaller bubble P1 must equal the pressure inside the larger bubble P2:
P1=P2
- Therefore:
4Tr−4TR=2Tr−4TR
6. Rearranging the Equation:
- Rearranging gives:
4TR=4Tr−2Tr
- Simplifying this leads to:
4TR=2Tr
7. Solving for R:
- Cross-multiplying gives:
4Tr=2TR
- Dividing both sides by 2T (assuming T≠0):
2r=R
8. Conclusion:
- Thus, the radius of the surface common to both bubbles is:
R=2r
Final Answer:
The radius of the surface common to both bubbles is 2r.
- Let the radius of the smaller bubble be r.
- Let the radius of the larger bubble be 2r.
- Let the radius of the common surface be R.
- The pressure inside a soap bubble is given by the formula:
P=4Tr
- Where T is the surface tension of the soap solution.
- For the smaller bubble (radius r):
P1=4Tr
- For the larger bubble (radius 2r):
P2=4T2r=2Tr
- At the common surface, the pressure inside the smaller bubble P1 must equal the pressure inside the larger bubble P2:
P1=P2
- Therefore:
4Tr−4TR=2Tr−4TR
- Rearranging gives:
4TR=4Tr−2Tr
- Simplifying this leads to:
4TR=2Tr
- Cross-multiplying gives:
4Tr=2TR
- Dividing both sides by 2T (assuming T≠0):
2r=R
- Thus, the radius of the surface common to both bubbles is:
R=2r
The radius of the surface common to both bubbles is 2r.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced
57 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A soap bubble of raidus R is surrounded by another soap bubble of radius 2R, as shown. Take surface tension =S. Then the pressure inside the smaller soap bubble, in excess of the atmosphere presure will be
View SolutionA soap bubble A of radius 0.03 m and another bubble B of radius 0.04 m are brought together, so that the combined bubble has a common interface of radius r, then the value of r is
View Solution
Knowledge Check
A spherical soap bubble of radius 1 cm is formed inside another of radius 3 cm the radius of single soap bubble which maintains the same pressure difference as inside the smaller and outside the larger soap bubble is ____cm
A0.75cmB0.75mC7.5cmD7.5m
A soap bubble of radius 'r' is blown up to form a bubble of radius 2r under isothemal conditions. If σ be the surface tension of soap solution , the energy spent in doing so is
View SolutionA small soap bubble of radius 4 cm is trapped inside another bubble of radius 6 cm without any contact. Let P2 be the pressure inside the inner bubble and P0, the pressure outside the outer bubble. Radius of another bubble with pressure difference P2−P0 between its inside and outside would be :
View SolutionA soap - bubble with a radius 'r' is placed on another bubbles with a radius R (figure). Angles between the film at the point of contact will be -

View SolutionTwo separate air bubbles (radii 0.002cm and 0.004) formed of the same liquid (surface tension 0.07N/m) come together to form a double bubble. Find the radius and the sense of curvature of the internal film surface common to both the bubbles.
View SolutionA soap bubble in vacuum has a radius of 3 cm ad another soap bubble in vacuum has a radius of 4 cm. if the two bubbles coalesce under isothermal condition, then the radius of the new bubble is
View SolutionAir is pushed into a soap bubble of radius r to double its radius. If the surface tension of the soap solution is S, the work done in the process is
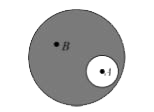
View SolutionThere is an air bubble of radius R inside a drop of water of radius 3R. Find the ratio of gauge pressure at point B to that at point A.
View Solution
