A steel ring of radius r and cross section area A is fitted on to a wooden disc of radius R(R>r). If Young's modulus be E, then the force with which the steel ring is expanded is
AERr
AE(R−rr)
EA(R−rA)
ErAR
The correct Answer is:B
To find the force with which the steel ring is expanded when fitted onto a wooden disc, we can follow these steps:
1. Identify the Parameters:
- Let the radius of the steel ring be r.
- Let the radius of the wooden disc be R.
- Let the cross-sectional area of the steel ring be A.
- Let Young's modulus of the steel be E.
2. Determine the Change in Circumference:
- The initial circumference of the steel ring is given by:
Cinitial=2πr
- The circumference of the wooden disc is:
Cfinal=2πR
- The change in length (circumference) when the ring is fitted onto the disc is:
ΔC=Cfinal−Cinitial=2πR−2πr=2π(R−r)
3. Calculate the Strain:
- Strain is defined as the change in length divided by the original length. Here, the original length is the initial circumference of the ring:
Strain=ΔCCinitial=2π(R−r)2πr=R−rr
4. Apply Young's Modulus:
- Young's modulus E is defined as the ratio of stress to strain:
E=StressStrain
- Stress is defined as force per unit area:
Stress=FA
- Therefore, we can write:
E=F/A(R−r)/r
5. Rearranging to Find Force:
- Rearranging the equation to solve for force F:
F=E⋅A⋅(R−r)r
6. Final Expression:
- Thus, the force with which the steel ring is expanded is:
F=E⋅A⋅(R−r)r
- Let the radius of the steel ring be r.
- Let the radius of the wooden disc be R.
- Let the cross-sectional area of the steel ring be A.
- Let Young's modulus of the steel be E.
- The initial circumference of the steel ring is given by:
Cinitial=2πr
- The circumference of the wooden disc is:
Cfinal=2πR
- The change in length (circumference) when the ring is fitted onto the disc is:
ΔC=Cfinal−Cinitial=2πR−2πr=2π(R−r)
- Strain is defined as the change in length divided by the original length. Here, the original length is the initial circumference of the ring:
Strain=ΔCCinitial=2π(R−r)2πr=R−rr
- Young's modulus E is defined as the ratio of stress to strain:
E=StressStrain
- Stress is defined as force per unit area:
Stress=FA
- Therefore, we can write:
E=F/A(R−r)/r
- Rearranging the equation to solve for force F:
F=E⋅A⋅(R−r)r
- Thus, the force with which the steel ring is expanded is:
F=E⋅A⋅(R−r)r
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Bulk Modulus And Shear Modulus29videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Elasticity And Work Done In Stretching A Wire25videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test29videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Chapter Test29videos
ROTATIONAL DYNAMICS
Similar Questions
A metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
View SolutionA steel ring is to be fitted on a wooden disc of radius R and thickness d. The inner radius of the ring is r which is slightly smaller than R. The outer radius of the ring is r + b and its thickness is d (same as the disc). There is no change in value of b and d after the ring is fitted over the disc, only the inner radius becomes R. If the Young’s modulus of steel is Y, calculate the longitudinal stress developed in it. Also calculate the tension force developed in the ring.
[Take b<<r]
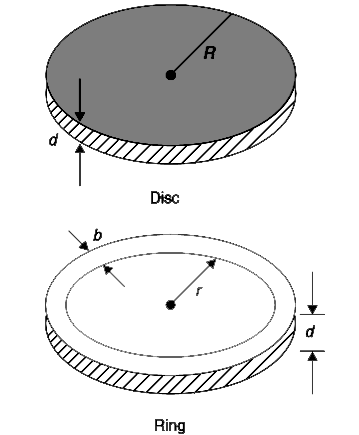 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A steel ring of radius r and cross section area A is fitted on to a wooden disc of radius R(R>r). If Young's modulus be E, then the force with which the steel ring is expanded is
AAERrBAE(R−rr)CEA(R−rA)DErAR - Question 2 - Select One
A metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
AAYRrBAY(R−r)rCYA(R−rr)DYrAR - Question 3 - Select One
A metallic rod of radius 2cm and cross sectional area 4cm2 is fitted into a wooden circular disc of radisu 4cm. If the Young's modulus of the materail of the ring is 2×1011N/m2, the force with which the metal ring expands is:
A2×107NB8×107NC4×107ND6×107N
A uniform ring of radius R and made up of a wire of cross - sectional radius r is rotated about its axis with a frequency f. If density of the wire is ρ and young's modulus is Y . Find the fractional change in radius of the ring .
View SolutionA steel wire is stretched by 5kwt. If the radius of the wire is doubled its Young's modulus
View SolutionA steel wire of length 1m, and radius 0.1mm is elongated by 1mm due to a weight of 3.14kg. If g=10m/s2, the Young's modulus of the steel wire will be
View SolutionA ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The tension in the ring will beView SolutionA ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The ratio of kinetic energy to potential energy isView Solution

