A steel rod of cross sectional area 4cm2 and length 2m shrinks by 0.1cm as the temperature decreases in night. If the rod is clamped at both ends during the day hours, find the tension developed in it during night hours. Young modulus of steel =1.9×1011Nm−2
The correct Answer is:A, C, D
To solve the problem step by step, we will follow these steps:
Step 1: Convert the given values into SI units
- Cross-sectional area A=4cm2=4×10−4m2
- Length of the rod L=2m
- Contraction ΔL=0.1cm=0.1×10−2m=0.001m
- Young's modulus Y=1.9×1011N/m2
Step 2: Calculate the strain in the rod
Strain ϵ is defined as the change in length divided by the original length:
ϵ=ΔLL=0.001m2m=0.0005
Step 3: Relate stress and strain using Young's modulus
Young's modulus Y is defined as the ratio of stress σ to strain ϵ:
Y=σϵ
Where stress σ is given by:
σ=TA
Thus, we can write:
Y=T/Aϵ
Step 4: Rearranging the equation to find tension T
From the equation above, we can express tension T as:
T=Y⋅ϵ⋅A
Step 5: Substitute the known values into the equation
Now we substitute the values we have:
T=(1.9×1011N/m2)⋅(0.0005)⋅(4×10−4m2)
Step 6: Calculate the tension
Calculating the above expression:
T=1.9×1011⋅0.0005⋅4×10−4
T=1.9×1011⋅2×10−7
T=3.8×104N
Final Answer
The tension developed in the rod during the night hours is T=3.8×104N.
---
- Cross-sectional area A=4cm2=4×10−4m2
- Length of the rod L=2m
- Contraction ΔL=0.1cm=0.1×10−2m=0.001m
- Young's modulus Y=1.9×1011N/m2
Strain ϵ is defined as the change in length divided by the original length:
ϵ=ΔLL=0.001m2m=0.0005
Young's modulus Y is defined as the ratio of stress σ to strain ϵ:
Y=σϵ
Where stress σ is given by:
σ=TA
Thus, we can write:
Y=T/Aϵ
From the equation above, we can express tension T as:
T=Y⋅ϵ⋅A
Now we substitute the values we have:
T=(1.9×1011N/m2)⋅(0.0005)⋅(4×10−4m2)
Calculating the above expression:
T=1.9×1011⋅0.0005⋅4×10−4
T=1.9×1011⋅2×10−7
T=3.8×104N
The tension developed in the rod during the night hours is T=3.8×104N.
Topper's Solved these Questions
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Question For short Anser
18 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-1
29 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Worked out Example
17 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
- SOUND WAVESBOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions
133 Videos
Similar Questions
A steel wire of length 4 m and diameter 5 mm is strethed by 5 kg-wt. Find the increase in its length, if the Young's modulus of steel is 2×1012 dyne cm2
View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×1011Nm−2
View Solution
Knowledge Check
A steel bar ABCD 40cm long is made up of three parts AB,BC and CD, as shown in figure. The rod is subjected to a pull of 25kN. Determine the stress in the the parts and the total extension of the rod. Young's modulus for steel=2×1011Nm−2.
 A0.0637 mmB0.0647 mmC0.0657 mmD0.0667 mm
A0.0637 mmB0.0647 mmC0.0657 mmD0.0667 mm
A steel wire of length 2.0 m is stretched through 2.0 mm. The cross sectional area of the wire is 4.0 mm^2. Calculate the elastic potential energy stored in the wire in the stretched condition. Young modulus of steel =2.0x1011Nm−2
View SolutionA steel plate of face area 4cm2 and thickness 0.5cm is fixed rigidly at the lower surface. A tangential force of 10N is applied on the upper surface. Find the lateral displacement of the upper surface with respect to the lower surface. Rigidity modulus of steel =8.4×1010Nm−2.
View SolutionA uniform steel wire of cross-sectional area 0.20mm2 is held fixed by clamping its two ends. Find the extra force exerted be each clamp on the wire if the wire is cooled from 1000C→0oC. Young's modulus of steel =2.0×1011Nm−2. Coefficient of linear expansion of =1.2×10−5C−1.
View SolutionOne end of a wire 2m long and 0.2 cm^2 in cross section is fixed in a ceiling and a load of 4.8 kg is attached to the free end. Find the extension of the wire. Young modulus of steel =2.0×1011Nm−2. Take g=10ms−2.
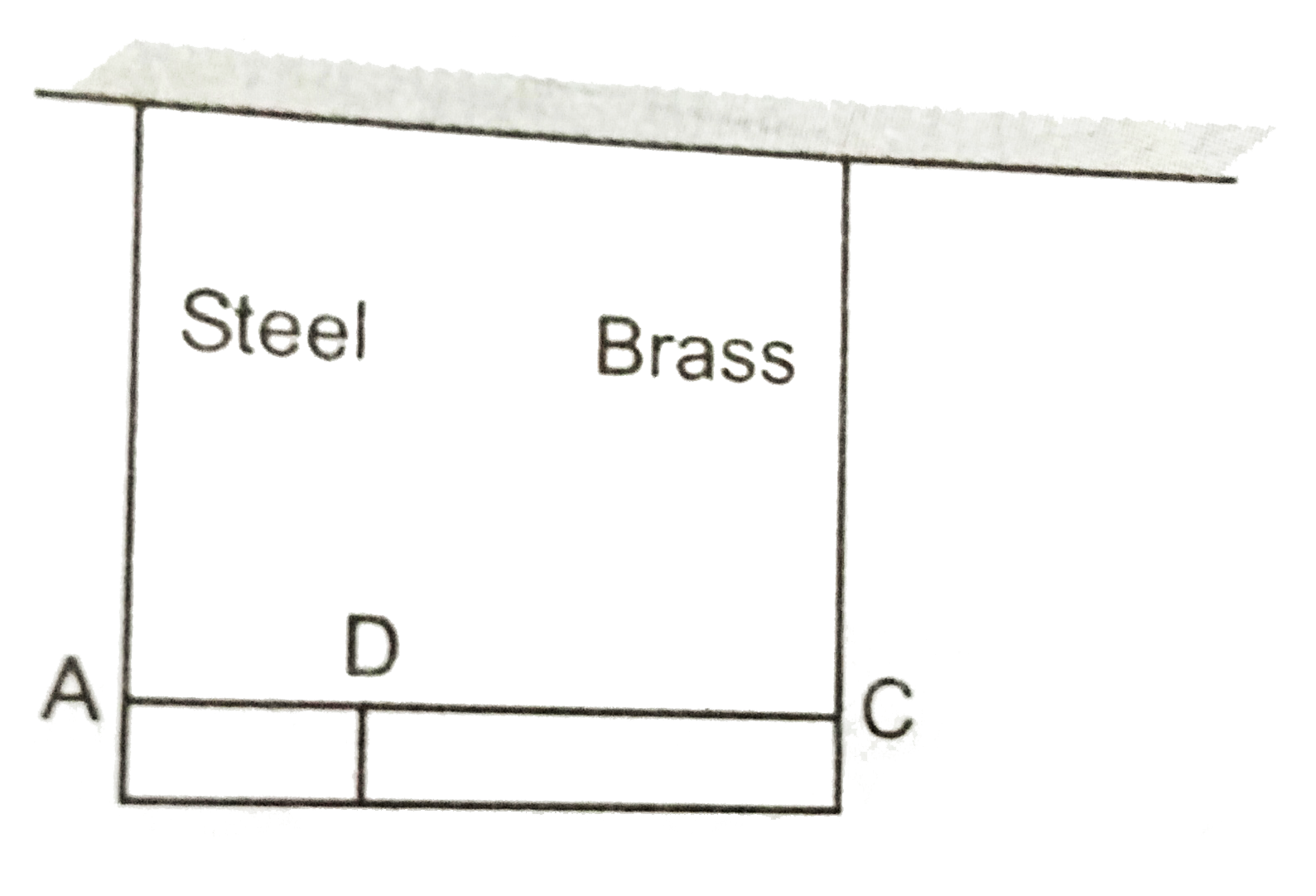
View SolutionA light rod AC of length 2.00 m is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. The position of point D from end A along the rod at which a weight may be hung to produce equal stress in both the wires is [Young's modulus of steel is 2×1011Nm2 and for brass is 1×1011Nm−2]
View SolutionA steel wire of length 600cm and diameter 1.2 mm is stretched through 4 mm by a load. Calculate the work done. Young's modulus of steel =2×1011Nm−2.
View SolutionA steel wire and a copper wire of equal length and equal cross sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of a. The stresses developed in the two wire and b. the strains developed. Y of steel =2×1011Nm−2. Y of copper =1.3×1011Nm−2.
View Solution
