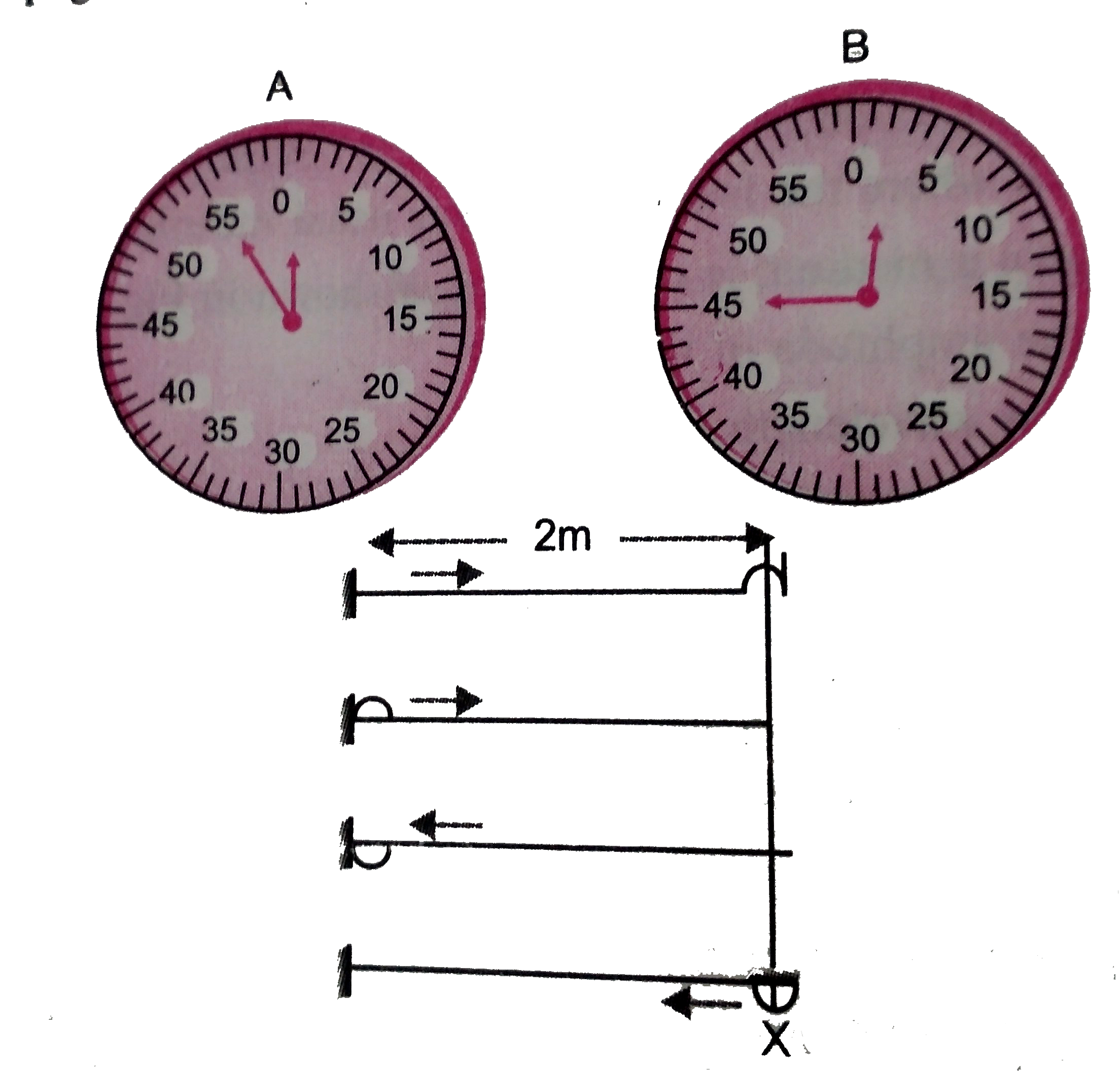
A strings is stretched and a pulse is created along it. The stopwatch is started from its position A, when the pulse is in position X and is stopped in its position B, when the pulse has travelled back to its position X.
Find the velocity of propagation of the pulse along the string.
 .
.
Initial reading of stopwatch (position A) =55s
Final reading of stopwatch (position B) =1min45s=105s
Time interval =105s−55s=50s
Distance travelled by the pulse in travelling back to position X=2m (in going from X to left side) +2m (in Velocity of propagation of the pulse =4m50s=400cm50s=8cm/s.
Topper's Solved these Questions
- SOUNDBook:PRADEEPChapter:SOUNDExercise:Model Test (sec - A)
Explore 24 Videos
- SOUNDBook:PRADEEPChapter:SOUNDExercise:Model Test (sec - B)
Explore 12 Videos
- SOUNDBook:PRADEEPChapter:SOUNDExercise:Mock Test (Sec - A)
Explore 24 Videos
- MOTIONBook:PRADEEPChapter:MOTIONExercise:Mock test
Explore 24 Videos
- WORK AND ENERGYBook:PRADEEPChapter:WORK AND ENERGYExercise:III Quiz Testing
Explore 10 Videos
Similar Questions
A string is hanging from a rigid support. A transverse
pulse is excited at its free end. The speed at which the
pulse travels a distance x is proportional to
View Solution(a) Two identical sinusoidal pulses move in opposite directions on a stretched string. Kinetic energy of each pulse is k. At the instant they overlap completely, what is kinetic energy of the resulting pulse?

(b) “A string clamped at both ends is vibrating. At the moment the string looks flat, the instantaneous transverse velocity of points along the string, excluding its end-points, must be same everywhere except at nodes.” Is this statement correct?
View Solution
Knowledge Check
A pulse on the string is inverted when it is reflected from
Aa fixed endBa free endCboth free as well as fixed endsDneither a fixed nor a free end.A string is hanging from a rigid support. A transverse pulse is excited at its free end. The speed at which the pulse travels a distance x is proportional to
AxB1xC1√xD√xA particle executing S.H.M. starts from the mean position. Its phase, when it reaches the extreme position, is
Aπ2BπC3π2D2π
A sinusoidal harmonic wave is propagating along a string stretched along x – axis. A particle on the string at x = 1 m is found to be at its mean position travelling in positive y direction at t=1s. The amplitude, wavelength and frequency of the wave are 0.01m,π2m and 20Hz respectively. Write the equation of the wave if-
(a) it is travelling along negative X direction
(b) it is travelling along positive X direction.
View SolutionA travelling wave pulse is given by y=105+(x+2t)2
Here, xandy are in meter and t in second. In which direction and with what velocity is the pulse propagation. What is the ampitude of pulse?
View SolutionA series of pulses, each of amplitude 0.150m, are sent on a string that is attached to a wall at one end. The pulses are reflected at the wall and travel back along the string without loss of amplitude. When two waves are present on the same string. The net displacement of a given point is the sum of the displacement of the individuals waves at the point. What is the net displacement at point on the spring where two pulses am crossing, (a) if the string is rigidly attached to the post? (b) if the end at which reflection occurs is free is slide up and down ?
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be its lowest position ?
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be at its lowest position?
View Solution