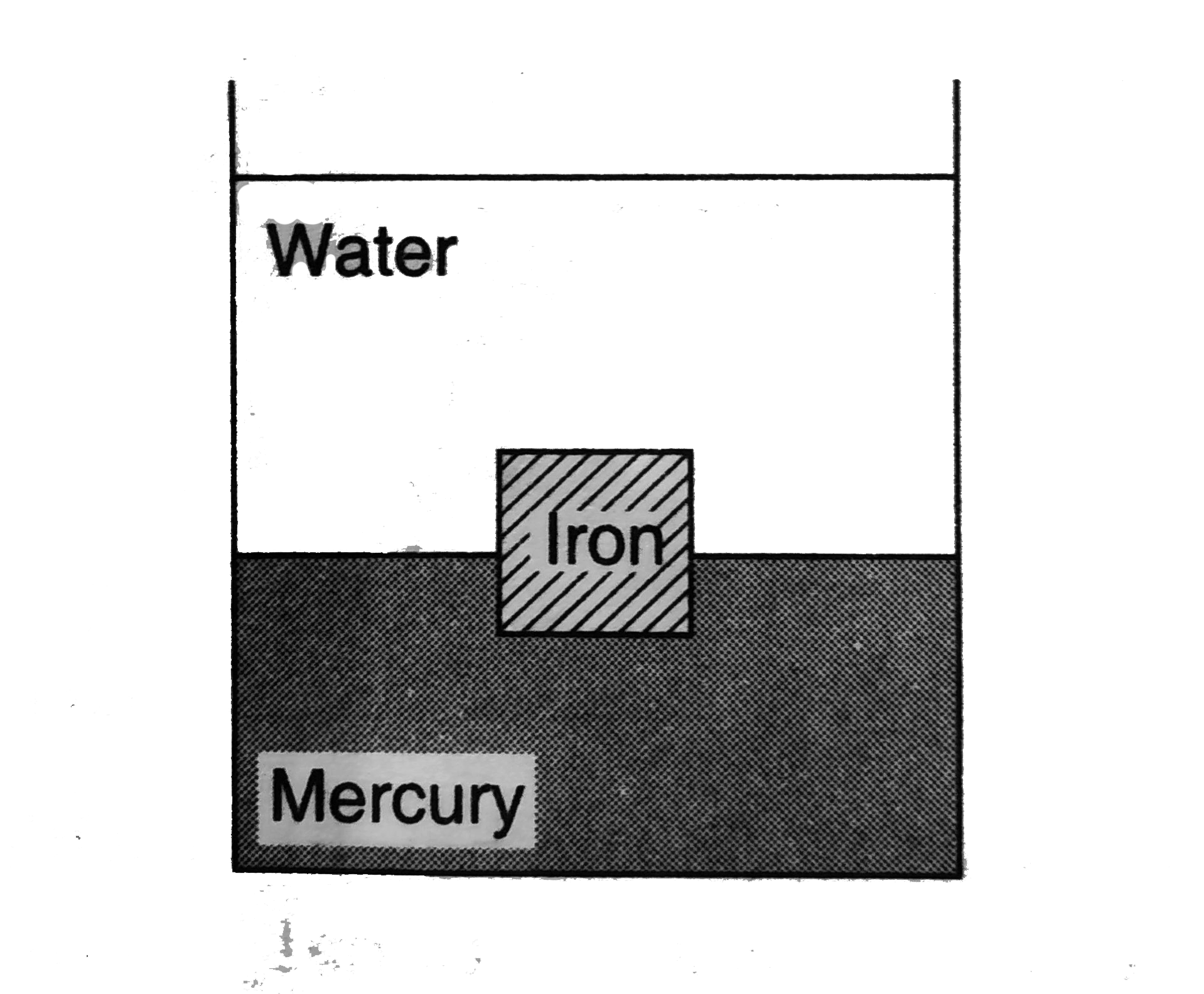
A tank contains water and mercury as shown in . An iron cube of edge 6cm is in equilibrium as shown. What is the fraction of cube inside the mercury ? Given density of iron =7.7×103kgm−3 and density of mercury =13.6×103kgm−3.
To solve the problem, we need to determine the fraction of the iron cube that is submerged in mercury. We will use the principle of buoyancy and the equilibrium condition of the cube.
1. Identify the Variables:
- Edge length of the iron cube, L=6cm=0.06m
- Density of iron, ρiron=7.7×103kg/m3
- Density of mercury, ρmercury=13.6×103kg/m3
- Let x be the height of the cube submerged in mercury.
- The height of the cube submerged in water will then be L−x=0.06−x.
2. Calculate the Weight of the Iron Cube:
Weight of the cube=Volume×Density×g=L3×ρiron×g
=(0.06)3×(7.7×103)×g
3. Calculate the Buoyant Force from Mercury:
Buoyant force from mercury=Volume submerged in mercury×Density of mercury×g=(x⋅L2)×ρmercury×g
=(x⋅(0.06)2)×(13.6×103)×g
4. Calculate the Buoyant Force from Water:
Buoyant force from water=Volume submerged in water×Density of water×g=((0.06−x)⋅L2)×ρwater×g
=((0.06−x)⋅(0.06)2)×(103)×g
5. Set Up the Equilibrium Condition:
Since the cube is in equilibrium, the total buoyant force equals the weight of the cube:
Weight of the cube=Buoyant force from mercury+Buoyant force from water
(0.06)3×(7.7×103)×g=(x⋅(0.06)2)×(13.6×103)×g+((0.06−x)⋅(0.06)2)×(103)×g
6. Cancel g and Simplify:
(0.06)3×(7.7×103)=x⋅(0.06)2⋅(13.6×103)+(0.06−x)⋅(0.06)2⋅(103)
(0.06)3×(7.7×103)=(0.06)2(x⋅(13.6×103)+(0.06−x)⋅(103))
7. Factor Out (0.06)2:
(0.06)×(7.7×103)=x⋅(13.6×103)+(0.06−x)⋅(103)
8. Distribute and Rearrange:
(0.06)×(7.7×103)=13.6×103x+0.06×103−103x
(0.06)×(7.7×103)−0.06×103=(13.6×103−103)x
9. Combine Like Terms:
(0.06)×(7.7−1)×103=(12.6×103)x
0.06×6.7×103=12.6×103x
10. Solve for x:
x=0.06×6.712.6≈0.031m=3.1cm
11. Calculate the Fraction of the Cube Inside Mercury:
Fraction=xL=3.1cm6cm≈0.51
Final Answer:
The fraction of the cube inside the mercury is approximately 0.51 or 51%.
---
- Edge length of the iron cube, L=6cm=0.06m
- Density of iron, ρiron=7.7×103kg/m3
- Density of mercury, ρmercury=13.6×103kg/m3
- Let x be the height of the cube submerged in mercury.
- The height of the cube submerged in water will then be L−x=0.06−x.
Weight of the cube=Volume×Density×g=L3×ρiron×g
=(0.06)3×(7.7×103)×g
Buoyant force from mercury=Volume submerged in mercury×Density of mercury×g=(x⋅L2)×ρmercury×g
=(x⋅(0.06)2)×(13.6×103)×g
Buoyant force from water=Volume submerged in water×Density of water×g=((0.06−x)⋅L2)×ρwater×g
=((0.06−x)⋅(0.06)2)×(103)×g
Since the cube is in equilibrium, the total buoyant force equals the weight of the cube:
Weight of the cube=Buoyant force from mercury+Buoyant force from water
(0.06)3×(7.7×103)×g=(x⋅(0.06)2)×(13.6×103)×g+((0.06−x)⋅(0.06)2)×(103)×g
(0.06)3×(7.7×103)=x⋅(0.06)2⋅(13.6×103)+(0.06−x)⋅(0.06)2⋅(103)
(0.06)3×(7.7×103)=(0.06)2(x⋅(13.6×103)+(0.06−x)⋅(103))
(0.06)×(7.7×103)=x⋅(13.6×103)+(0.06−x)⋅(103)
(0.06)×(7.7×103)=13.6×103x+0.06×103−103x
(0.06)×(7.7×103)−0.06×103=(13.6×103−103)x
(0.06)×(7.7−1)×103=(12.6×103)x
0.06×6.7×103=12.6×103x
x=0.06×6.712.6≈0.031m=3.1cm
Fraction=xL=3.1cm6cm≈0.51
The fraction of the cube inside the mercury is approximately 0.51 or 51%.
Topper's Solved these Questions
Similar Questions
Water rises up in a glass capillary upto a heigh 10 cm, while mercury falls down by 3.5 cm in the same capillary. If the angles of contact for water-glass and mercury-glass are taken as 0∘ and 135∘ respectively, compare the surface tensions of water and mercury. [Given density of water = 103 kg m−3, density of mercury = 13.6 ×103 kg m−3]
View SolutionA piece of ice floats in water . What fraction of its volume will be above the surface of water ? Given density of ice =0.90×103kgm−3.
View Solution
Knowledge Check
A tank contains water and mercury as shown in figure. An iron cube of edge d=6 cm is in equilibrium as shown. (density of iron =7.8×103kg/m3, density of mercury =13.6×103kg/m3, density of water =103kgm3)(Neglect the atmospheric pressure)

Time period of small oscillations of block isA2π√dρFe(ρHg−ρW)gB2π√dρFe(ρHg+ρW)gC2π√dρFeρHggD2π√dρFeρwgA tank contains water on top of mercury as shown in figure. A cubical block of side 10 cm is in equilibrium inside the tank. The depth of the block inside mercury is (RD of the material of block =8.56, RD of mercury =13.6)
 A6 cmB5 cmC7 cmD8 cm
A6 cmB5 cmC7 cmD8 cm
A copper cube of mass 0.50kg is weighed in water. The mass comes out to be 0.40kg. Is the cube hollower solid ? Given density of copper =8.96×103kgm−3 and density of water =103kgm−3.
View SolutionA tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively
View SolutionCalculate the root mean square velocity of the molecules of hydrogen at 0∘C and 100∘C. Density of hydrogen at NTP =0.0896kgm−3 and density of mercury =13.6×103kgm−3
View SolutionIf the mercury in the barometer is replaced by water what will be the resulting height of the water column?
Density of water =1000kgm−3 density of mercury =13600kgm−3
View SolutionIf the mercury in the barometer is replaced by water, what will be the resulting height of the water column? Density of water =1000kgm−3 density of mercury =13600kgm−3
View SolutionMercury has an angle of contact equal to 140∘ with soda lime galss. A narrow tube of radius 1.00mm made of this glass is dipped in a through containing mercury. By what amount does the mercury dip down in the tube relative to the mercury surface outside? Surface tension of mercury at the temperature of the experiment is 0.465Nm−1. Density of mercury = 13.6×103kgm−3.
View Solution
