A thin metal disc of mass 0.5 kg and cross sectional area 0.05m2 is placed coaxially with a cylindrical block of height 0.5 m and same cross sectional area. The top face of the cylindrical block is maintained at a constant temperature of 300 K. If the initial temperature of the metal disc is 200 K, how long will it take for its temperature to raise to 250 K? The thermal conductivity of cylinder's material is 10Wm−1K−1 and specific heat capacity of disc's metal is 600Jkg−1K−1
Note: Consider that the thermal conductivity of disc is very high and the system is thermally insulated except the top face of cylinder.
The given situation is shown below:

Here T is the temperature of the disc at any time t. The rate of heat flowing to the disc is
dQdt=KA(T0−T)/h ...(i)
Due to flow of this heat, temperature of the disc increases by dT. Then,
dQdt=ms(dTdt) ...(ii)
Comparing (i) and (ii), we get
KA(T0−T)/h=ms(dTdt)
⇒dTdt=KAhms(T0−T)
Integrating above equation, we get
∫T2T1dTT0−T=KAhms0∫t0dt
where T1 and T2 are the initial and final temperatures of the metal disc, respectively, and t is the time taken
⇒t=hmsKAln(T0−T1T0−T2)
Here , T1=200K
T2=250K,T0=300K
h = 0.5 m, m = 0.5 kg
s=600Jkg−1K−1
K=10Wm−1K−1
A=0.05m2
t=0.5×0.5×60010×0.05ln(300−200300−250)
=300ln(2)=208s
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - NCERT FILE (TEXTBOOK EXERCISE)
25 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - HIGH ORDER THINKING SKILLS AND ADVANCED LEVEL
10 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CONCEPTUAL QUESTIONS
16 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Chapter Practice Test (for Board Examination)
16 Videos
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
A cylindrical block of length 0.4 m and area of cross-section 0.04m2 is placed coaxially on a thin metal disc of mass 0.4 kg and of the same cross - section. The upper face of the cylinder is maintained at a constant temperature of 400 K and the initial temperature of the disc is 300K. if the thermal conductivity of the material of the cylinder is 10watt/m−K and the specific heat of the material of the disc is 600J/kg−K, how long will it take for the temperature of the disc to increase to 350 K? Assume for purpose of calculation the thermal conductivity of the disc to be very high and the system to be thermally insulated except for the upper face of the cylinder.
View SolutionA cylindrical block of length 0.4 m and area of cross-section 0.04m2 is placed coaxially on a thin metal disc of mass 0.4 kg and of the same cross - section. The upper face of the cylinder is maintained at a constant temperature of 400 K and the initial temperature of the disc is 300K. if the thermal conductivity of the material of the cylinder is 10watt/m−K and the specific heat of the material of the disc is 600J/kg−K, how long will it take for the temperature of the disc to increase to 350 K? Assume for purpose of calculation the thermal conductivity of the disc to be very high and the system to be thermally insulated except for the upper face of the cylinder.
View Solution
Knowledge Check
A metal rod of length 2 m has cross sectional areas 2 A and A as shown in figure. The ends are maintained at temperatures 100° C and 70° C . The temperature at middle point C is
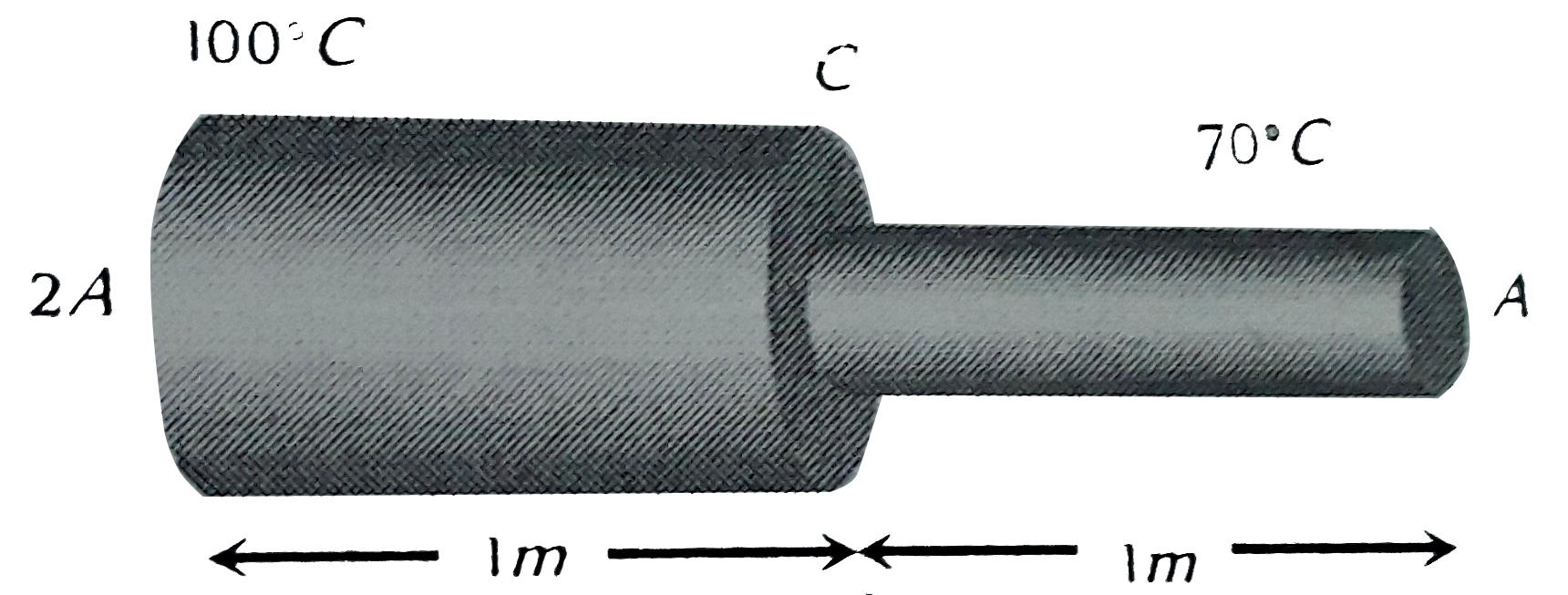 A80∘CB85∘CC90∘CD95∘C
A80∘CB85∘CC90∘CD95∘CThe inductance of a solenoid 0.5m long of cross-sectional area 20cm2 and with 500 turns is
A12.5mHB1.25mHC15.0mHD0.12mHA cylindrical conductor of length l and uniform area of cross section A has resistance R. Another conductor of length 2.5l and resistance 0.5R of the same material has an area of cross section
A5AB2.5AC0.5AD15A
A metal rod of length 2 m has cross sectional areas 2 A and A as shown in figure. The ends are maintained at temperatures 100° C and 70° C . The temperature at middle point C is

View Solution
A cylindrical tube of uniform cross-sectional area A is fitted with two air tight frictionless pistons. The pistons are connected to each other by a metallic wire. Initially the pressure of the gas is P0 and temperature is T0, atmospheric pressure is also P0. Now the temperature of the gas is increased to 2T0, the tension in the wire will be
View SolutionWater is filled in a cylindrical container to a height of 3m. The ratio of the cross-sectional area of the orifice and the beaker is 0.1. The square of the speed of the liquid coming out from the orifice is (g=10m/s2).
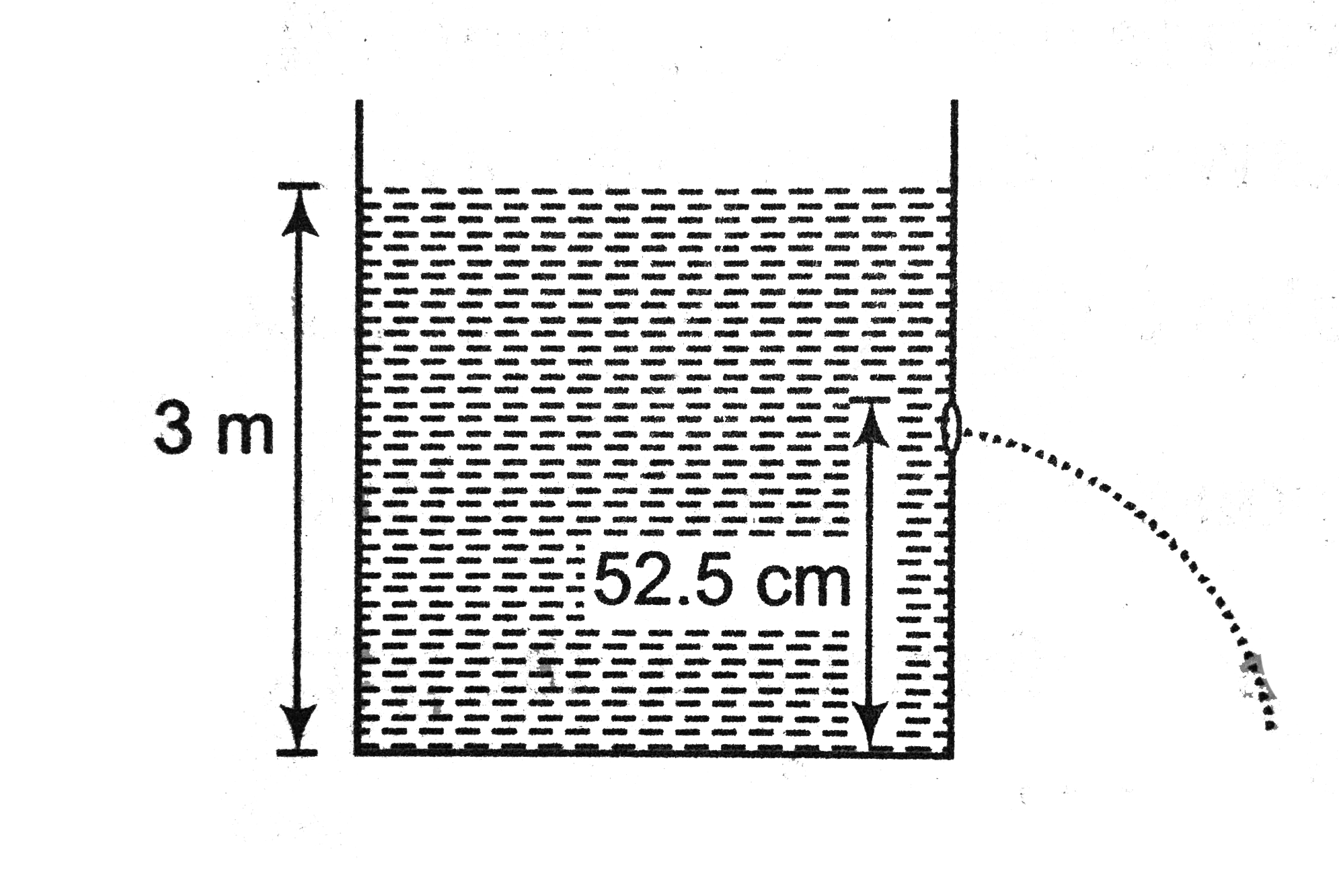
View SolutionA cylindrical conductor of length 'I' and uniform area of cross-section 'A' has resistance 'R'.
Another conductor of length 2.5 I and resistance 0.5 R of the same material has area of crosssection
View Solution
A cylindrical tube of uniform cross-sectional area A is fitted with two air tight frictionless pistons. The pistons are connected to each other by a metallic wire. Initially the pressure of the gas is P0 and temperature is T0, atmospheric pressure is also P0. Now the temperature of the gas is increased to 2T0, the tension in the wire will be
View Solution
