A train has just completed a U-curve in a trach which is a semi circle. The engine is at the forward end of the semi circular part of the trach while the last carriage is at the rear end of the semi circular track. The driver blows a whistle of frequency 200 Hz. Velocity of sound is 340ms. Then the apparent frequency as observed by a passenger in the middle of the train, when the speed of the train is 30 m/s, is
219 Hz
188 Hz
200 Hz
181 Hz
The correct Answer is:C
To find the apparent frequency observed by a passenger in the middle of the train, we can use the Doppler effect formula. Here's a step-by-step solution:
Step 1: Identify the given values
- Frequency of the whistle (f) = 200 Hz
- Velocity of sound (v) = 340 m/s
- Speed of the train (v_s) = 30 m/s
Step 2: Determine the direction of motion
Since the train is moving in a semicircular path and the observer (passenger) is in the middle of the train, we need to consider the relative motion of the source (the whistle) and the observer.
Step 3: Calculate the components of velocity
The train is moving with a speed of 30 m/s. When the train is at the semicircular part, the angle between the direction of the train and the line joining the observer and the source is 45 degrees. Therefore, we need to find the component of the velocity along the line joining the source and the observer.
Using trigonometry:
- The component of the velocity of the train along the line joining them is given by:
vcomponent=vs⋅cos(45∘)=30⋅1√2=30√2≈21.21 m/s
Step 4: Apply the Doppler effect formula
The apparent frequency (f') can be calculated using the Doppler effect formula:
f′=f(v+vov−vs)
Where:
- v = velocity of sound = 340 m/s
- vo = velocity of the observer (passenger) = 30 m/s (since the observer is moving with the train)
- vs = velocity of the source (the whistle) = 30 m/s (the source is also moving with the train)
Substituting the values:
f′=200(340+30340−30)=200(370310)
Step 5: Calculate the apparent frequency
Now, calculate:
f′=200⋅370310≈200⋅1.1935≈238.7 Hz
Final Answer
The apparent frequency as observed by a passenger in the middle of the train is approximately 238.7 Hz.
---
- Frequency of the whistle (f) = 200 Hz
- Velocity of sound (v) = 340 m/s
- Speed of the train (v_s) = 30 m/s
Since the train is moving in a semicircular path and the observer (passenger) is in the middle of the train, we need to consider the relative motion of the source (the whistle) and the observer.
The train is moving with a speed of 30 m/s. When the train is at the semicircular part, the angle between the direction of the train and the line joining the observer and the source is 45 degrees. Therefore, we need to find the component of the velocity along the line joining the source and the observer.
- The component of the velocity of the train along the line joining them is given by:
vcomponent=vs⋅cos(45∘)=30⋅1√2=30√2≈21.21 m/s
The apparent frequency (f') can be calculated using the Doppler effect formula:
f′=f(v+vov−vs)
Where:
- v = velocity of sound = 340 m/s
- vo = velocity of the observer (passenger) = 30 m/s (since the observer is moving with the train)
- vs = velocity of the source (the whistle) = 30 m/s (the source is also moving with the train)
f′=200(340+30340−30)=200(370310)
Now, calculate:
f′=200⋅370310≈200⋅1.1935≈238.7 Hz
The apparent frequency as observed by a passenger in the middle of the train is approximately 238.7 Hz.
Topper's Solved these Questions
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Multiple Correct
44 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Assertion-Reasoning
24 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Subjective
56 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
Similar Questions
The length of a wire which is tied as a boundary of a semi ciruclar park is 72 cm. Find the radius and the area of the semi circular park
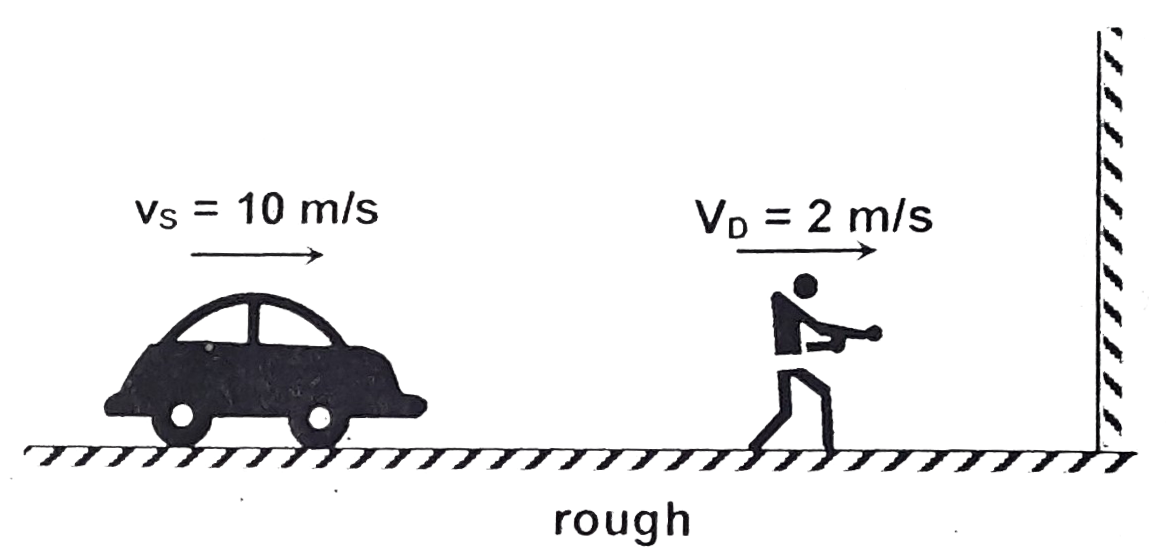
View SolutionA car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
View SolutionThe engine of a train sound a whistle at frequency v, the frequency heard by a passenger is
View SolutionThe engine of a train sound a whistle at frequency v, the frequency heard by a passenger is
View SolutionTwo trains are moving towards each other at speeds of 144 km/hr and 54 km/hr relative to the ground. The first train sounds a whistle of frequency 600 Hz. Find the frequency of the whistle as heard by a passenger in the second train before the trains meet. (y =340m/s)
View SolutionA man is travelling in a train towards the station with a speed of 50 m/s. calculate the apparent frequency heard by him of whistle which is blown at the station with a frequency 200 Hz. Velocity of sound in air is 350 m/s.
View SolutionTrain A and B are approaching towards each other on a parallel track 99 m apart at t = 0 their position are shown. Train A whistles a short pulse of frequency of 596 Hz. Velocity of sound is 330 m/s.

View SolutionA train is moving with a constant speed along a circular track. The engine of the train emits a sound of frequency f. The frequency heard by the guard at the rear end of the train.
View SolutionA source is moving with a constant speed of 10 m/s on a circular track of 200 m. It emits a sound of frequency 200 Hz. A listener stands at the centre of the circular track. The frequency received by the listener is (velocity of sound = 340 m/s)
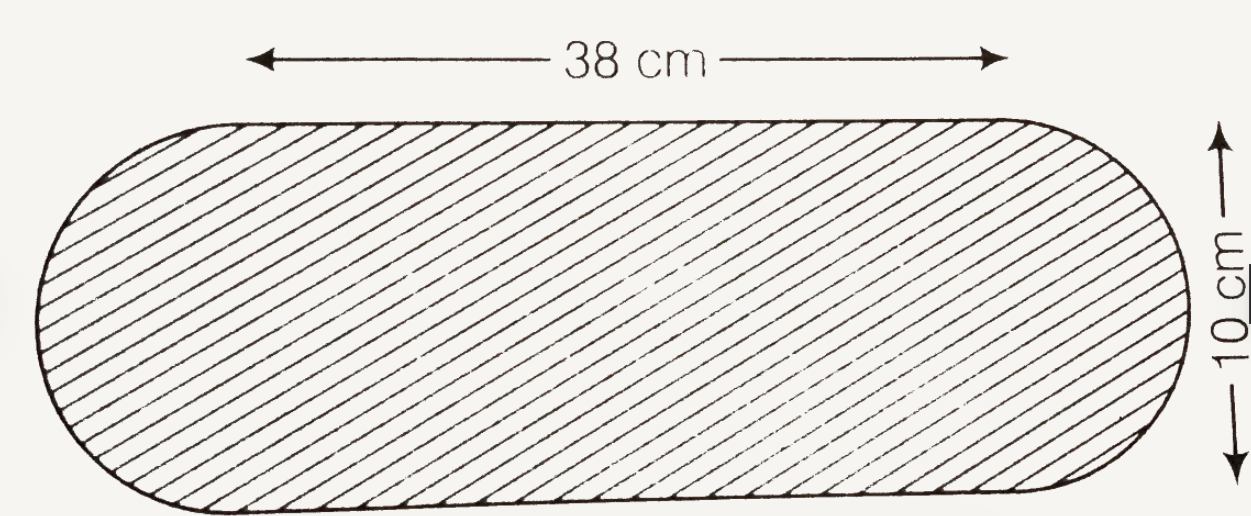
View SolutionFind the area of the flower bed (with semi-circular ends) shown in figure.
View Solution
