A trolley containing a liquid slides down a smooth inclined plane of angle $α$ with the horizontal. Find the angle of inclination $θ$ of the free surface with the horizontal.

A

$θ = \frac{α}{2}$

B

$θ = 2 α$

C

$θ = z e r o$

D

$θ = α$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

The acceleration of the trolley is $a = g sin θ$ down the inclined plane
$a_{x} = a cos α = (g sin α) cos α$
$a_{y} = a sin α = (g sin α) sin α = g {sin}^{2} α$
Let $θ$ be the angle made by free surface of liquid, then
$tan θ = \frac{a_{x}}{g - a_{y}} = \frac{g sin α cos α}{g - g {sin}^{2} α} = tan α$
$θ = α$

|

Updated on:21/07/2023

Class 11 PHYSICS FLUID MECHANICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A trolley containing a liquid slides down a smooth inclined plane of angle $α$ with the horizontal. Find the angle of inclination $θ$ of the free surface with the horizontal.

AEqual to $α$
BGreater than $α$
CEqual to $\frac{α}{2}$
DLesser than $α$
Question 2 - Select One
The angle of inclination with the horizontal is
Aangle of banking
Bbanking angle
Cangle of contact
Dboth 'a' and 'b'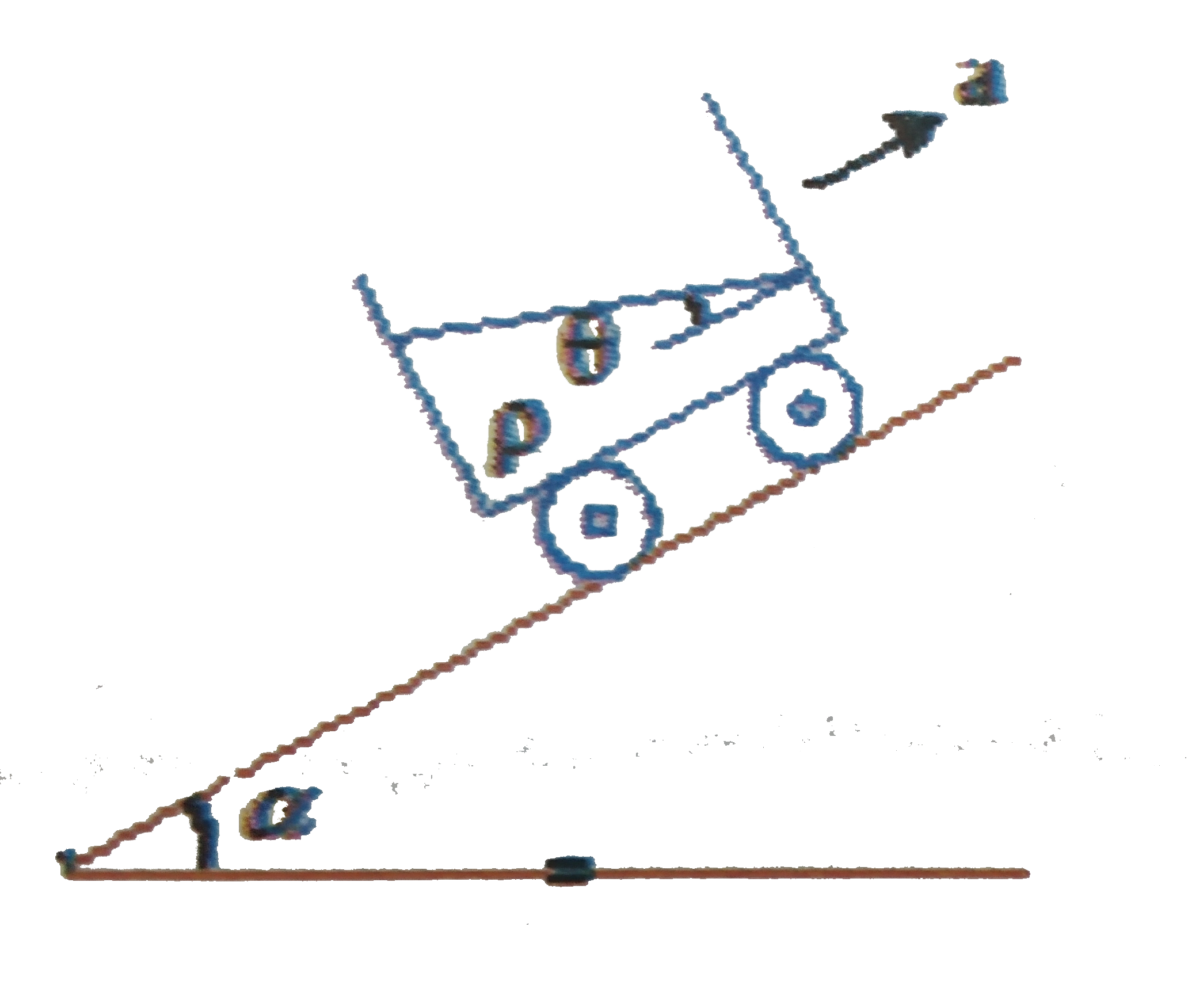
Question 3 - Select One

A fluid container is containing a liquid of density $ρ$ is accelerating upward with acceleration a along the inclined plane of inclination $α$ as shown in the figure. Then the angle of inclination $θ$ of free surface is
A ${tan}^{- 1} [\frac{a}{g cos α}]$
B ${tan}^{- 1} [\frac{a + g sin α}{g cos α}]$
C ${tan}^{- 1} [\frac{a - g sin α}{g (1 + cos α)}]$
D ${tan}^{- 1} [\frac{a - g sin α}{g (1 - cos α)}]$

CENGAGE PHYSICS-FLUID MECHANICS-Exercise 4.2

Home
Ask Doubt
Profile