A turn of radius 20 m is banked for the vehicles going at a speed of 36km/h. If the coefficient of static friction between the road and the tyre is 0.4, what are the possible speeds of a vehicle so that it neither slips down nor skids up?
The correct Answer is:A, B, D
Banking angle, θ=tan−1(v2Rg)
36Km/h=10m/s
θ=tan−1(10020×9.8)=27∘
Angle of repose,
θr=tan−1(μ)=tan−1(0.4)=21.8∘
Sinne θ>θr , vehicle cannot remain in the given position with v=0 . At rest it will slide down. To find minimum speed, so that vehicle does not slip down, maixmum friction will act up the plane.
To find maximum speed, so that the vehicle does not skid up, maximum friction will act down the plane.
Minimum Speed
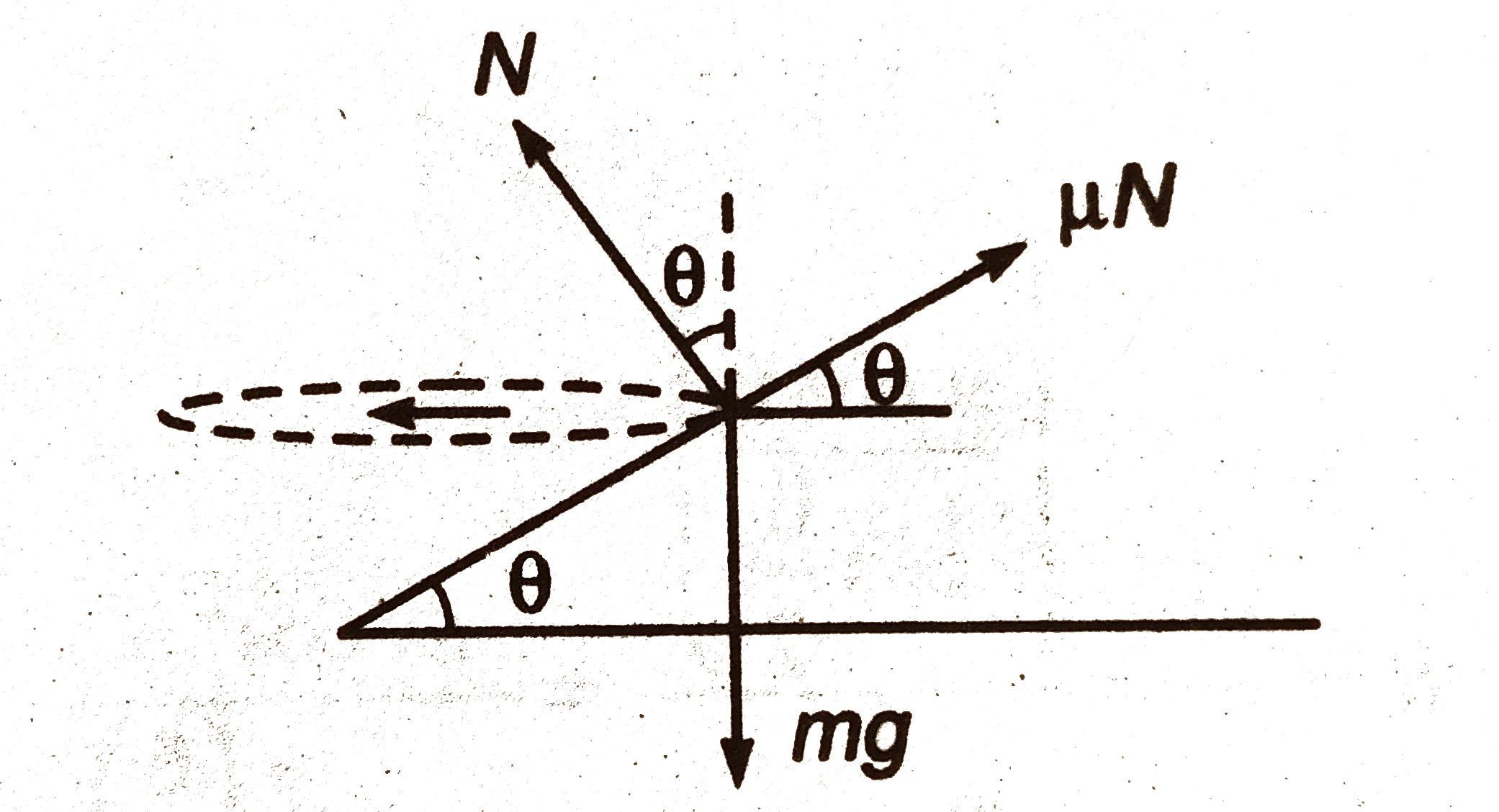
Equation of motion are,
Ncosθ+μNsinθ=mg ...(i)
Nsinθ−μNcosθ=mRv2max...(ii)
Solving these two equations, we get
vmin=4.2m/s
Maximum Speed ltbr> Equations of motion are, Ncosθ−μNsinθ=mg ...(iii)
Nsinθ+μNcosθ=mRv2max ...(iv)
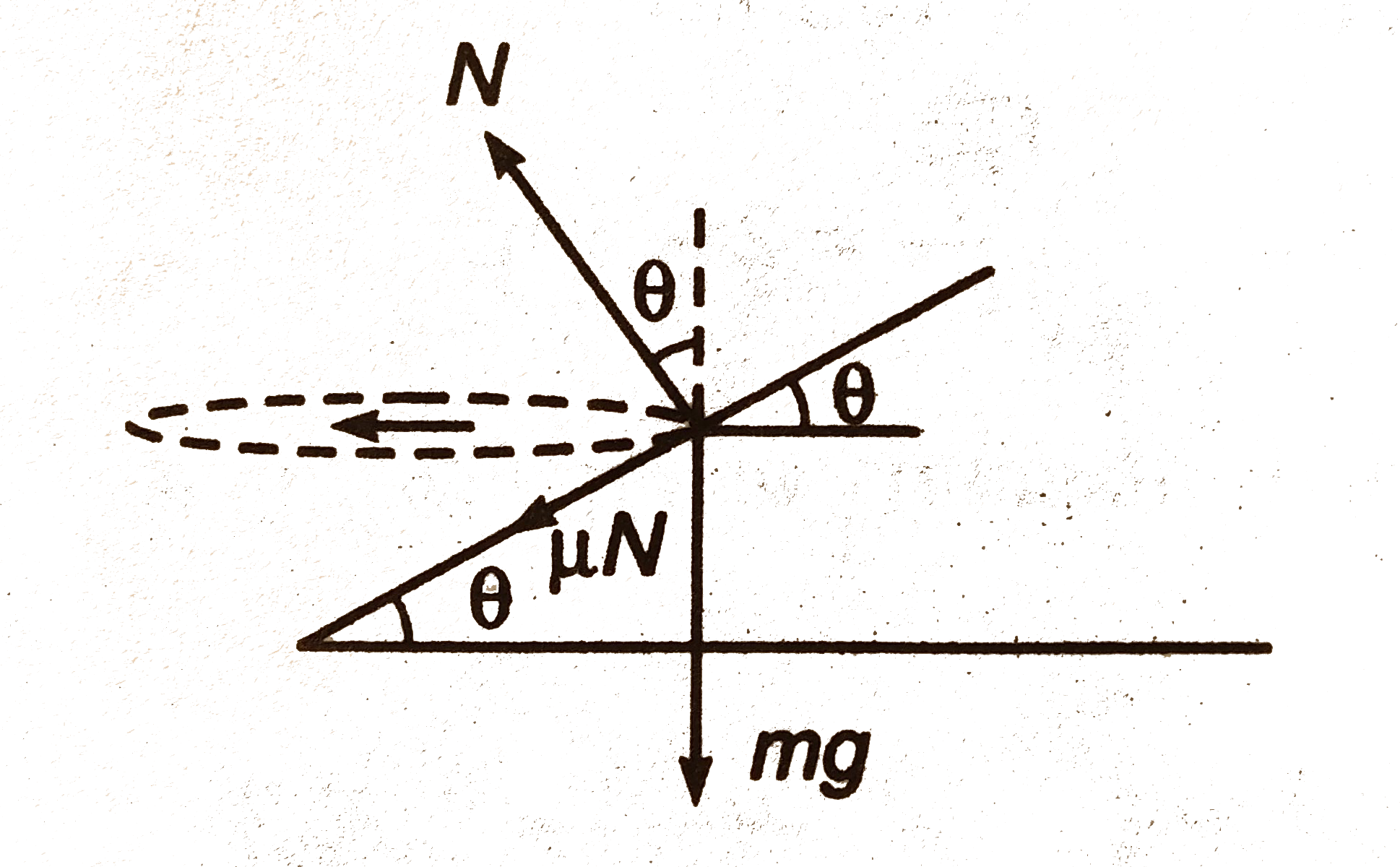
Solving two equations, we have
vmax=15m/s
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Subjective Questions2videos
CIRCULAR MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - JEE Main23videos
CIRCULAR MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 Comprehension Based5videos
CIRCULAR MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective21videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct27videos
COMMUNICATION SYSTEM
Similar Questions
A pick up van negotiates an unbanked curve of radius 40 m with a crate of eggs in the middle of its flat bed. If the coefficient of static friction between the crate and the truck, is 0.55 what is the maximum speed the van can take, so that the crate does not slide ?
View SolutionA 10 metric ton truck drives up a hilly road of gradient 1 in 50 at a speed of 36kmh−1 if the coefficient of kinetic friction between the road and tyres is 0.2, calculate the power delivered by the engine (g=10ms−2)
View SolutionA 10 metric ton truck drives up a hilly road of gradient 1 in 50 at a speed of 36kmh−1 if the co-efficient of kinetic friction between the road and tyres is 0.2, calculate the power delivered by the engine (g=10ms−2)
View SolutionA car has to move on a level turn of radius 45 m. If the coefficient of static friction between the tyre and the road is μs=2.0, find the maximum speed the car can take without skidding.
View SolutionA motorcycle is travelling on a curved track of radius 500 m. If the coefficient of friction between road and tyres is 0.5, the speed avoiding skidding will be
View SolutionA cyclist speeding at 4.5km h−1 on a level road takes a sharp circular turn of radius 3 m without reducing the speed The coefficient of static friction between the road and the tyres is 0.1 will the cyclist slip while taking the turn (i) with a speed of 4.5km h−1 and (ii) with a speed of 9kmk−1?
View SolutionA cyclist speeding at 4.5km h−1 on a level road takes a sharp circular turn of radius 3 m without reducing the speed The coefficient of static friction between the road and the tyres is 0.1 will the cyclist slip while taking the turn (i) with a speed of 405km h−1 and (ii) with a speed of 9kmk−1?
View SolutionA turn of radius 600 m is banked for a vehicle of mass 200 kg going with a speed of 180kmh−1 . Determine the banking angle of its path.
View SolutionA car starts from rest on a horizontal circular road of radius 190 m and gains speed at a uniform rate 1.2m/s2 . The coefficient of static friction between the road and the tyres is 0.37 . Calculate the distance travelled by the car before it begins to skid.
View SolutionA cyclist speeding at 4.5 km/h on a level road takes a sharp circular turn of radius 3 m without reducing the speed. The coefficient of static friction between the road and the tyres is 0.1.
(i) Will he slip while taking the turn?
(ii) Will he slip if his speed is 9 km/h?View Solution

