A uniform chain of length L and mass M overhangs a horizontal table with its two third part n the table. The friction coefficient between the table and the chain is μ. Find the work done by the friction during the period the chain slips off the table.
Let at a certain instant length c of the string is hanging vertically as shown in figure .

Let N be the normal reaction applied by the table on portion of string lyng on the table . Normal reaction will be equal to the weight of string lying on the ytable .
N=mL(L−x)g
Instanteneous force of friction applied by teh table can be written as follows :
F=μmL(L−x)g
Let string further slip off by a distance dx , then for this duration we can assume friction to be constant and work done can be written as follows :
dW=−Fdx=−μmL(L−x)gdx
Negative sign is due to thef fact that force and displacement are opposite to each other . Total work done by the friction can be calculated by integrating above relation nder suitable limits .
⇒ W=μmgL∫LL/3(L−x)dx
⇒ W=μmgL[Lx−x22]LL/3
⇒ W=−μMgL[{L2−L22}{L23−L218}]
⇒ W=−μmgL[{L22}]−{5L218}]
⇒ W=−2μmgL9
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Revision Exercise (Very Short Answer Questions)31videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Revision Exercise (Additional Questions)3videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Exemplar Problems (Short Answer Type Questions))10videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
WAVES
Similar Questions
AS uniform chain of length L and mass M overhangs a horizontal table with its two third part n the table. The friction coefficient between the tabe and the chian is μ. Find the work done by the friction during the period the chain slips off the table.
View SolutionA uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is µ. The work done by the friction during the period the chain slips off the table is [−2kμMgL]. Find the value of k.
View Solution
Knowledge Check
- Question 1 - Select One
A uniform rope of total length l is at rest on a table with fraction f of its length hanging (see figure). If the coefficient of friction between the table and the chain is μ then
 Af=μBf=1/(1+μ)Cf=1/(1+1/μ)Df=1/(μ+1/μ)
Af=μBf=1/(1+μ)Cf=1/(1+1/μ)Df=1/(μ+1/μ) - Question 2 - Select One
A chain is placed on a rough table, partially hanging, as shown in the figure. The coefficient of static friction between the chain and the table is μ = 0.4. If the maximum possible length of the hanging part is x and the length of the chain is L, then what is the value of Lx?
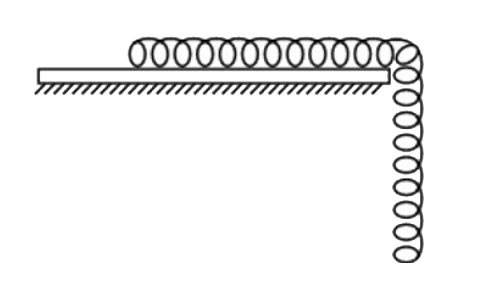 AμL1+μBμL1−μC(1−μ)L1+μDμL
AμL1+μBμL1−μC(1−μ)L1+μDμL - Question 3 - Select One or More
A uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
AThe acceleration of the chain is (gx/L), where x is the length of the hanging part of chain.BThe acceleration of the chain is (g/L)(L−x), where x is the length of hanging part of chain.CThe velocity of the chain is x√gL, where x is the length of hanging part of chain.DThe velocity of the chain is (L−x)√gL, where x is the length of hanging part of chain.
A uniform chain of length l and mass m overhangs a smooth table with its tow third pat lying on the tabl. Find the kinetic energy of the chain s it completely slips off the table.
View SolutionA uniform chain of mass m and length l overhangs a table with its two third part on the table. Fine the work to be done by a person to put the hanging part back on the table.
View SolutionA uniform chain of length L is lying partly on a table, the remaining part hanging down from the edge of the table. If the coefficient of friction between the chain and the table is 0.5 what is the minimum length of the chain that should lie on the table, to prevent the chain from slipping down to the ground.
View SolutionA uniform chain of mass m and length l is at the verge of sliding under the effect of gravity of the hanging part. Find the
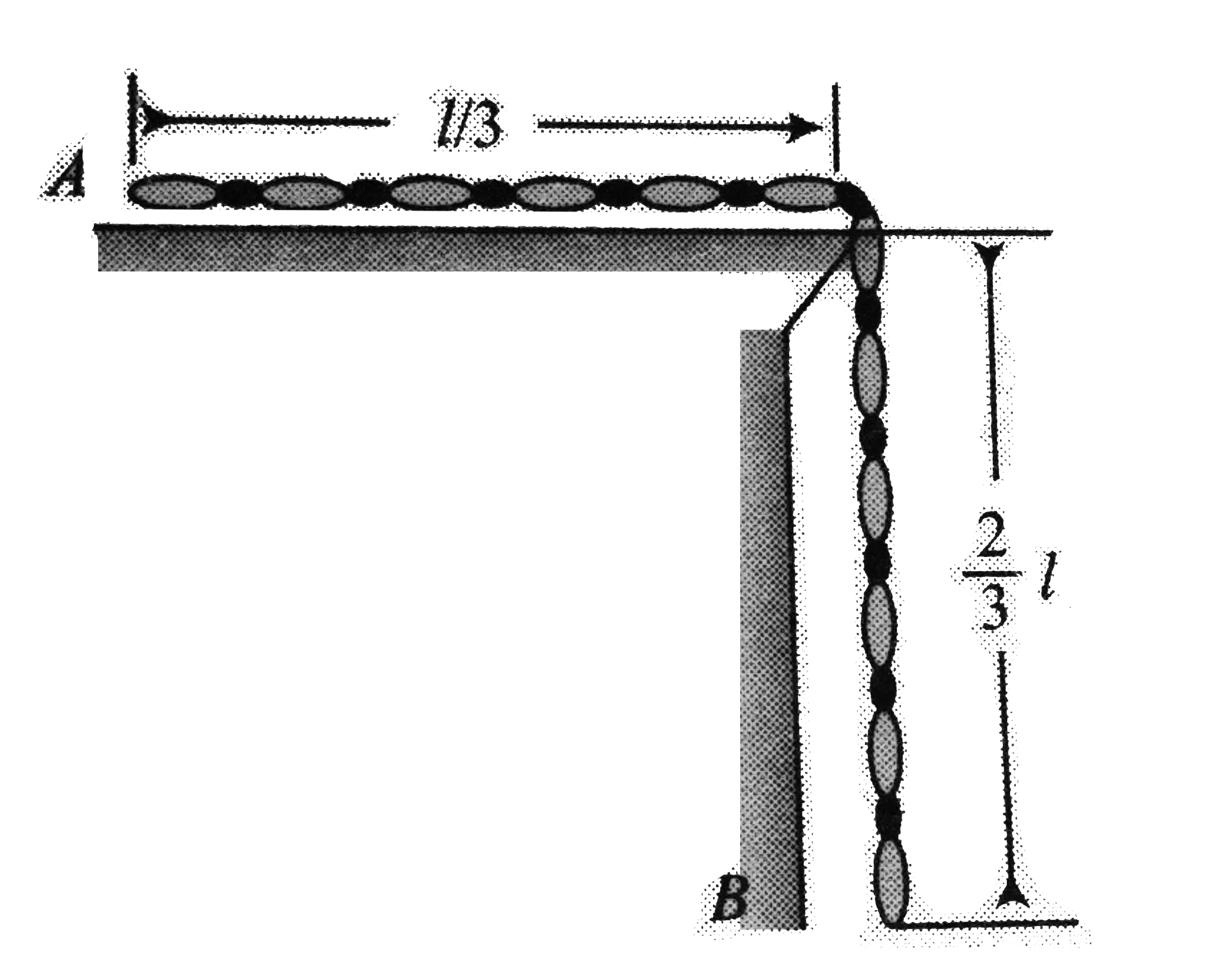
a. coefficient of friction, between chain and table.
b. work done by friction and gravity till the chain leaves the table if the hanging part is pulled gently and released.
c. speed of the chain at the time of leaving the table in part (b) .
d. work done by the external force acting at the end A of the chain in slowly pulling the chain completely onto the table.View SolutionA uniform chain of mass m and length I is kept on the table with a part of it overhanging (see figure). If the coefficient of friction between the table and the chain is 1/3 then find the maximum length of the chain that can overhang such that the chain remain in equilibrium.
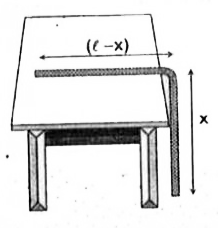 View Solution
View Solution

