Question

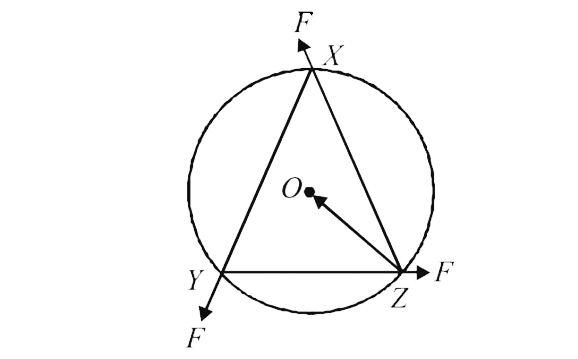
A uniform circular disc of mass 1.5 kg and raius 0.5 m is initially ar rest on a horiozntal frictonless surface. Three forces of equal matgnitude F = 0.5 N are applied simultaneously along the three sides of an equilateral triangle XYZ with its vertices on the perimeter of the disc (see figure). One second after applying the forces the angular speed of the disc in $rad{s}^{-1}$ is

Answer

`(2)`

Explanation

Torque on disc due to all three forces is
`tau = 3 xx Fe sin 30^(@) = 3 xx 0.5 xx 0.5 xx 1//2 = (3)/(8)`
Moment of inertia of disc about an axis passing through its centre and perpendicualr to the plane
`I = (M r^(2))/(2) = (1.5 xx (0.5)^(2))/(2) = (3)/(16)`
If `alpha` is the angular acceleration of the disc due to torque, then
`tau = I alpha` or `alpha = (tau)/(I) = (3//8)/(3//16) = 2 rad//s^(2)`
`omega = omega_(0) + alpha t = 0 + 2 xx 1 = 2 rad//s`