A uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected along the direction of the fields with a certain velocity then

Video Solution

Text Solution

Verified by Experts

Refer to the figure to understand the described situation. We have shown the X-Y plane on paper. Electric field is applied along negative Y-axis, hence it will drive the electron along Y-axis att0. Just after t = 0, the magnetic force starts acting on the particle along X-axis because the magnetic field is directed along negative Z-axis, or inwards in the figure. This way the electron is moved on the X-Y plane. The magnetic force always remains perpendicular to velocity as well as magnetic field and hence electron remains restricted to X-Y plane only and moves along the path as shown in the figure.

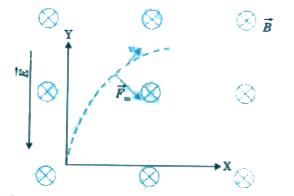
Displacement of electron along Y-axis first increases and then when the velocity of electron becomes perpendicular to Y-axis (or electric field) its Y-coordinate starts decreasing. The meaning of velocity being perpendicular to Y-axis is that the Y-component of velocity becomes zero for that instant. But firstly, we can understand that the velocity of electron in general is having only two components, which are along X and Y-axes only.
So the velocity of electron at any instant of time can be written as follows:
$\to v = v_{x} ˆ i + v_{y} ˆ j$
Now, the Lorentz force on electron can be written as follows:
$\to F = - e \to E - e \to v \times \to B$
$\Rightarrow \to F = - e (- E ˆ j) - e (v_{x} ˆ i + v_{y} ˆ j) \times (- B ˆ k)$
$\Rightarrow \to F = e E ˆ j + e (v_{x} B ˆ i + v_{y} B ˆ j) \times (ˆ k)$
$\Rightarrow \to F = e E ˆ j - e B v_{x} ˆ j + e B v_{y} ˆ i$
$\Rightarrow \to F = e B v_{y} ˆ i + (e E - e B v_{x}) ˆ j$
Hence, we have got the X and Y-components of instantaneous force acting on the electron. Hence we can write the following equations:
$m \frac{d v}{d t} = e B v_{y} \Rightarrow \frac{d v}{d t} = \frac{e B}{m} v_{y}$ ...(i)
$m \frac{d v}{d t} = e E - e B v_{x} \Rightarrow \frac{d v_{y}}{d t} = \frac{e E}{m} - \frac{e B}{m} v_{x}$ ...(ii)
Let us differentiate equation (ii) to get the following:
$\frac{d^{2} v_{y}}{{d t}^{2}} = - \frac{e B}{m} \frac{d v}{d t}$ .....(iii)
Now substituting $\frac{d v_{x}}{d t}$ from equation (i) in equation (iii) we get the following :
$\frac{d^{2} v_{y}}{{d t}^{2}} = - \frac{e B}{m} (\frac{e B}{m} v_{y})$
$\Rightarrow \frac{d^{2} v_{y}}{{d t}^{2}} = - {(\frac{e B}{m})}^{2} v_{y}$
$\Rightarrow \frac{d^{2} v_{y}}{{d t}^{2}} = - ω^{2} v_{y}$ ...(iv)
Here, $ω = \frac{e B}{m}$
We can see that equation (iv) is same as the equation of SHM. Thus, we can write $v_{y}$ , as follows:
$v_{y} = A sin (ω t + δ)$ ......(v)
We have two conditions to be used for equation (v).
At $t = 0, v_{y} = 0 and at t = 0, a_{y} = d v_{y} / d t = e E / m$ .
On substituting these two conditions in equation (v) we get the following:
$δ = 0 and A = e E / m ω = E / B$ . Hence, the Y-component of velocity can finally be written as follows:
$v_{y} = \frac{E}{B} sin ω t$ .....(vi)
We have to find maximum displacement along Y-axis, which is the instant when
$v_{y} = 0$
From equation (vi) we get
$sin ω t = 0 \Rightarrow ω t = π \Rightarrow t = π / ω = π m / e B$ Further , we can write equation (vi) as follows :
$\frac{d y}{d t} = \frac{E}{B} sin ω t \Rightarrow d y = \frac{E}{B} sin ω t d t$
$\Rightarrow y \int 0 d y = \frac{E}{B} t = π m / e B \int 0 sin ω t d t$
$\Rightarrow y = \frac{E}{B} {[- \frac{cos ω t}{ω}]}_{0}^{π m / e B}$
$\Rightarrow y = \frac{E}{B ω} {[- cos ω t]}_{0}^{π m / e B}$
$\Rightarrow y = \frac{E}{B \frac{e B}{m}} [1 - cos (\frac{e B}{m} \frac{π m}{e B})] = \frac{2 m E}{e B^{2}}$

Updated on:21/07/2023