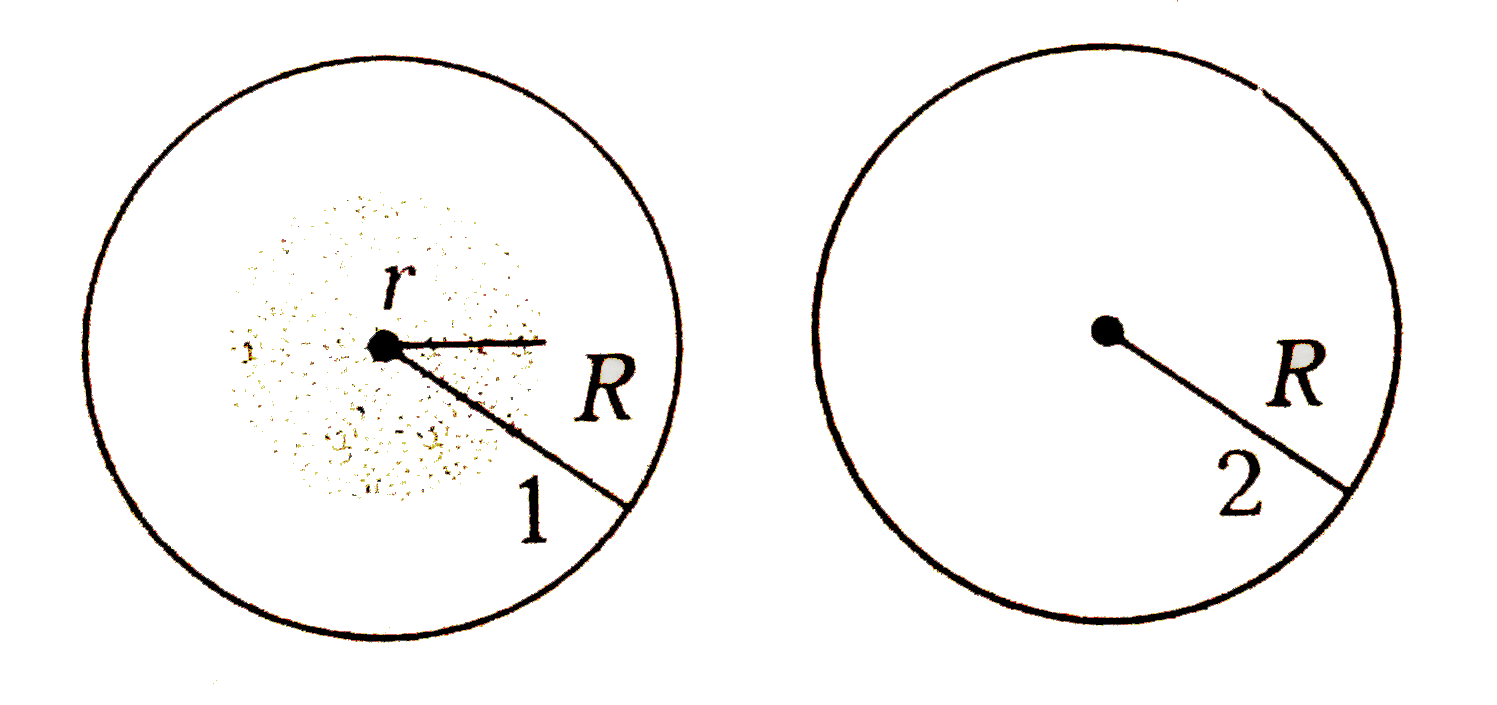
A uniform magnetic field is restricted within a region of radius r. The magnetic field changes with time at a rate dBdt. Loop 1 of radius R > r encloses the region r and loop 2 of radius R is outside the region of magnetic field as shown in figure. Then, the emf generated is
(a) To calculate the field magnitude E, we apply Faraday.s law in the form of Eq. 30-22. We use a circular path of integration with radius r≤R because we want E for points within the magnetic field. We assume from the symmetry that →E in Fig. 30-22b is tangent to the circular path at all points. The path vector d→s is also always tangent to the circular path, so the dot product →E.d→s in Eq. 30-22 must have the magnitude E ds at all points on the path. We can also assume from the symmetry that E has the same value at all points along the circular path. Then the left side of Eq. 30-22 becomes
∫o→E.d→s=∫oEds=E∫ods=E(2πr) (30-25)
(The integral ∫o ds is the circumference 2πr of the circular path.)
Next, we need to evaluate the right side of Eq. 30-22. Because →B is uniform over the area A encircled by the path of integration and is directed perpendicular to that area, the magnetic flux is given by Eq. 30-2:
ϕB=BA=B(πr2) (30-26)
Substituting this and Eq. 30-25 into Eq. 30-22 and dropping the minus sign, we find that
E(2πr)=(πr2)dBdt
or E=r2dBdt
or E=r2dBdt (30-27)
Equation 30-27 gives the magnitude of the electric field at any point for which r≤R (that is, within the magnetic field). Substituting given values yields, for the magnitude of →E at r = 5.2 cm,
E=(5.2×102m)2(0.13T/s)
= 0.0034 V/m= 3.4mV/m.
(b) We can now write
ϕB=BA=B(πR2) (30-28)
Substituting this and Eq. 30-25 into Eq. 30-22 (without the minus sign) and solving for E yield
E=R22rdBdt
Because E is not zero here, we know that an electric field is induced even at points that are outside the changing magnetic field, an important result that (as you will see in Section 31.9) makes transformers possible.
With the given data, Eq. 30-29 yields the magnitude of →E at r = 12.5 cm:
E=(8.5×10−2m)2(2)(12.5×10−2m)(0.13T/s)
=3.8×10−3V/m=3.8mV/m=3.8mV/m
Because Eis not zero here, we know that an electric field is induced even at points that are outside the changing magnetic field, an important result that (as you will see in Section 31.9) makes transformers possible. With the given data, Eq. 30-29 yields the magnitude of E at r = 12.5 cm:
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHECKPOINTS9videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PROBLEMS67videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type1videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT EXERCISE - Practice Questions (Integer)7videos
ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT
Similar Questions
A uniform magnetic field is restricted within a region of radius 1m. The magnetic field changes with time(t) as →B=B0(2t3)ˆk. A loop of radius 2m is outside the region of magnetic field as shown in figure. The emf induced in loop is
_PHY_E06_055_Q01.png)
View SolutionA current carrying loop of radius R and mass 'm' is placed inside a uniform magnetic field an shown
View Solution
Knowledge Check
- Question 1 - Select One
A uniform magnetic field is restricted within a region of radius r. The magnetic field changes with time at a rate dBdt. Loop 1 of radius R > r encloses the region r and loop 2 of radius R is outside the region of magnetic field as shown in figure. Then, the emf generated is
A−d→BdtπR2 in loop 1 and zero in loop 2B−d→Bdtπr2 in loop 1 and zero in loop 2CZero in loop 1 and zero in loop 2D−d→Bdtπr2 in loop 1 and −d→Bdtπr2 in loop 2 - Question 2 - Select One or More
The magnetic field perpendicular to the plane of a conducting ring of radius r changes at the rate.dBdt . Then
Athe emf induced in the ring is πr2dBdtBthe emf induced in the ring is 2πrdBdtCdiametrically opposite points on the ring are at the same potentialDall points on the ring are at the same potential - Question 3 - Select One
A uniform but time varying magnetic field is present in a circular region of radius R. The magnetic field is perpendicular and into the plane of the loop and the magnitude of field is increasing at a constant rate α. There is a straight conducting rod of length 2R placed as shown in figure.
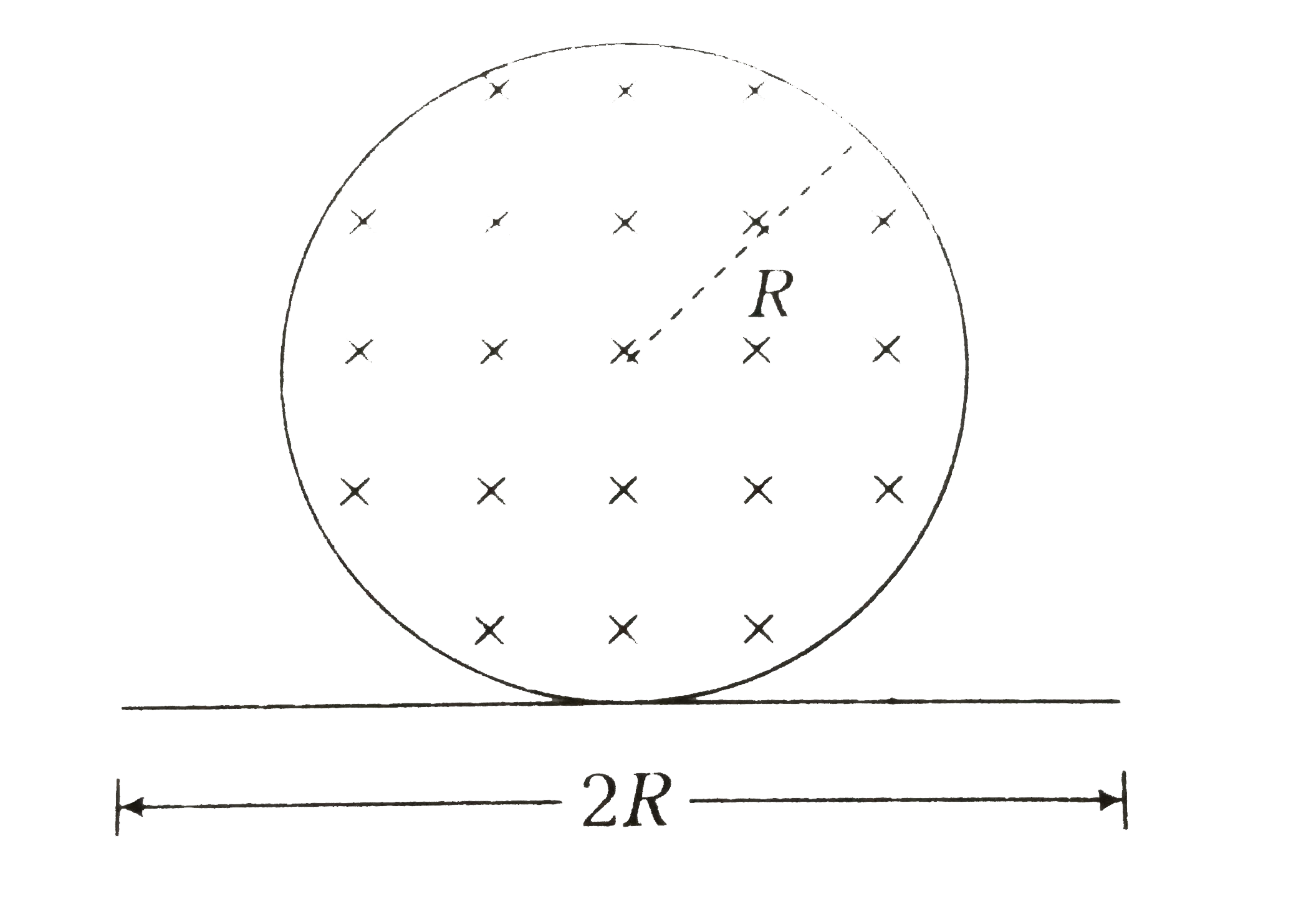
The magnitude of induced emf across the rod isAπR2αBπR2α2CR2α√2Dπr2α4
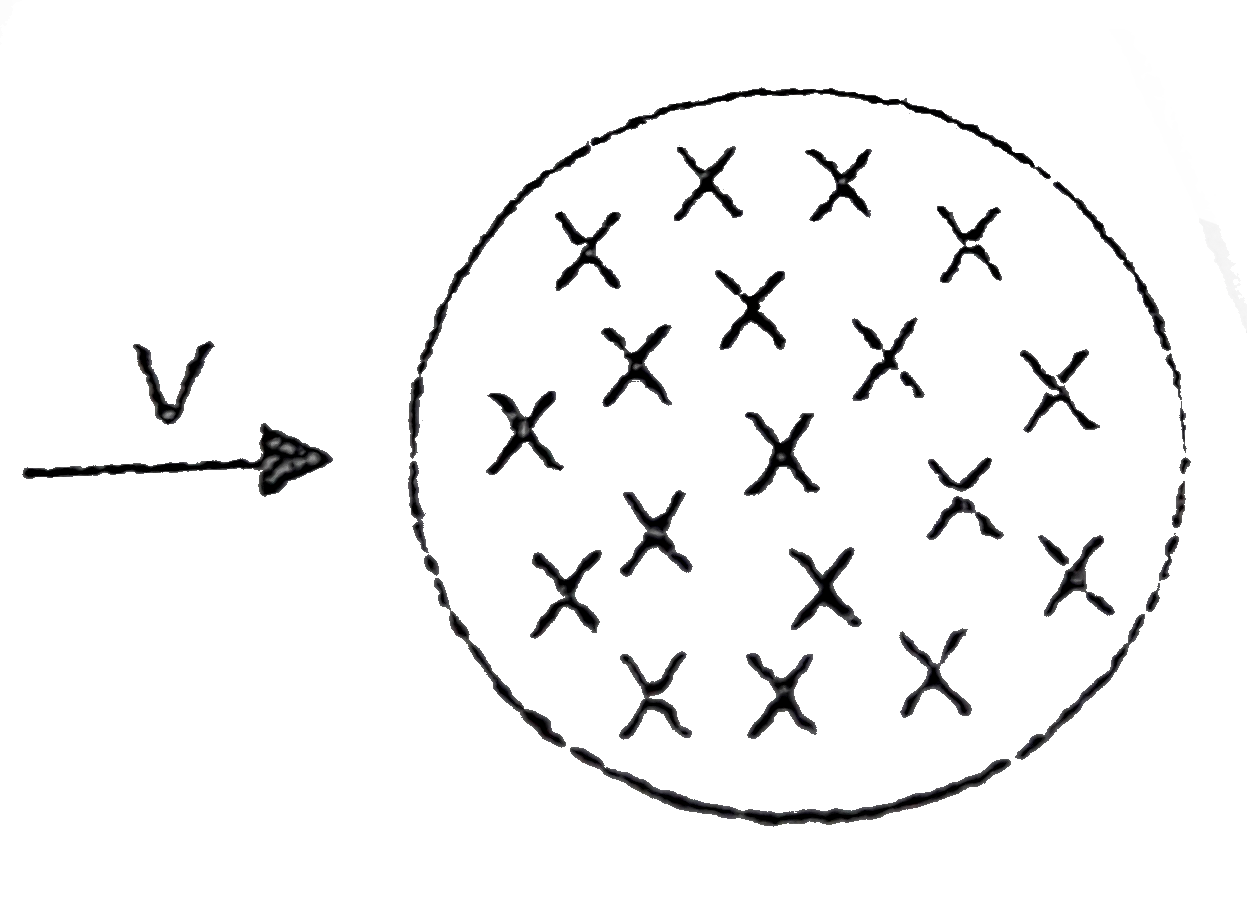
A uniform magnetic field exists in a circular region of radius r.The magnitude of magnetic field is B and points inward.An electron flies into the region radially as shown in figure.After a certain time, the electron deflected by the magnetic field leaves the region.The time interval during which the electron moves in the region is Nmtan−1eBrmVeB.Then find value of N.(m is mass of electron and e is charge of electron)
View SolutionA uniform magnetic field B exists in a circular region of radius R. the field is perpendicular to the plane and is increasing at a constant rate of dBdt=α. There is a straight conducing rod AB of length 2R. Find the emf induced in the rod when it is placed as shown in figure (a) & (b). Point C is midpoint of the rod in figure (b)
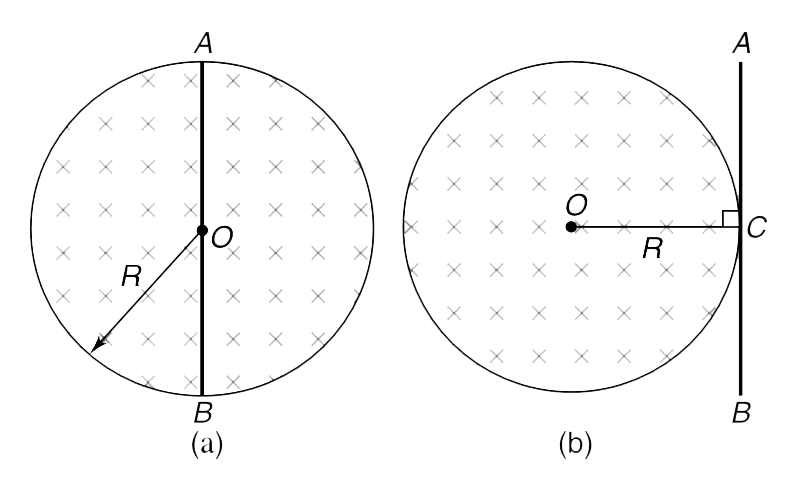View SolutionMagnetic field in cylindrical region of radius R in inward direction is as shown in figure.
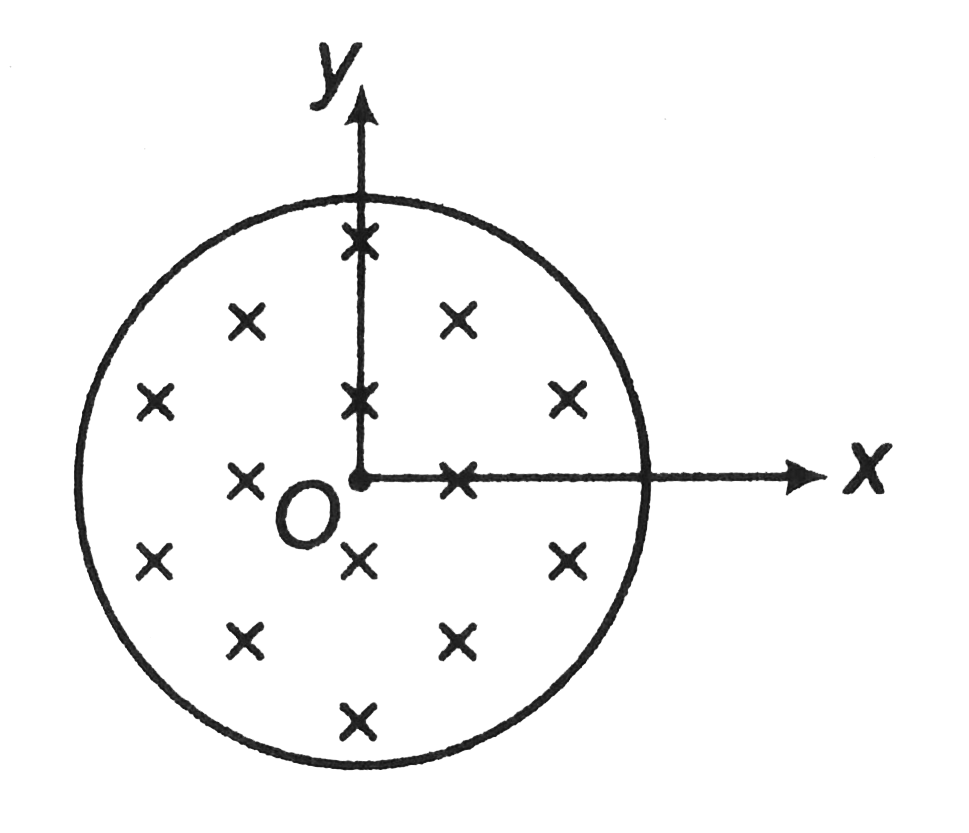View SolutionA uniform but time varying magnetic field is present in a circular region of radius R. The magnetic field is perpendicular and into the plane of the loop and the magnitude of field is increasing at a constant rate α. There is a straight conducting rod of length 2R placed as shown in figure.
The magnitude of induced emf across the rod isView SolutionThe magnetic field perpendicular to the plane of a conducting ring of radius r change ate the rate dBdt
View Solution