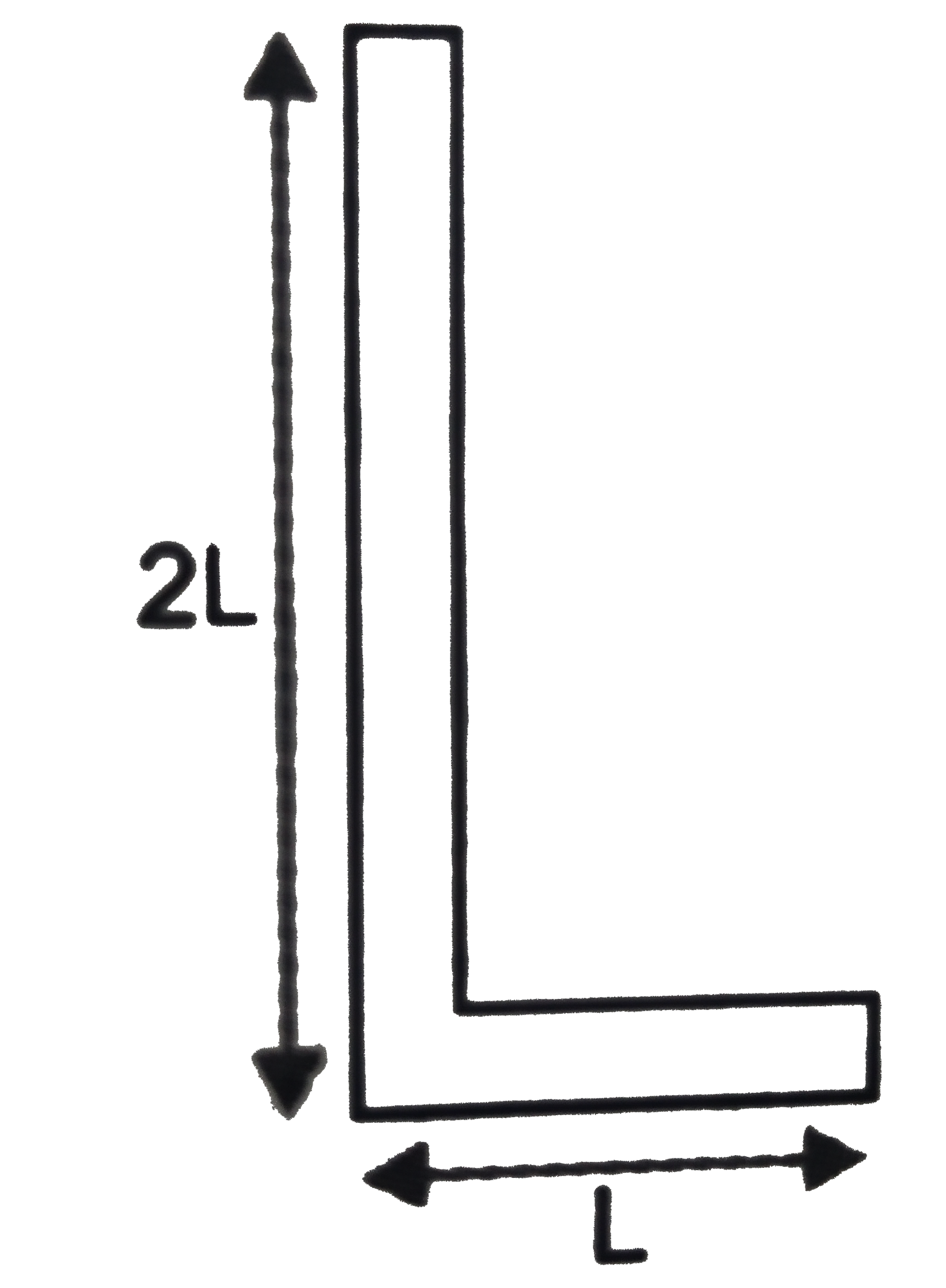
A uniform metal rod of length 1m is bent at 90∘, so as to form two arms of equal length. The centre of mass of this bent rod is
on the bisector of the angle, (1√2) m from vertex
on the bisector of angle, (12√2) m from vertex
on the bisector of the angle, (12) m from vertex
on the bisector of the angle, (14√2)m from vertex
The correct Answer is:D
To find the center of mass of a uniform metal rod bent at a 90-degree angle, we can follow these steps:
Step 1: Understand the Geometry of the Bent Rod
The rod has a total length of 1 meter and is bent at a right angle, forming two arms of equal length. Since the total length is 1 meter, each arm will have a length of:
L=1 m2=0.5 m
Step 2: Define the Coordinate System
We can place the bent rod in a coordinate system. Let's assume:
- Point B (the vertex of the angle) is at the origin (0, 0).
- Point A (end of the first arm) is at (0, 0.5) on the y-axis.
- Point C (end of the second arm) is at (0.5, 0) on the x-axis.
Step 3: Determine the Center of Mass of Each Arm
For each arm, the center of mass is located at its midpoint:
- The center of mass of arm AB (vertical arm) is at:
CMAB=(0,0+0.52)=(0,0.25)
- The center of mass of arm BC (horizontal arm) is at:
CMBC=(0+0.52,0)=(0.25,0)
Step 4: Calculate the Overall Center of Mass
Since the rod is uniform, we can treat each arm as having equal mass. Let’s denote the mass of each arm as m. The coordinates of the center of mass (CM) of the entire bent rod can be calculated using the formula:
XCM=m1x1+m2x2m1+m2
YCM=m1y1+m2y2m1+m2
Substituting the values:
- For the x-coordinates:
XCM=m⋅0+m⋅0.25m+m=0+0.25m2m=0.252=0.125
- For the y-coordinates:
YCM=m⋅0.25+m⋅0m+m=0.25m+02m=0.252=0.125
Step 5: Final Coordinates of the Center of Mass
Thus, the coordinates of the center of mass of the bent rod are:
CM=(0.125,0.125)
Step 6: Distance from the Vertex
To find the distance from the vertex (point B) to the center of mass:
Distance=√(0.125)2+(0.125)2=√0.015625+0.015625=√0.03125=14√2 m
Conclusion
The center of mass of the bent rod is located at a distance of 14√2 meters from the vertex along the bisector of the angle formed by the two arms.
---
The rod has a total length of 1 meter and is bent at a right angle, forming two arms of equal length. Since the total length is 1 meter, each arm will have a length of:
L=1 m2=0.5 m
We can place the bent rod in a coordinate system. Let's assume:
- Point B (the vertex of the angle) is at the origin (0, 0).
- Point A (end of the first arm) is at (0, 0.5) on the y-axis.
- Point C (end of the second arm) is at (0.5, 0) on the x-axis.
For each arm, the center of mass is located at its midpoint:
- The center of mass of arm AB (vertical arm) is at:
CMAB=(0,0+0.52)=(0,0.25)
CMBC=(0+0.52,0)=(0.25,0)
Since the rod is uniform, we can treat each arm as having equal mass. Let’s denote the mass of each arm as m. The coordinates of the center of mass (CM) of the entire bent rod can be calculated using the formula:
XCM=m1x1+m2x2m1+m2
YCM=m1y1+m2y2m1+m2
- For the x-coordinates:
XCM=m⋅0+m⋅0.25m+m=0+0.25m2m=0.252=0.125
YCM=m⋅0.25+m⋅0m+m=0.25m+02m=0.252=0.125
Thus, the coordinates of the center of mass of the bent rod are:
CM=(0.125,0.125)
To find the distance from the vertex (point B) to the center of mass:
Distance=√(0.125)2+(0.125)2=√0.015625+0.015625=√0.03125=14√2 m
The center of mass of the bent rod is located at a distance of 14√2 meters from the vertex along the bisector of the angle formed by the two arms.
Topper's Solved these Questions
- FORCEBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - FORCEEXERCISE - Exercise 1
41 Videos
- ELECTROSTATICSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELECTROSTATICSEXERCISE - MHT CET Corner
29 Videos
- FRICTION IN SOLID AND LIQUIDSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - FRICTION IN SOLID AND LIQUIDSEXERCISE - EXERCISE 2 (Miscellaneous Problems)
24 Videos
Similar Questions
Knowledge Check
A thin uniform rod of length L is bent at its mid point as shown in the figure. The distance of the centre of mass from the point O is
 AL2sin(θ2)BL2cos(θ2)CL4sin(θ2)DL4cos(θ2)
AL2sin(θ2)BL2cos(θ2)CL4sin(θ2)DL4cos(θ2)The density of a non-uniform rod of length 1m is given by ρ(x)=a(1+bx2) where, a and b are constant and 0≤x≤1. The centre of mass of the rod will be at
A3(2+b)4(3b+b)B4(2+b)3(3+b)C3(3+b)4(2+b)D4(3+b)3(2b+b)A uniform rod o length one meter is bent at its midpoint to make 90∘. The distance of centre of mass from the centre of rod is (in cm)
A20.2B13.4C15D17.6
A uniform rod of mass m is bent into the form of a semicircle of radius R. The moment of inertia of the rod about an axis passing through A and perpendicular to the plane of the paper is

View SolutionA rod of mass m and length L is non- uniform.The mass of rod per unit length (mu)], varies as "(mu)=bx" ,where b is a constant.The , centre of mass of the ,rod is given by
View SolutionA uniform metre rod is bent into L shape with the bent arms at 90∘ to each other. The distance of the centre of mass from the bent point is
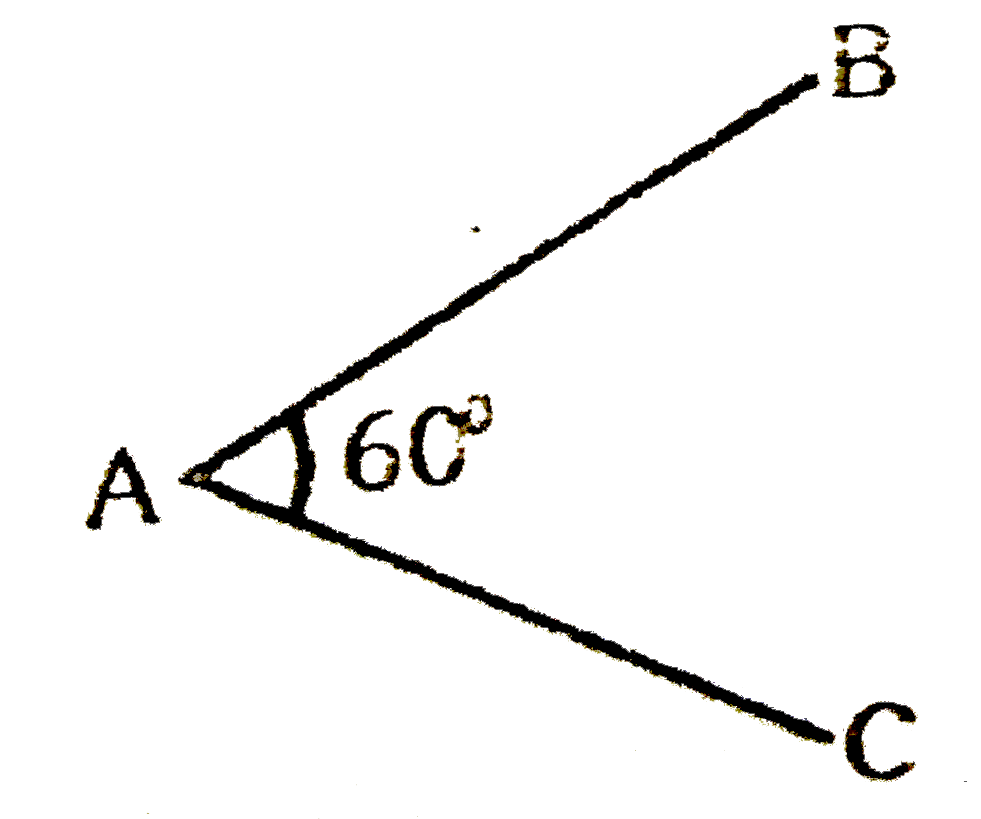
View SolutionA uniform wire of length l is bent into the shape of 'V' as shown. The distance of its centre of mass from the vertex A is :-
View SolutionThe density of a non-uniform rod of length 1m is given by ρ(x)=a(1+bx2)
where a and b are constants and 0≤x≤1.
The centre of mass of the rod will be at
View Solution