A uniform metallic rod PQ of length 2 m is acted upon by two forces A and B along the directions as shown in the figure. Find the magnitude and position of the resultant normal force that acts on the rod.

The forces that act normally to the rod are
Acos60∘=90×12=45N
Bcos60∘=30×12=15N.
As the two forces are unlike parallel forces, the resultant of these two forces is outside the rod at a point 'O' and in the direction of greater force, i.e. , A.
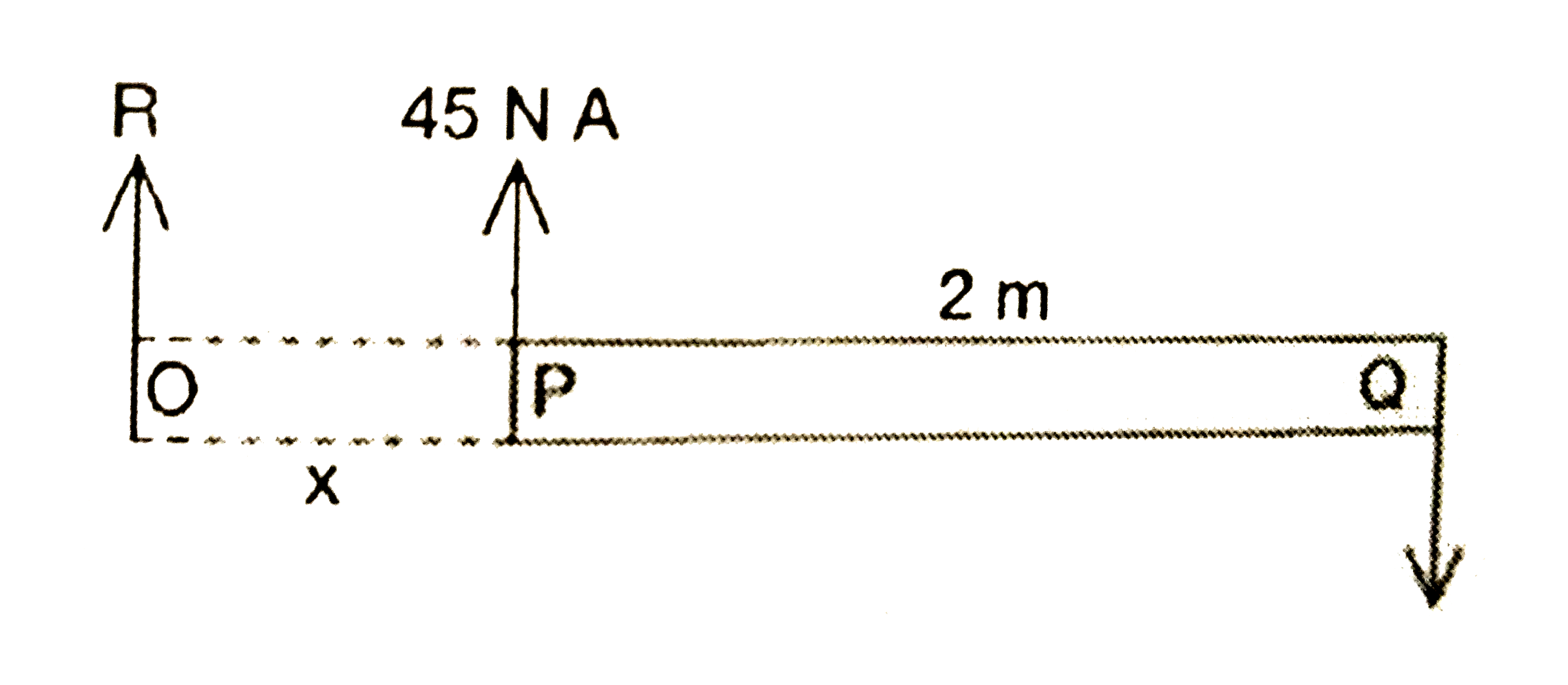
Magnitude of R = 45 - 15 = 30 N
Position of R: Let 'R' is at 'O' and at a distance of x m from 'P'. Then
45×x=15×(2+x)
3x=2+x
2x=2
x=1m
Topper's Solved these Questions
- SIMPLE MACHINESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SIMPLE MACHINESEXERCISE - Level- 3
10 Videos
- SIMPLE MACHINESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SIMPLE MACHINESEXERCISE - Concept Application (Level - 1 )
44 Videos
- MODERN PHYSICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MODERN PHYSICSEXERCISE - Level 3
5 Videos
- WAVES MOTION AND SOUND BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - WAVES MOTION AND SOUND EXERCISE - Level 3
10 Videos
Similar Questions
A uniform metallic rod AB of length 1.4 m is lifted by two forces P and Q acting along the directions as shown in the figure. If the magnitudes of P and Q are 20 N and 50 N, respectively, find the magnitude and the position of the resultant normal force that acts on the rod.

View SolutionThere are four forces acting on a block as shown in the figure ,The magnitude and direction of the net force acting on it is _______.
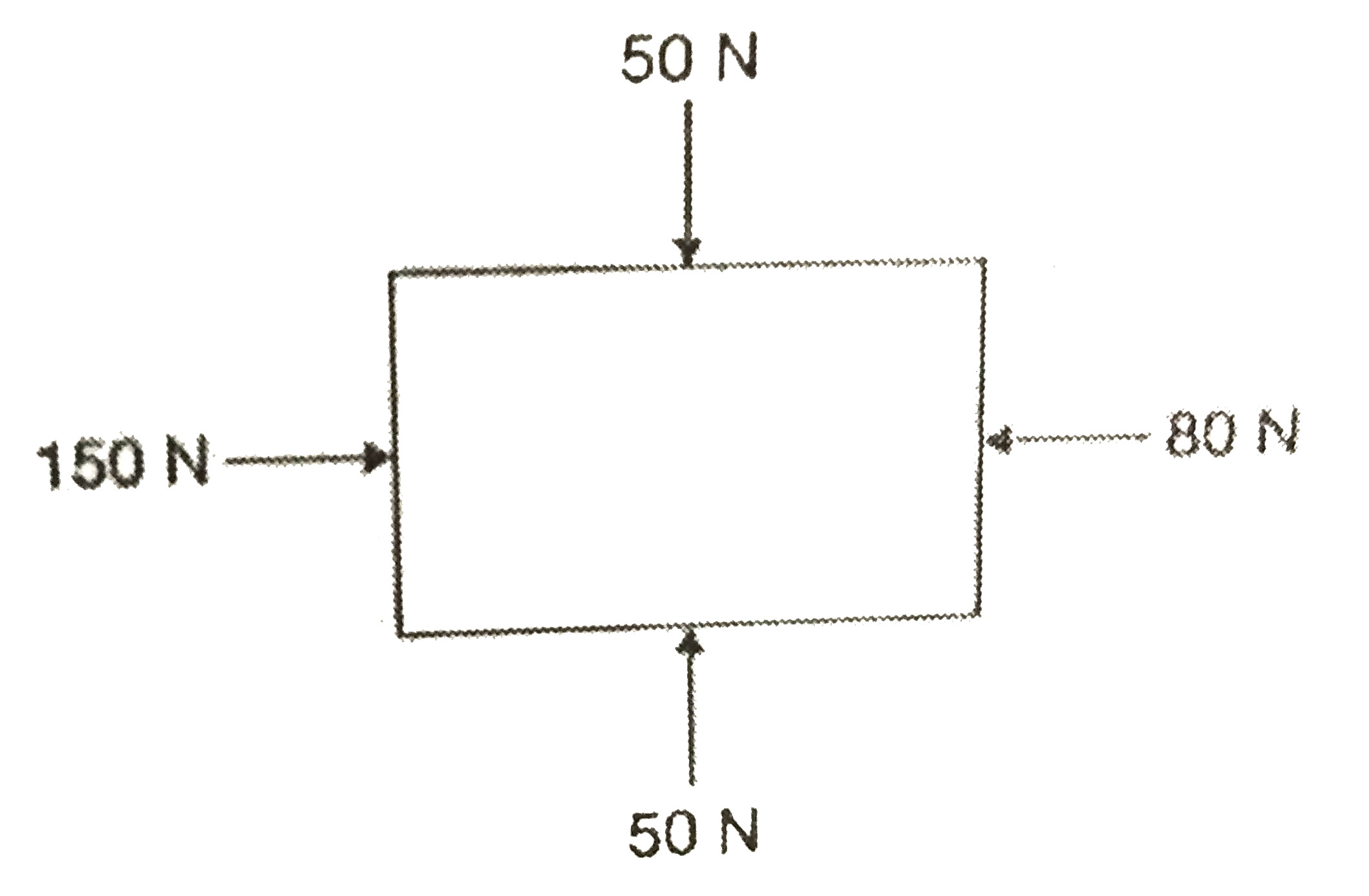
View Solution
Knowledge Check
There are four forces acting on a block as shown in the figure. The magnitude and direction of the net force acting on it is __________.
 A150 N, towards rightB100 N, upwardsC70 N, towards rightD70 N, towards left
A150 N, towards rightB100 N, upwardsC70 N, towards rightD70 N, towards leftA rod of length L and mass M is acted on by two unequal forces F1 and F2(<F)as shown in the figure.
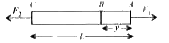
The tension in the rod at a distance y from the end A is given byAF1(1−yL)+F2(yL)BF2(1−yL)+F1(YL)C(F1−F2)yLDNone of theseTwo forces F1 and F2 are acting on a rod abc as shown in figure.
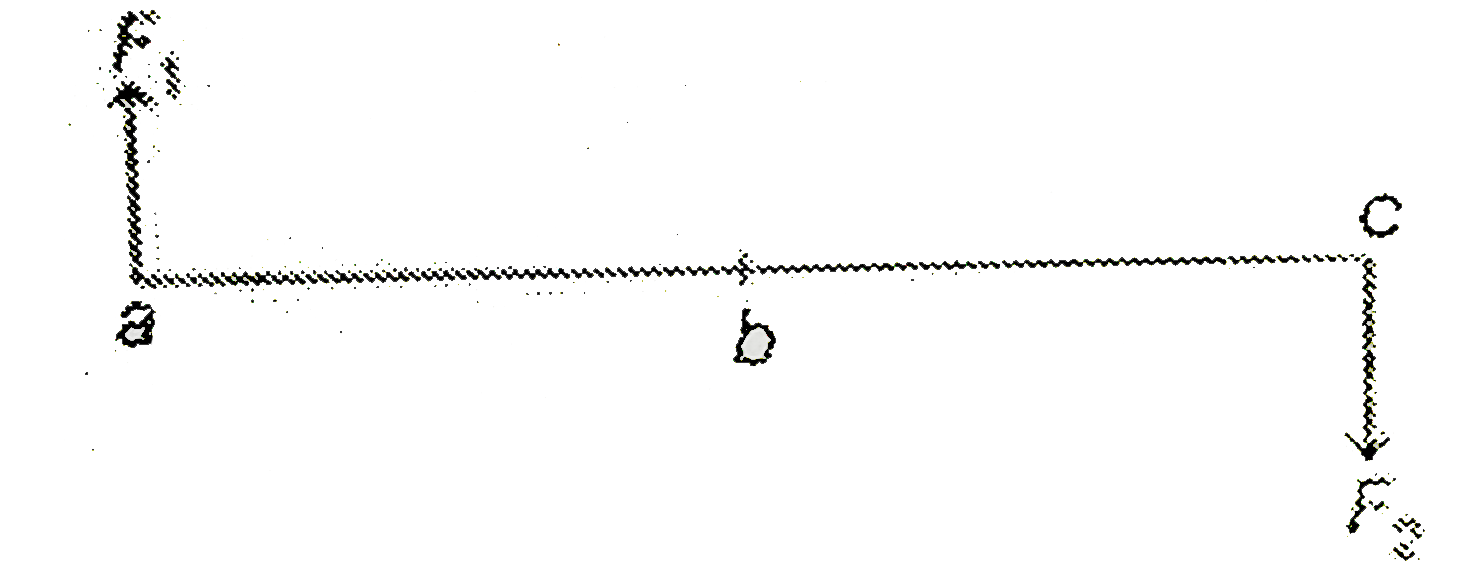 Aif F1=F2 then τa=τb=τc ( for both forces)Bif F1=F2 then τa=τc≠τb ( for both forces)Cif F1≠F2 then τa≠τb≠τc ( for both forces)Dif F1≠F2 then τa=τc≠τb ( for both forces)
Aif F1=F2 then τa=τb=τc ( for both forces)Bif F1=F2 then τa=τc≠τb ( for both forces)Cif F1≠F2 then τa≠τb≠τc ( for both forces)Dif F1≠F2 then τa=τc≠τb ( for both forces)
Calculate the magnitude and the direction of the resultant force acting on an object shown in the picture.

View SolutionA wedge of mass M resting on a horizontal frictionless surface is acted upon by a force F in the horizontal direction as shown in figure. Find the net horizontal force acting on the shaded portion of the wedge.
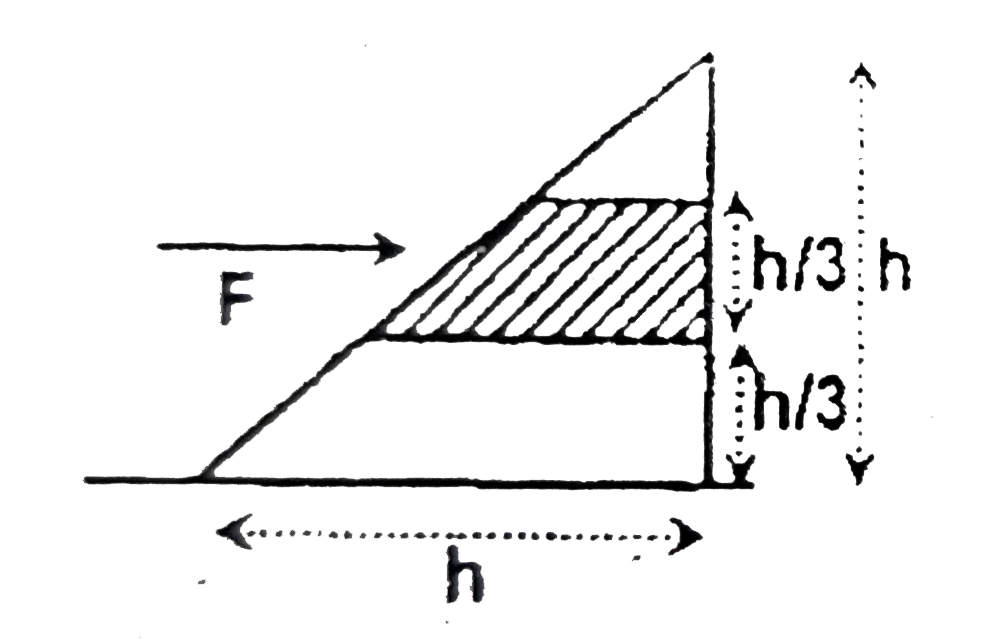
View SolutionIf two forces of 5 N each are acting along X and Y axes, then the magnitude and direction of resultant is
View SolutionA uniform rod of length L pivoted at the bottom of a pond of depth L/2 stays in stable equilibrium as shown in the figure.

Find the force acting at the pivoted end of the rod in terms of mass m of the rod.
View SolutionA rectangular loop is acted upon by two forces of same magnitude but opposite directions. The net force acting on the loop is
View Solution
