A uniform plank of Young's modulus Y is moved over a smooth horizontal surface by a constant horizontal force F. The area of cross section of the plank is A. The compressive strain on the plank in the direction of the force is
F/AY
2F/AY
12(F/AY)
3F/AY
The correct Answer is:A
To solve the problem, we need to determine the compressive strain on a uniform plank when a constant horizontal force F is applied to it. We will use the concept of Young's modulus and the definitions of stress and strain.
1. Understand Young's Modulus:
Young's modulus Y is defined as the ratio of stress to strain in a material. Mathematically, it is given by:
Y=StressStrain
2. Define Stress:
Stress (σ) is defined as the force (F) applied per unit area (A). Thus, we can express stress as:
σ=FA
3. Define Strain:
Strain (ϵ) is defined as the change in length per unit original length. However, in this case, we are interested in the compressive strain due to the applied force.
4. Relate Stress and Strain using Young's Modulus:
From the definition of Young's modulus, we can rearrange the equation to find strain:
Strain=StressY
5. Substitute Stress into the Strain Formula:
Now, substituting the expression for stress into the strain formula:
Strain=σY=F/AY=FAY
6. Conclusion:
Therefore, the compressive strain on the plank in the direction of the force is given by:
Compressive Strain=FAY
Final Answer:
The compressive strain on the plank in the direction of the force is FAY.
Young's modulus Y is defined as the ratio of stress to strain in a material. Mathematically, it is given by:
Y=StressStrain
Stress (σ) is defined as the force (F) applied per unit area (A). Thus, we can express stress as:
σ=FA
Strain (ϵ) is defined as the change in length per unit original length. However, in this case, we are interested in the compressive strain due to the applied force.
From the definition of Young's modulus, we can rearrange the equation to find strain:
Strain=StressY
Now, substituting the expression for stress into the strain formula:
Strain=σY=F/AY=FAY
Therefore, the compressive strain on the plank in the direction of the force is given by:
Compressive Strain=FAY
The compressive strain on the plank in the direction of the force is FAY.
Topper's Solved these Questions
Similar Questions
A rod of length L kept on a smooth horizontal surface is pulled along its length by a force F. The area of cross-section is A and Young's modulus is Y. The extension in the rod is

View SolutionA plank of mass m1 with a uniform sphere of mass m2 placed on it rests on a smooth horizontal plane. A constant horizontal force F is applied to the plank. With what acceleration will the plank and the centre of the sphere move provided there is no sliding between the plank and the sphere?
View Solution
Knowledge Check
A uniform elastic plank moves due to a constant force F0 applied at one end whose area is S. The Young's modulus of the plank is Y. The strain produced in the direction of force is
AFo2SYBFoSYC2F0SYD√2F0SYA uniform plank is resting over a smooth horizontal floor and is pulled by applying a horizontal force at its one end. Which of the following statements are not correct?
AStress developed in plank material is maximum at the end at which force is applied and decrease linearly to zero at the other end.BA uniform tensile stress is developed in the plank material.CSince plank is pulled at one end only, plank starts to accelerate along direction of the force. Hence, no stress developed in the plank material.Dnone of theseConsider the system shown. A solide sphere (of mass m and radius R) is placed over a plak (of mass 2m and placed over a smooth horizontal surface.) A horizontal force F is applied at the top of the sphere, as shown. Consider that the friction between the sphere and plank is sufficient enough for no slipping of the sphere over then plank.
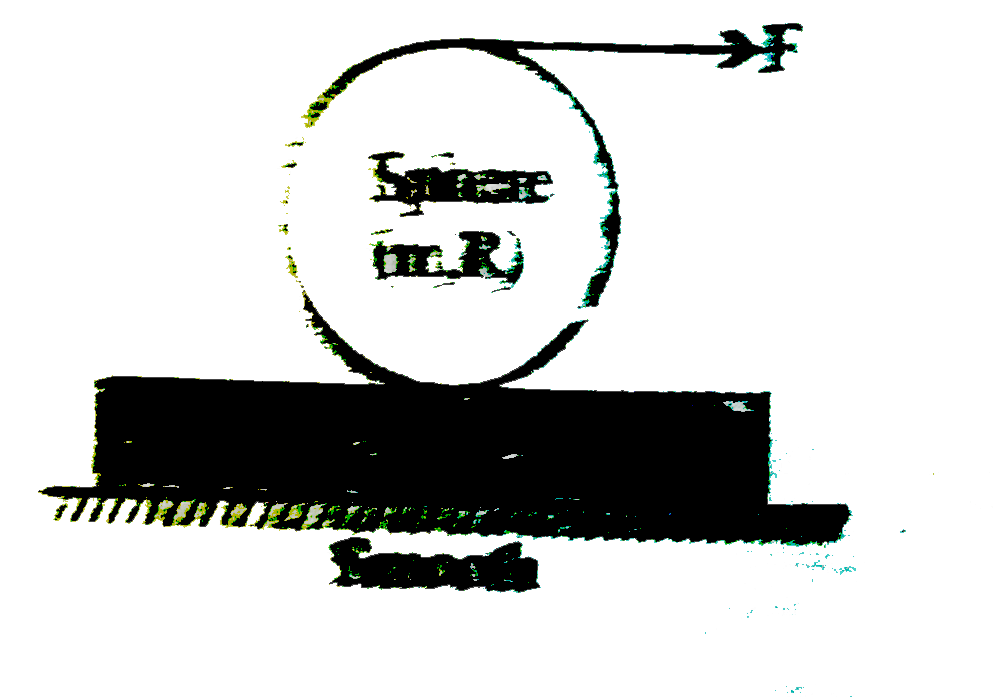
Acceleration of the plank isA3F16mB11F8mC9F4mDZero
A plank of mass M is placed on a rough horizontal surface and a contant horizontal force F is applied on it . A man of mass m runs on the plank find the range of acceleration of the man that the plank does not move on the surface is μ .Assume that the does not slip on the plank

View SolutionA sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View SolutionA plank of mass m1 with a sphere of mass m2 on it rests on a smooth horizontal surface. A canstant horizontal force F is applied to the plank. With what sliding between the plank and the sphere?
[hint: Rel vel. Of the lowest point v−(2)+εR−v1=0 for no sliding)
View SolutionThere is a uniform elastic plank moveing over a smooth horizontal plane due to a constant force F0 distributed uniformly over the front end of the plank whose area is A. If Young's modulus of the material is Y, then the compressive strain of the plank along the direction of the force is F0KAY. What is K = ?
View SolutionA block of mass'm' it kept over the smooth surface of the plank of mass M. the plank of length l is kept over the smooth horizontal surface. A constant horizontal force F is applied onto the plank as shown in figure. Calculate the time after which the block falls off the plank?
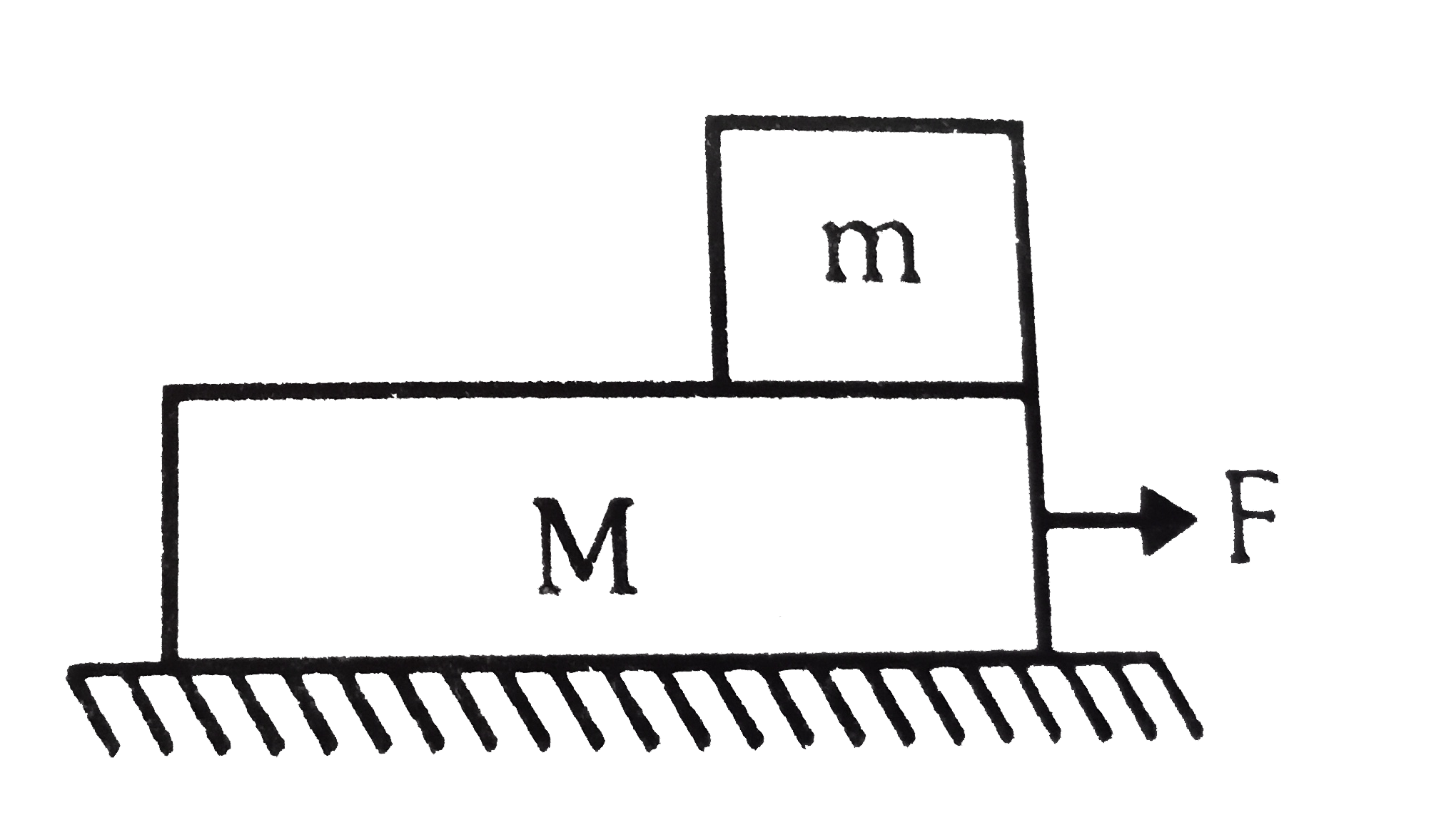
View Solution
