A uniform wire of radius r and length L hanging vertically and fixed at its upper ends, is attached to a weight at its lower end. If Young's modulus for the material of the wire is Y, the extension is
inversely proportional to Y.
inversely proportional to r2.
directly proportional to L.
inversely proportional to r.
The correct Answer is:A, B, C
To solve the problem of finding the extension (ΔL) of a uniform wire hanging vertically with a weight at its lower end, we can follow these steps:
Step 1: Understand the relationship defined by Young's Modulus
Young's modulus (Y) is defined as the ratio of stress to strain. Mathematically, it can be expressed as:
Y=StressStrain
Step 2: Define Stress and Strain
- Stress is defined as the force (F) applied per unit area (A):
Stress=FA
- Strain is defined as the change in length (ΔL) divided by the original length (L):
Strain=ΔLL
Step 3: Substitute definitions into Young's Modulus
Substituting the definitions of stress and strain into the equation for Young's modulus, we get:
Y=F/AΔL/L
Step 4: Rearrange the equation
Rearranging the equation gives:
Y=F⋅LA⋅ΔL
From this, we can solve for ΔL:
ΔL=F⋅LA⋅Y
Step 5: Calculate the Area of the wire
The area (A) of the wire can be calculated using the formula for the area of a circle:
A=πr2
where r is the radius of the wire.
Step 6: Substitute the area back into the ΔL equation
Substituting the area back into the equation for ΔL gives:
ΔL=F⋅Lπr2⋅Y
Step 7: Analyze the relationship
From the final equation, we can see how ΔL is related to other variables:
- ΔL is directly proportional to the force (F) applied.
- ΔL is directly proportional to the original length (L) of the wire.
- ΔL is inversely proportional to the cross-sectional area (πr²) and Young's modulus (Y).
Final Expression
Thus, the expression for the extension of the wire is:
ΔL=F⋅Lπr2⋅Y
Young's modulus (Y) is defined as the ratio of stress to strain. Mathematically, it can be expressed as:
Y=StressStrain
- Stress is defined as the force (F) applied per unit area (A):
Stress=FA
- Strain is defined as the change in length (ΔL) divided by the original length (L):
Strain=ΔLL
Substituting the definitions of stress and strain into the equation for Young's modulus, we get:
Y=F/AΔL/L
Rearranging the equation gives:
Y=F⋅LA⋅ΔL
From this, we can solve for ΔL:
ΔL=F⋅LA⋅Y
The area (A) of the wire can be calculated using the formula for the area of a circle:
A=πr2
where r is the radius of the wire.
Substituting the area back into the equation for ΔL gives:
ΔL=F⋅Lπr2⋅Y
From the final equation, we can see how ΔL is related to other variables:
- ΔL is directly proportional to the force (F) applied.
- ΔL is directly proportional to the original length (L) of the wire.
- ΔL is inversely proportional to the cross-sectional area (πr²) and Young's modulus (Y).
Thus, the expression for the extension of the wire is:
ΔL=F⋅Lπr2⋅Y
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (Linked Comprehension)6videos
ELASTICITY
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (Matrix - Match)5videos
ELASTICITY
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS21videos
ELASTICITY
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )3videos
ELECTRIC CHARGES AND FIELDS
Similar Questions
A load of 3kg produces an extension of 1.5mm in a wire of length 3m and diameter 2mm. Young's modulus of the material of the wire is
View SolutionA uniform steel wire of length 3.14 m and diameter 2×10−3 m is fixed at its upper end. When a mass of 10 kg is attached to its lower end, the extension of the wire is 1×10−3 m. The Young's modulus of elasticity is,
View Solution
Knowledge Check
- Question 1 - Select One
A wire suspended vertically from one of its ends is stretched by attaching a weight of 20 N to its lower end. If its length changes by 1% and if the Young's modulus of the material of the wire is 2×1011N/m2, then the area of cross section of the wire is
A1mm2B10−1mm2C10−2mm2D10−3mm2 - Question 2 - Select One
If the length of a wire is doubled, then its Young's modulus
Abecome doubleBbecome halfCincreases but becomes not exactly doubleDremains unchanged - Question 3 - Select One or More
A metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
AThe loss in gravitational potential energy of mass M is Mgl.BThe elastic potential energy stored in the wire is MglCThe elastic potential energy stored in the wire is 12MglDHeat produced is loss of mechanical energy of system is 12Mgl
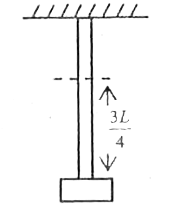
One end of uniform wire of length L and of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. If s is the area of cross section of the wire, the stress in the wire at a height (3L/4) from its lower end is
View SolutionOne end of a uniform wire of length L and weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. If S is the area of cross section of the wire, the stress in the wire at a height (3L/4) from its lower end is
 View Solution
View SolutionOne end of a uniform wire of length L and of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. If S is the area of cross-section of the wire, the stress in the wire at a height 3 L/4 from its lower end is
View SolutionOne end of uniform wire of length L and of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. If s is the area of cross section of the wire, the stress in the wire at a height (3L/4) from its lower end is
View SolutionOne end of uniform wire of length Land of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. If S is the area if cross-section of the wire, the stress in the wire at a height (3L/4) from its lower end is
View Solution

