A vertical loop of radius R and mass M rolls without slipping on horizontal circular tracks of radius R with velocity V as shown the total kinetic energy of the loop is
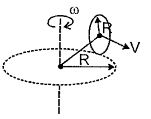
32MV2
1/2MV^2
MV2
54MV2
The correct Answer is:D
Topper's Solved these Questions
Similar Questions
A spherical ball of radius r and mass m is rolling without slipping on a horizontal table. Calculate the percentage of KE, which is translational.
View SolutionA loop and a disc have same mass and roll without slipping with the same linear velocity v. If the total kinetic energy of the loop is 8J, the kinetic energy of the disc must be.
View Solution
Knowledge Check
A solid sphere of radius 'r' is rolling with velocity V on a smooth plane. The total kinetic energy of sphere is
A710mv2B34mv2C12mv2D14mv2A loop and a disc have same mass and roll without slipping with the same linear velocity v. If the total kinetic energy of the loop is 8J, the kinetic energy of the disc must be.
A8 JB16 JC6 JD4 JA solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.
A solid sphere of mass m and radius R rolls without slipping on the horizontal surface such that its velocity of center of mass is v0. Find its translational kinetic energy, rotational kinetic energy, total kinetic energy, gravitational potential energy taking surface as reference level and mechanical energy.
View SolutionA uniform thin spherical shell of mass m and radius R rolls down without slipping over an inclined plane of angle θ with the horizontal. The ratio of rotational kinetic energy to the total kinetic energy of the shell will be
View SolutionA rigid body of radius R and radius of gyration k rolls without slipping along a horizontal plane. Then, the ratio of its rotational energy to total energy is
View SolutionA symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
View SolutionA solid sphere is rolling without slipping on a horizontal plane. The ratio of its rotational kinetic energy and translational kinetic energy is
View Solution
