A vertical pipe open at both ends is partially submerged in water . A tuning fork is unknown frequency is placed near the top of the pipe and made to vibrate . The pipe can be moved up and down and thus length of air column the pipe can be adjusted. For definite lengths of air column in the pipe, standing waves will be set up as a result of superposition of sound waves travelling in opposite directions. Smallest value of length of air column , for which sound intensity is maximum is 10cm[ take speed of sound , v=344m/s].
Answer the following questions.
Length of air column for third resonance will be
30cm
45cm
20cm
50cm
The correct Answer is:A
To find the length of the air column for the third resonance in a vertical pipe open at both ends, we can follow these steps:
1. Understand the Resonance in a Pipe Open at Both Ends:
- In a pipe open at both ends, the fundamental frequency (first harmonic) occurs when there is one antinode at each open end. The length of the pipe (L) corresponds to half the wavelength (λ) of the sound wave.
- For the first harmonic (fundamental frequency), the relationship is given by:
L1=λ2
2. Identify the Relationship for Higher Harmonics:
- For the second harmonic, the length of the pipe corresponds to one full wavelength:
L2=λ
- For the third harmonic, the length of the pipe corresponds to one and a half wavelengths:
L3=3λ2
3. Given Information:
- The smallest length of the air column for maximum sound intensity (first resonance) is given as L1=10cm.
- From the first harmonic formula, we can express the wavelength:
L1=λ2⟹λ=2L1=2×10cm=20cm
4. Calculate the Length for Third Resonance:
- Using the wavelength calculated, we can now find the length of the air column for the third resonance:
L3=3λ2=3×20cm2=60cm2=30cm
5. Conclusion:
- The length of the air column for the third resonance is 30cm.
Final Answer:
The length of the air column for the third resonance is 30 cm.
- In a pipe open at both ends, the fundamental frequency (first harmonic) occurs when there is one antinode at each open end. The length of the pipe (L) corresponds to half the wavelength (λ) of the sound wave.
- For the first harmonic (fundamental frequency), the relationship is given by:
L1=λ2
- For the second harmonic, the length of the pipe corresponds to one full wavelength:
L2=λ
- For the third harmonic, the length of the pipe corresponds to one and a half wavelengths:
L3=3λ2
- The smallest length of the air column for maximum sound intensity (first resonance) is given as L1=10cm.
- From the first harmonic formula, we can express the wavelength:
L1=λ2⟹λ=2L1=2×10cm=20cm
- Using the wavelength calculated, we can now find the length of the air column for the third resonance:
L3=3λ2=3×20cm2=60cm2=30cm
- The length of the air column for the third resonance is 30cm.
The length of the air column for the third resonance is 30 cm.
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Integer
9 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Single Correct Answer Type
56 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Assertion - Reasoning
6 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
Similar Questions
A vertical pipe open at both ends is partially submerged in water . A tuning fork is unknown frequency is placed near the top of the pipe and made to vibrate . The pipe can be moved up and down and thus length of air column the pipe can be adjusted. For definite lengths of air column in the pipe, standing waves will be set up as a result of superposition of sound waves travelling in opposite directions. Smallest value of length of air column , for which sound intensity is maximum is 10cm[ take speed of sound , v=344m/s].
Answer the following questions.
Frequency of the tuning fork is
View SolutionA vertical pipe open at both ends is partially submerged in water . A tuning fork is unknown frequency is placed near the top of the pipe and made to vibrate . The pipe can be moved up and down and thus length of air column the pipe can be adjusted. For definite lengths of air column in the pipe, standing waves will be set up as a result of superposition of sound waves traveling in opposite directions. Smallest value of length of air column , for which sound intensity is maximum is 10cm [ take speed of sound , v=344m/s].
Answer the following questions.
The air column here is closed at one end because the surface of water acts as a wall. Which of the following is correct ?
View Solution
Knowledge Check
velocity of sound in air is 320 m/s. A pipe closed at one end has a length of 1 m. Neglecting end corrections, the air column in the pipe can resonates for sound of frequency
A80 HzB240 HzC320 HzD400 Hz
A simple apparatus for demonstrating resonance in an air column is depicted in Fig. 7.59.A vertical pipe open at both ends is partially submerge in water , and a tuning fork vibration at an unknown frequency is placed near the top of the pipe . The length L of the air column can be adjusted by moving the pipe vertically . The sound waves generated by the fork are reinforced of the pipe . For a certain pipe , the smallest value of L for which a peak occurs in the intensity is 9.00cm.
a. What is the frequency of the tuning fork ?
b. What are the values of L for the next two resonance conditions?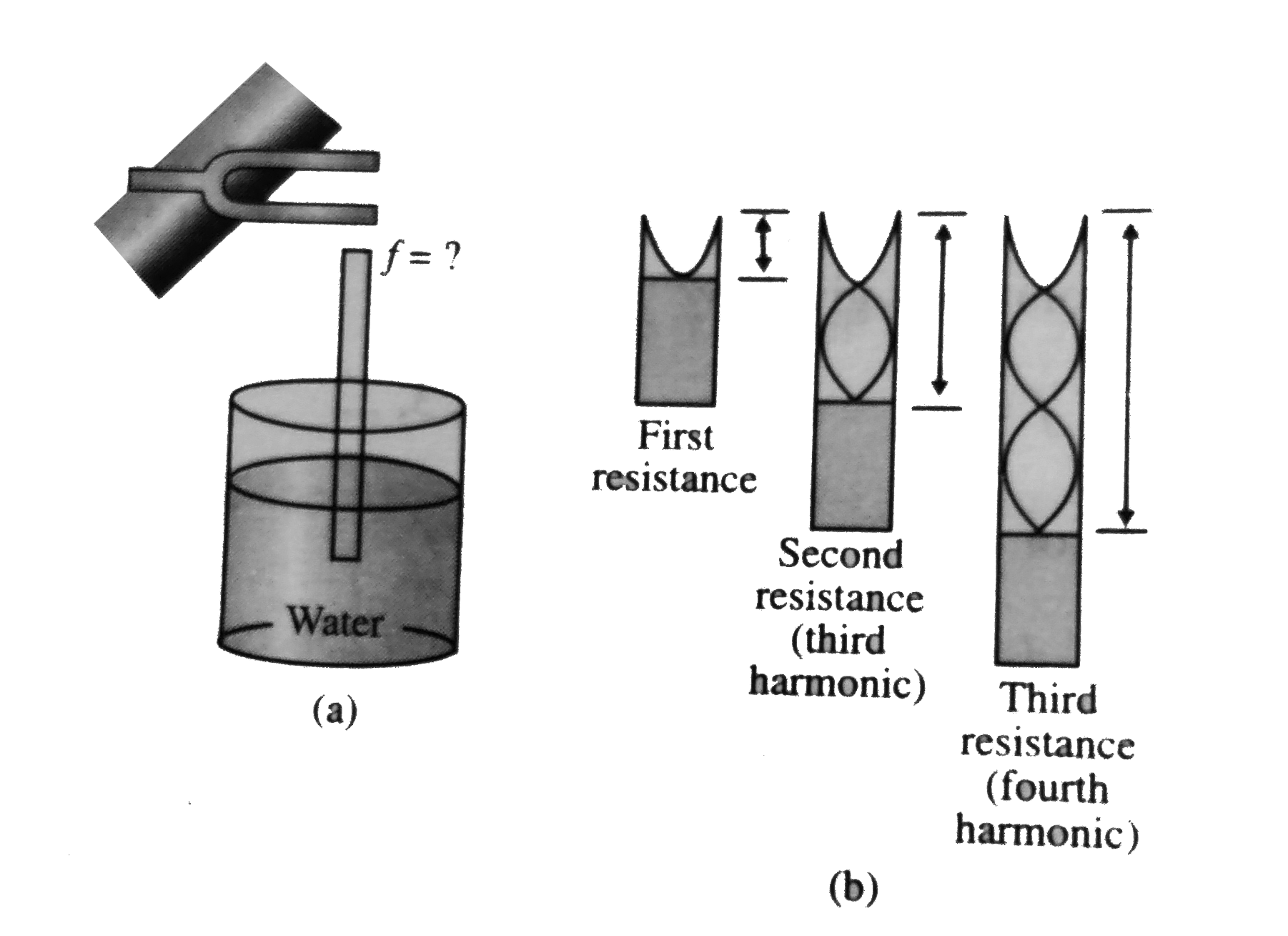
View SolutionA tuning fork vibrats with frequnecy 256Hz and gives one beat per seond with the third normal mode of vibration of an open pipe. What is the length of the pipe (Speed of sound in air is 340ms−1)
View SolutionAn air column in pipe, which is closed at one end, will be in resonance with a vibrating tuning fork of frequency 264Hz if the length of the column in cm is :
View SolutionThe air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440 Hz. The speed of sound in air is 330 m/s. find the length of air column.
View SolutionIn open organ pipe, first overtone produced is of such frequency that length of the pipe is equal to
View SolutionAn organ pipe open at one end is vibrating in first overtone and is in resonance with another pipe open at both ends and vibrating in third harmonic. The ratio of length of two pipes is–
View SolutionA sufficiently long closed organ pipe has a small hole at its bottom. Initially the pipe is empty. What poured into the pipe at a constant rate. The fundamental frequency of the air column in the pite
View Solution
