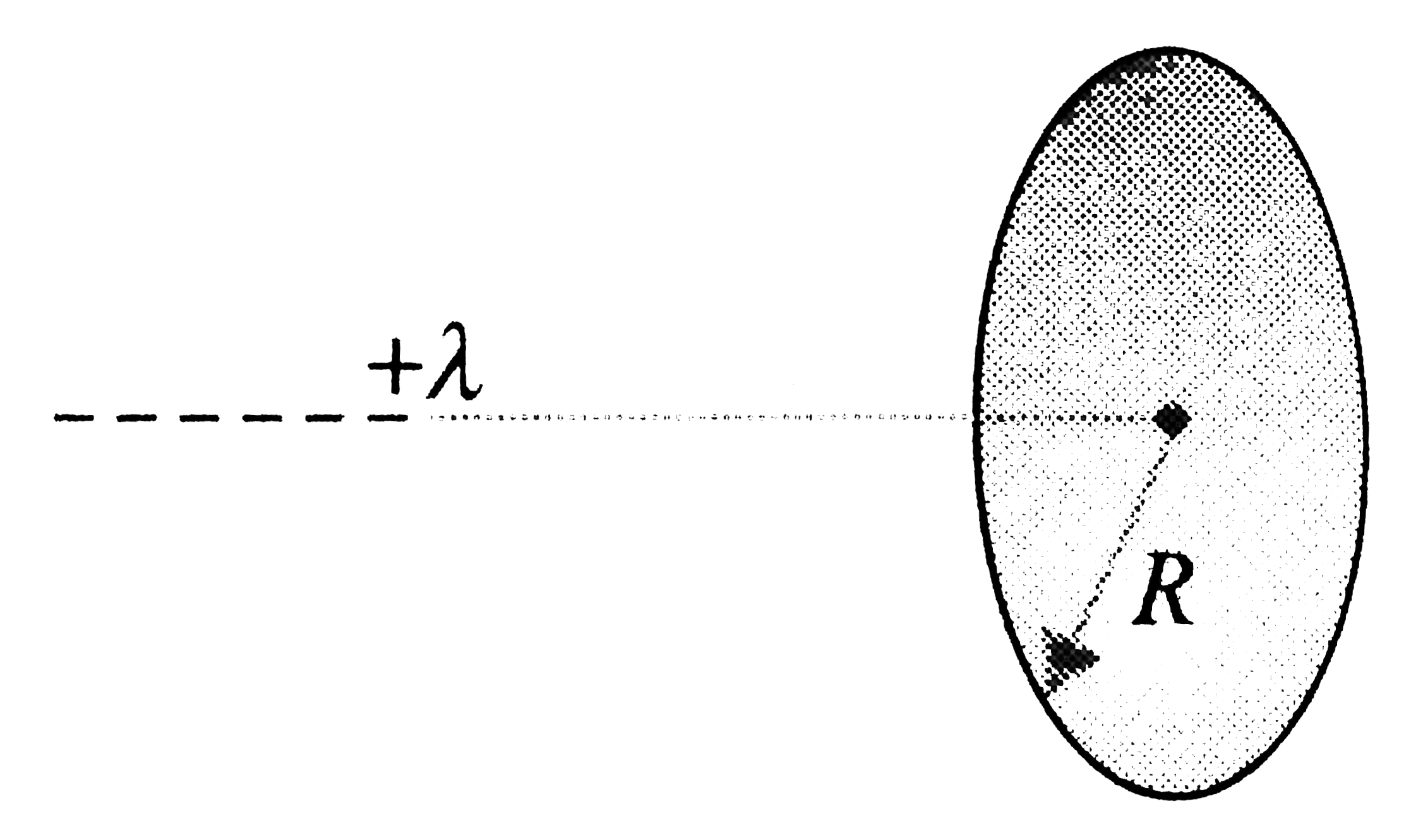
A very long uniformly thread oriented along the axis of a a circle of raius R rests on its centre with one of the ends. The charge of the thread per unit length is equla to λ. Find the flux of the vector E across the circle area.
From the solution of 3.14 field strength at a perpendicular distance r<R from its left end
→E(r)=λ4πε0r(−→i)+λ4πε0r(ˆer)
Here ˆer is a unit vector along radial direction.
Let us consider an elemental surface, dS=dydz=sz(rdθ) a figure. Thus fluix fo →E(r) over the element −→dS is given by
dΦ=→E.−→dS=[λ4πε0r(−→−i)+λ4πε0r(ˆer)].dr(rdθ)→i
=−λ4πε0drdθ(as →er⊥→i)
The sought flux, φ=−λ45πε0∫R0dr∫2π0dθ=−λR2ε0,
If we have taken →ds↑⏐
⏐⏐↑⏐
⏐⏐(−→i), then φ were λR2ε0
Hence |φ|=λR2ε0

Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Conductors And Dielectrics In An Electric Field
47 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Capacitance - Energy Of An Electric Field
46 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
A very long uniformly charge thred oriented along the axis of a circle of radius R=1m rests on its centre with one of the ends. The charge per unit length on the thread is λ=16ε0. Find the flux of the vector E through the circle area in (Vm).
View SolutionA very long uniformly charged wire oriented along the axis of a circular ring of radius R rests on its centre with one of the ends (as shown in figure). The linear charge density on the wire is λ. Evalute the flux of the vector →E across the circle area.
View Solution
Knowledge Check
A wire is bent to form a semi circle such that the radius of the semicircle is R. The charge per unit length is λ. The total electric field at the centre will be
A2λ/πε0RBλ/2πε0RC2λ/πε0R2Dλ/πε20RA very long uniformly charged thin wire whose one end is at point (0,a) extends along positive y-axis . The charge per unit length of wire is λ . The electric field at origin is
Aλ4πε0a(−ˆj)Bλ2πε0a(ˆj)Cλ4πε0a(−ˆi−ˆj)Dλ2πε0a(ˆi)A semicircular are of radius a is charged uniformly and the charge per unit length is λ. The electric field at its centre is
Aλ2πε0a2Bλ22πε0aCλ4ε0aDλ2πε0a
A system consits fo a thin charged wire ring of radius R and a very long uniformly charged thread oriented along the axis of the ring, with one of its ends coinciding with the centre of the ring. The total charge of the ring, with one of the ring so equal to q. The charge of the thread (per unit length) is equal to λ. Find the interaction froce between the ring and the thread.
View SolutionA very long straight solenoid carries a current i. The cross-sectional area of the solenoid is equal to S, the number of turns per unit length is equal to n. Find the flux of the vector B through the end plane of the solenoid.
View SolutionA system consists of a thin ring of radius R and a very long uniform wire oriented along axis of the ring with one of its ends coinciding with the centre of the ring. If mass of ring be M and mass of wire be λ per unit length, calculate interaction force between the ring and the wire.
View SolutionTwo point charges q and −q are separated by the distance 2l. Find the flux of the electric field strength vector across a circle of radius R.

View SolutionA thin infinitely long thread carrying a charge λ per unit length is oriented parallel to the infinite conducting plane. The distance between the thread and the plane is equal to l. Find :
(a) the moducles of the vector of the force acting on a unit length of the thread ,
(b) the disribution of surface chagre density σ(x) over the plane, where x is the distance form the plane perpendicular to the conducting surface and passing through the thread.
View Solution
