A vessel is partly filled with a liquid. Coefficients of cubical expansion of material of the vessel and liquid are γv unoccupied by the liquid will necessarily
(a)remain unchanged if γv=γL
(b)increase if γv=γL
(c)decrease if γv=γL
(d)none of the above
The correct Answer is:B
To solve the problem, we need to analyze the situation of a vessel that is partially filled with a liquid, taking into account the coefficients of cubical expansion of both the vessel and the liquid.
1. Understanding the Coefficients of Cubical Expansion:
- The coefficient of cubical expansion (γ) measures how much a substance expands per degree change in temperature. For the vessel, we denote it as γ_v (for the vessel) and for the liquid as γ_l (for the liquid).
2. Initial Volume of the Vessel and Liquid:
- Let the initial volume of the liquid be V_l and the initial volume of the vessel be V_v. The volume of the vessel that is unoccupied by the liquid is V_u = V_v - V_l.
3. Change in Volume Due to Temperature Change:
- When the temperature changes by ΔT, the change in volume of the liquid (ΔV_l) can be expressed as:
ΔVl=Vl⋅γl⋅ΔT
- Similarly, the change in volume of the vessel (ΔV_v) is given by:
ΔVv=Vv⋅γv⋅ΔT
4. Volume Relationships:
- The volume of the liquid expands as it heats up, and the volume of the vessel also expands. The total volume of the vessel remains constant, which means the volume that is unoccupied will change based on the expansions of both the liquid and the vessel.
5. Setting Up the Equation:
- For the volume that remains unoccupied (V_u), we can write:
Vu+ΔVl=Vv+ΔVv
- Rearranging gives us:
Vu=Vv+ΔVv−ΔVl
6. Equating Changes:
- Since the unoccupied volume must remain constant, we can set the changes equal:
ΔVl=ΔVv
- This leads us to conclude that:
Vl⋅γl⋅ΔT=Vv⋅γv⋅ΔT
- Simplifying this, we find:
γl=γv
7. Conclusion:
- Therefore, the coefficient of cubical expansion of the liquid must equal that of the vessel for the unoccupied volume to remain unchanged. Thus, the correct option is A.
- The coefficient of cubical expansion (γ) measures how much a substance expands per degree change in temperature. For the vessel, we denote it as γ_v (for the vessel) and for the liquid as γ_l (for the liquid).
- Let the initial volume of the liquid be V_l and the initial volume of the vessel be V_v. The volume of the vessel that is unoccupied by the liquid is V_u = V_v - V_l.
- When the temperature changes by ΔT, the change in volume of the liquid (ΔV_l) can be expressed as:
ΔVl=Vl⋅γl⋅ΔT
- Similarly, the change in volume of the vessel (ΔV_v) is given by:
ΔVv=Vv⋅γv⋅ΔT
- The volume of the liquid expands as it heats up, and the volume of the vessel also expands. The total volume of the vessel remains constant, which means the volume that is unoccupied will change based on the expansions of both the liquid and the vessel.
- For the volume that remains unoccupied (V_u), we can write:
Vu+ΔVl=Vv+ΔVv
- Rearranging gives us:
Vu=Vv+ΔVv−ΔVl
- Since the unoccupied volume must remain constant, we can set the changes equal:
ΔVl=ΔVv
- This leads us to conclude that:
Vl⋅γl⋅ΔT=Vv⋅γv⋅ΔT
- Simplifying this, we find:
γl=γv
- Therefore, the coefficient of cubical expansion of the liquid must equal that of the vessel for the unoccupied volume to remain unchanged. Thus, the correct option is A.
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Multiple Correct
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Comprehension
30 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Subjective
25 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A vessel is partly filled with a liquid. Coefficients of cubical expansion of material of the vessel and liquid are γv and γL , then the volume unoccupied by the liquid will necessarily
View SolutionDifferent liquids have same value of cubical expansion.
View SolutionA vessel is partly filled with liquid. When the vessel is cooled to a lower temperature, the space in the vessel unoccupied by the liquid remains constant. Then the volume of the liquid (VL) volume of the vessel (VV) the coefficient of cubical expansion of the material of the vessel (γv) and of the liquid (γL) are related as
View SolutionA vessel is partly filled with liquid. When the vessel is cooled to a lower temperature, the space in the vessel unoccupied by the liquid remains constant. Then the volume of the liquid (VL) volume of the vessel (VV) the coefficient of cubical expansion of the material of the vessel (γv) and of the solid (γL) are related as
View SolutionA fluid having a thermal coefficient of volume expansion γ is filled in a cylindrical vessel up to a height h0. The coefficient of linear thermal expansion of the material of the vessel is α . If the fluid is heated with the vessel , then find the level of liquid when temperature increases by Δθ.
View SolutionA vessel is quarter filled with a liquid of refractive index μ. The remaining parts of the vessel is filled with an immiscible liquid of refractive index 3μ/2. The apparent depth of the vessel is 50% of the actual depth. The value of μ is
View SolutionAmong the following , the liquid having negative coefficient of cubical expansion between 273K and 277K is
View SolutionA solid cube is first floating in a liquid. The coefficient of linear expansion of cube is α and the coefficient of volume expansion of liquid is γ. On increasing the temperature of (liquid + cube) system, the cube will sink if

View SolutionA cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
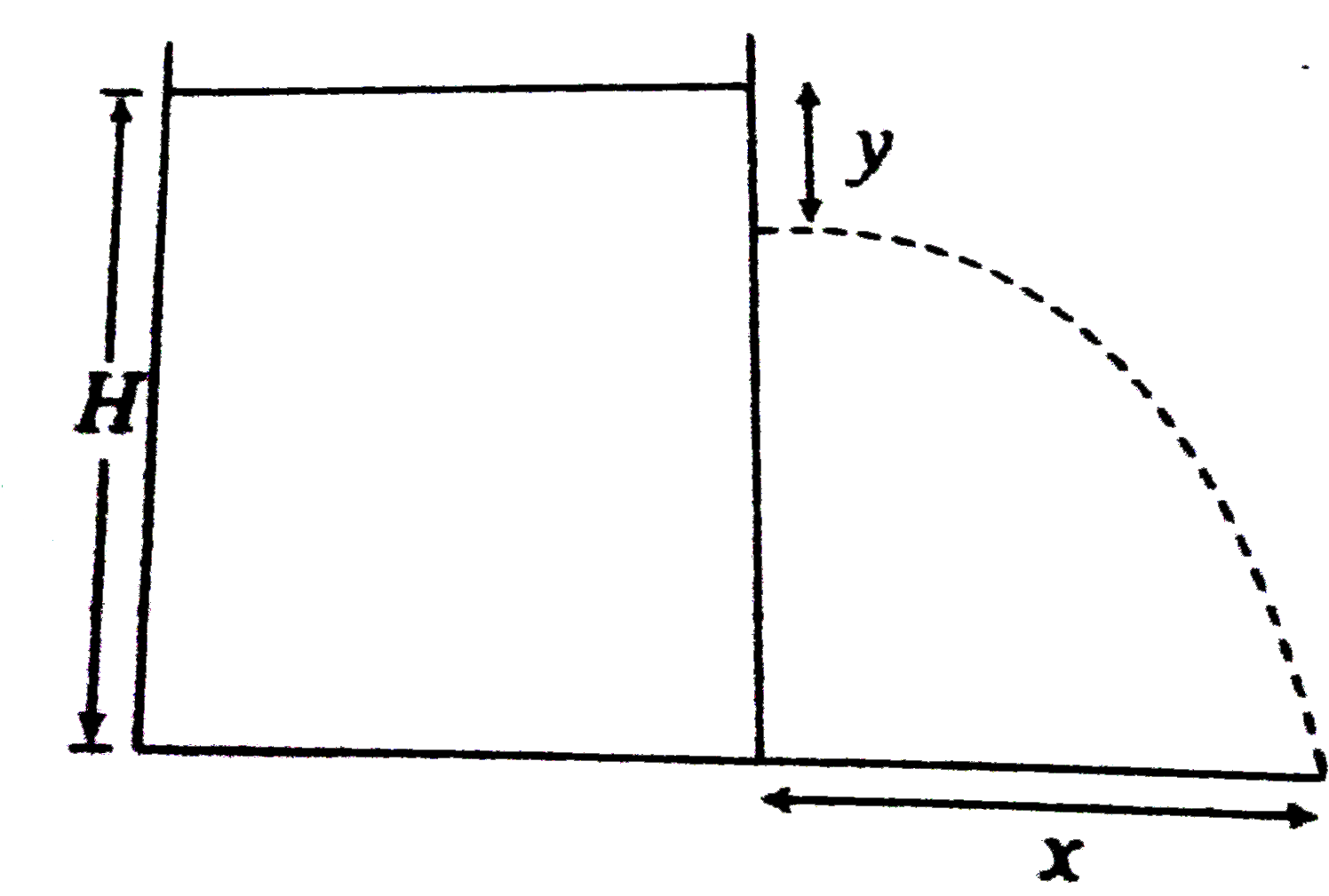
View SolutionA glass vessel of volume 100cm3 is filled with mercury and is heated from 25∘C→75∘C. What volume of mercury will overflow? Coefficient of linear expansion of glass =1.8×10−6/'∘C and coefficient of volume expansion of mercury is 1.8×10−4/'∘C.
View Solution
