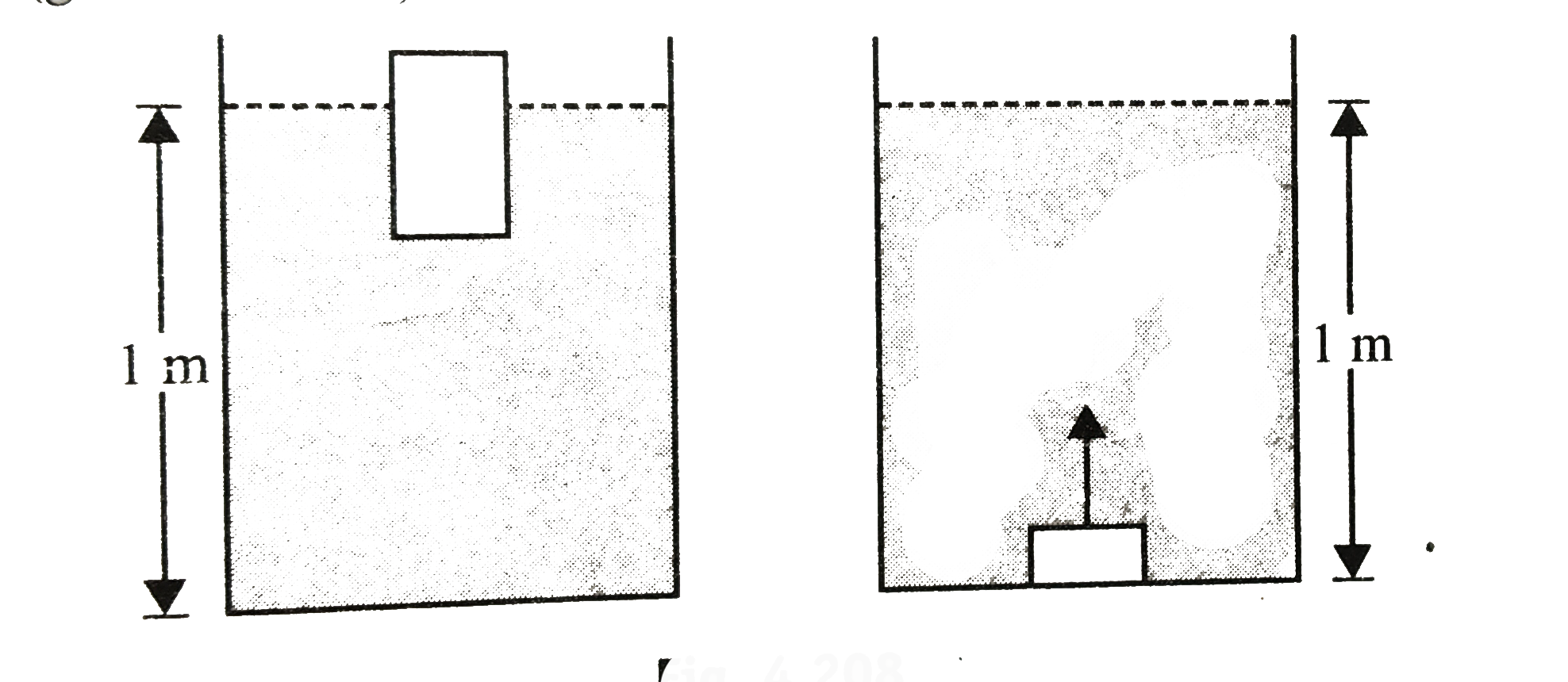
A wooden block in the form of a uniform cylinder floats with one-third length above the water surface. A small chip of this block is held at rest at the bottom of a container containing water to a height of 1m and is then released. The time in which it will rise to the surface of water is =10m/s2)
0.84s
0.63s
0.5s
1s
The correct Answer is:B
Since two thirds of the wooden block is immersed in water, its densityy =2ρ/3 where ρ is the density of water.
Let the volume of the chip be V. Then its weight is (2/3)Vρg.
The upthrust acting on it inside the water is Vρg. Hence, the unbalanced upward forces is Vρg(1−2/3)=Vρg3.
Hence its aceleratioin a in the upward direction is
a=Vρg/3(2/3)Vρ=g2
If t is the required time time 1=012at2=12g2t2
Hence t2=4g=410=0.4=0.63s
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct15videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning8videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Subjective25videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
Similar Questions
A rubber ball of mass 100 g and radius 5 cm is submerged in water to a depth of 1 m and released. To what height will the ball jump up above the surface of water ? (Take g=10ms−2)
View SolutionA container of filled with water to a height of 10 m.
The pressure exerted by the water at the bottom of the container is _________ .View Solution
Knowledge Check
- Question 1 - Select One
A large wooden piece in the form of a cylinder floats on water with two-thirds of its length immersed. When a man stands on its upper surface, a further one-sixth of its length is immersed. The ratio between the masses of the man and the wooden piece is
A1:2B1:3C1:4D1:5 - Question 2 - Select One
A small wooden ball of density ρ is immersed in water of density σ to depth h and then released. The height H above the surface of water up to which the ball will jump out of water is
AσhρB(σρ−1)hChDzero - Question 3 - Select One
A wooden block is floating on water kept in a beaker. 40% of the block is above the water surface. Now the beaker is kept inside a lift that starts going upward with acceleration equal to g/2. The block will then
AsinkBfloat with 10% above the water surfaceCfloat with 40% above the water surfaceDfloat with 70% above the water surface
Why does a block of wood held under water rise to the surface when released ?
View SolutionA capillary tube of radius 0.5 mm is dipped in a vessel containing water. Calculate the height to which water will rise in the capillary tube. Take, surface tension of water =7.4×10−3 N/m.
View SolutionA tank containing water has an orifice 2 m below the surface of water. If there is no wastage of energy. Find the speed of discharge.
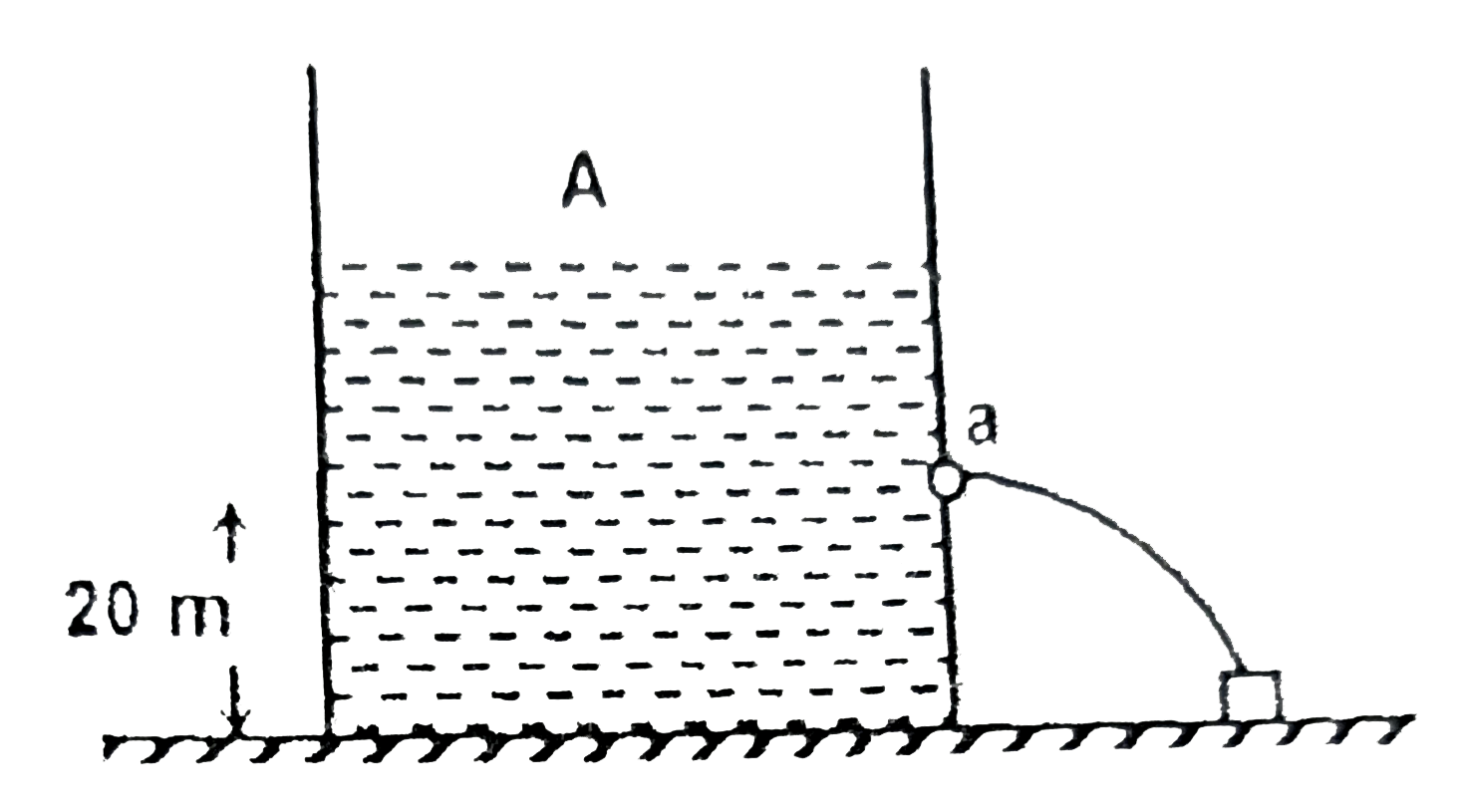
View SolutionWater is filled in a uniform container of area of cross section A. A hole of cross section area a (<<A) is made in the container at a height of 20m above the base. Water streams out and hits a small block on surface at some distance from container. Block is moved on surface in such a way that stream always hits the block. The initial velocity of the block (in m/s) is (Given aA=120). (Take g=10ms−2)
 View Solution
View SolutionA large cylindrical vessel contains water to a height of 10 m. If is found that the thrust acting on the curved surface is equal to that at the bottom. If atmospheric pressure can support a water column of 10 m. the radius of the vessel is
View Solution

