After falling from rest through a height h, a body of mass m begins to raise a body of mass M(M>m) connected to it through a pulley.
Find the fraction of kinetic energy lost when the body of mass M is jerked into motion
MM+m
MM−m
2MM+m
M2(M+m)
The correct Answer is:A
To solve the problem, we need to analyze the motion of two bodies connected by a pulley system. Here’s a step-by-step breakdown of the solution:
Step 1: Determine the velocity of mass m just before the string becomes taut
When the body of mass m falls from rest through a height h, we can use the equation of motion to find its velocity just before the string becomes taut.
Using the equation:
\(
v = \sqrt{2gh}
\)
where \( g \) is the acceleration due to gravity.
Step 2: Apply conservation of momentum when the string becomes taut
When the string becomes taut, the body of mass M is jerked into motion. At this point, we can apply the principle of conservation of momentum. Let \( v' \) be the common speed of both masses after the string is taut.
Before the string becomes taut, the momentum is:
\(
p_{\text{before}} = mv
\)
After the string becomes taut, the momentum is:
\(
p_{\text{after}} = (M + m)v'
\)
Setting these equal gives us:
\(
mv = (M + m)v'
\)
From this, we can solve for \( v' \):
\(
v' = \frac{mv}{M + m}
\)
Step 3: Calculate the acceleration of the system
The acceleration of the system can be determined by considering the forces acting on it. The net force acting on the system is:
\(
F_{\text{net}} = Mg - mg
\)
According to Newton's second law, this net force is equal to the total mass times acceleration:
\(
Mg - mg = (M + m)a
\)
Rearranging gives:
\(
a = \frac{Mg - mg}{M + m}
\)
Step 4: Find the time taken for the system to return to its original position
Let the system return to its original position at time \( t \). Using the kinematic equation:
\(
s = vt + \frac{1}{2}at^2
\)
Since the displacement \( s \) is zero when the system returns to its original position, we can set up the equation:
\(
0 = v't - \frac{1}{2}at^2
\)
Solving for \( t \) gives:
\(
t = \frac{2v'}{a}
\)
Step 5: Calculate the fractional loss of kinetic energy
The initial kinetic energy (just before the string becomes taut) is:
\(
KE_{\text{initial}} = \frac{1}{2}mv^2
\)
The final kinetic energy (after the string becomes taut) is:
\(
KE_{\text{final}} = \frac{1}{2}(M + m)v'^2
\)
The fractional loss of kinetic energy is given by:
\(
\text{Fractional loss} = \frac{KE_{\text{initial}} - KE_{\text{final}}}{KE_{\text{initial}}}
\)
Substituting the expressions for kinetic energy:
\(
\text{Fractional loss} = \frac{\frac{1}{2}mv^2 - \frac{1}{2}(M + m)v'^2}{\frac{1}{2}mv^2}
\)
This simplifies to:
\(
\text{Fractional loss} = 1 - \frac{(M + m)v'^2}{mv^2}
\)
Step 6: Substitute \( v' \) and simplify
Substituting \( v' = \frac{mv}{M + m} \) into the equation gives:
\(
\text{Fractional loss} = 1 - \frac{(M + m)\left(\frac{mv}{M + m}\right)^2}{mv^2}
\)
This simplifies to:
\(
\text{Fractional loss} = 1 - \frac{m}{M + m}
\)
Thus, the final result for the fractional loss of kinetic energy is:
\(
\text{Fractional loss} = \frac{M}{M + m}
\)
Final Answer
The fraction of kinetic energy lost when the body of mass M is jerked into motion is:
\(
\frac{M}{M + m}
\)
When the body of mass m falls from rest through a height h, we can use the equation of motion to find its velocity just before the string becomes taut.
\(
v = \sqrt{2gh}
\)
where \( g \) is the acceleration due to gravity.
When the string becomes taut, the body of mass M is jerked into motion. At this point, we can apply the principle of conservation of momentum. Let \( v' \) be the common speed of both masses after the string is taut.
\(
p_{\text{before}} = mv
\)
After the string becomes taut, the momentum is:
\(
p_{\text{after}} = (M + m)v'
\)
Setting these equal gives us:
\(
mv = (M + m)v'
\)
From this, we can solve for \( v' \):
\(
v' = \frac{mv}{M + m}
\)
The acceleration of the system can be determined by considering the forces acting on it. The net force acting on the system is:
\(
F_{\text{net}} = Mg - mg
\)
According to Newton's second law, this net force is equal to the total mass times acceleration:
\(
Mg - mg = (M + m)a
\)
Rearranging gives:
\(
a = \frac{Mg - mg}{M + m}
\)
Let the system return to its original position at time \( t \). Using the kinematic equation:
\(
s = vt + \frac{1}{2}at^2
\)
Since the displacement \( s \) is zero when the system returns to its original position, we can set up the equation:
\(
0 = v't - \frac{1}{2}at^2
\)
Solving for \( t \) gives:
\(
t = \frac{2v'}{a}
\)
The initial kinetic energy (just before the string becomes taut) is:
\(
KE_{\text{initial}} = \frac{1}{2}mv^2
\)
The final kinetic energy (after the string becomes taut) is:
\(
KE_{\text{final}} = \frac{1}{2}(M + m)v'^2
\)
The fractional loss of kinetic energy is given by:
\(
\text{Fractional loss} = \frac{KE_{\text{initial}} - KE_{\text{final}}}{KE_{\text{initial}}}
\)
\(
\text{Fractional loss} = \frac{\frac{1}{2}mv^2 - \frac{1}{2}(M + m)v'^2}{\frac{1}{2}mv^2}
\)
This simplifies to:
\(
\text{Fractional loss} = 1 - \frac{(M + m)v'^2}{mv^2}
\)
Substituting \( v' = \frac{mv}{M + m} \) into the equation gives:
\(
\text{Fractional loss} = 1 - \frac{(M + m)\left(\frac{mv}{M + m}\right)^2}{mv^2}
\)
This simplifies to:
\(
\text{Fractional loss} = 1 - \frac{m}{M + m}
\)
Thus, the final result for the fractional loss of kinetic energy is:
\(
\text{Fractional loss} = \frac{M}{M + m}
\)
The fraction of kinetic energy lost when the body of mass M is jerked into motion is:
\(
\frac{M}{M + m}
\)
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Integer16videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Fill In The Blanks2videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning2videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
Similar Questions
After falling from rest through a height h, a body of mass m begins to raise a body of mass M(M>m) connected to it through a pulley. Determnethe time it will take for the body of mass M to return to its original position
View SolutionA moving body of mass m makes a head on elastic collision with another body of mass 2m which is initially at rest. Find the fraction of kinetic energy lost by the colliding particles after collision.
View Solution
Knowledge Check
- Question 1 - Select One
A spherical ball of mass m1 collides head on with another ball of mass m2 at rest . The collision is elastic . The fraction of kinetic energy lost by m1 is :
A4m1m2(m1+m2)2Bm1m1+m2Cm2m1+m2Dm1m2(m1+m2)2 - Question 2 - Select One
Body 1 of mass M is dropped from a height of 1 m and body 2 of mass 3 M is dropped from a height of 9 m. Ratio of time taken by bodies 1 and 2 to reach the ground is
A1:1B1:3C3:1D9:1
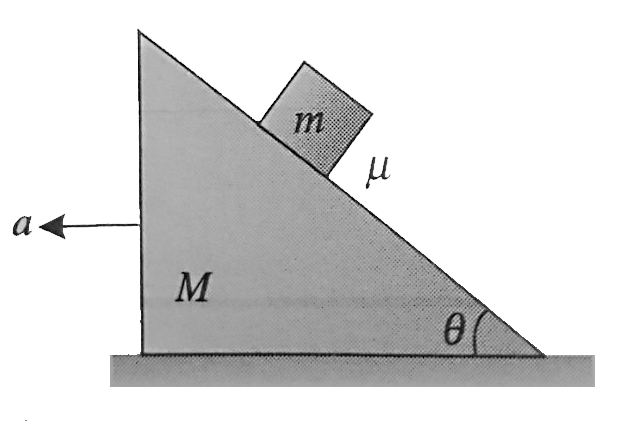
A block of mass m is at rest relative to the stationary wedge of mass M. The coefficient of friction between block and wedge is μ. The wedge is now pulled horizontally with acceleration a as shown in figure. Then the minimum magnitude of a for the friction between block and wedge to be zero is:View SolutionFind the kinetic energy of a body of mass 1 kg moving with a uniform velocity of 10 ms−1
View SolutionIf a body of mass m is raised to height 2 R from the earths surface, then the change in potential energy of the body is (R is the radius of earth)
View SolutionA body of mass 0.2 kg falls from a height of 10 m to a height of 6 m above the ground. Find the loss in potential energy taking place in the body.
[g=10ms−2]View SolutionA body of mass 5 kg is taken a height 5 m to 10 m .Find the increase in its potential energy (g=10 m s−2)
View SolutionA body of mass 4kg moving with velocity 12m/s collides with another body of mass 6kg at rest. If two bodies stick together after collision , then the loss of kinetic energy of system is
View Solution

