An aeroplane is flying in a horizontal direction with a velocityu and at a height of 2000 m. When it is vertically below a point A on the ground a food packet is released from it. The packet strikes the ground at point B. If AB = 3 km and g =10 m/s2 , then the value of u is
54 km/h
540 km/h
150 km/h
300 km/h
The correct Answer is:b
To solve the problem, we need to find the horizontal velocity u of the aeroplane when a food packet is released from a height of 2000 m and strikes the ground at a distance of 3 km horizontally. We will use the principles of projectile motion to derive the solution step-by-step.
Step 1: Identify the given values
- Height h=2000m
- Horizontal distance AB=3km=3000m
- Acceleration due to gravity g=10m/s2
Step 2: Calculate the time of flight t
The time taken for the packet to fall from the height h can be calculated using the formula for free fall:
h=12gt2
Rearranging the formula to solve for t:
t2=2hg
Substituting the values:
t2=2×200010=400010=400
Taking the square root:
t=√400=20s
Step 3: Use the range formula to find u
The horizontal distance (range) covered by the packet can be expressed as:
Range=u⋅t
Substituting the known values:
3000=u⋅20
Solving for u:
u=300020=150m/s
Step 4: Convert u to km/h (if required)
To convert from meters per second to kilometers per hour, we use the conversion factor 185:
u=150m/s×185=540km/h
Final Answer
The horizontal velocity u of the aeroplane is 150m/s or 540km/h.
---
- Height h=2000m
- Horizontal distance AB=3km=3000m
- Acceleration due to gravity g=10m/s2
The time taken for the packet to fall from the height h can be calculated using the formula for free fall:
h=12gt2
Rearranging the formula to solve for t:
t2=2hg
Substituting the values:
t2=2×200010=400010=400
Taking the square root:
t=√400=20s
The horizontal distance (range) covered by the packet can be expressed as:
Range=u⋅t
Substituting the known values:
3000=u⋅20
Solving for u:
u=300020=150m/s
To convert from meters per second to kilometers per hour, we use the conversion factor 185:
u=150m/s×185=540km/h
The horizontal velocity u of the aeroplane is 150m/s or 540km/h.
Topper's Solved these Questions
Similar Questions
An aeroplane is flying horizontally at a height of 23km with a velocity of 15 km/h. Find the rate at which it is receding from a fixed point on the ground which it passed over 2 min ago.
View SolutionA ball is projected from a point O as shown in figure. It will strike the ground after (g = 10 m/s2)
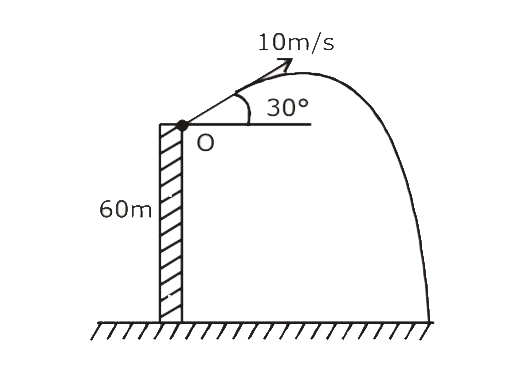
View Solution
Knowledge Check
An areoplane is flying horizontally with a velocity 720km/h at a height of 2000m. When it is vertically at a point A on the ground, a bomb is released from it. The bomb strikes the gound at point B. The distance AB is
A1kmB2kmC3kmD4kmAn aeroplane is flying in a horizontal direction with a velocity 600km/h at a height of 1960 m. When it is vertically above the point A on the ground, a body is dropped from it. The body strikes the ground at point B. Calculate the distance AB.
A3.5kmB4.5kmC3.33kmD5.5kmAn acroplane is flying horizontally with a velocity of 600 km/h and a height of 1960m. When it is vectrically above a point A on the ground a bomb is released from it. The bomb strikes the ground at point B. the distance AB is:
A1200mB0.33kmC333.3kmD3.33km
A helicopter while flying at a height of 100 m with velocity 30 m.s at an angle 30∘ with the horizontal, drops a packet. Where will the packet strike the ground ? ( g = 10 m/s2)
View SolutionA particle is thrown vertically up from the top of a building of height 20m with initial velocity u. A second particle is released from the same point 1 second later. If both particles reach the ground at the same instant ,3u=m/s. [Takeg=10m/s2]
View SolutionAn aeroplane is flying in a horizontal direction with a velocity of 900 km/h and at a height of 1960m. When it is vertically above the point A on the ground, a body is dropped from it. The body strikes the ground at point B. The distance AB will be (take g=9.8m/s2)
View SolutionAn aeroplane is flying at a height of 1960 m in horizontal direction with a velocity of 360 km/hr. When it is vertically above the point. A on the ground, it drops a bomb. The bomb strikes a point B on the ground, then the time taken by the bomb to reach the ground is-
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
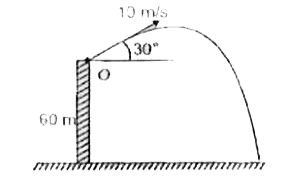
View Solution
