An amusement park ride consists of a car mvoing an a vertical circle on the end of a rigid boom of negligible mass. The combined weight of the car and riders is 6.0 kN, the circle's radius is 10m. At the top of the circle, what are the (a) magnitude and (b) direction (up or down) of the force on the car from the boom if the car's speed is v=5.0m/s? What are (c) and (d) the direction if v=12m/s?
The correct Answer is:(a) 4.5 kN(b) upward(c) 2.8kN (d) Downward
To solve the problem, we will analyze the forces acting on the car at the top of the vertical circle. The forces involved are the gravitational force (weight of the car and riders) and the force exerted by the boom (denoted as FB).
Given Data:
- Weight of the car and riders, W=6.0kN=6000N
- Radius of the circle, r=10m
- Speed at the top of the circle for part (a) and (b), v=5.0m/s
- Speed at the top of the circle for part (c) and (d), v=12.0m/s
- Acceleration due to gravity, g=9.8m/s2
1. Calculate the mass of the car:
m=Wg=6000N9.8m/s2≈612.24kg
2. Calculate the centripetal force required:
The centripetal force Fc needed to keep the car moving in a circle is given by:
Fc=mv2r
Substituting the values:
Fc=612.24×(5.0)210=612.24×2510=1530.6N
3. Apply the force balance at the top of the circle:
At the top of the circle, the net centripetal force is provided by the weight of the car minus the force from the boom:
Fc=W−FB
Rearranging gives:
FB=W−Fc=6000N−1530.6N=4469.4N
4. Convert to kN:
FB≈4.47kN
Part (b): Direction of FB when v=5.0m/s
- Since FB is positive, the direction of the force exerted by the boom on the car is upward.
---
Part (c): Magnitude of FB when v=12.0m/s
1. Calculate the centripetal force required:
Fc=mv2r=612.24×(12.0)210=612.24×14410=8805.6N
2. Apply the force balance at the top of the circle:
Fc=W−FB
Rearranging gives:
FB=W−Fc=6000N−8805.6N=−2805.6N
3. Convert to kN:
FB≈−2.81kN
Part (d): Direction of FB when v=12.0m/s
- Since FB is negative, the direction of the force exerted by the boom on the car is downward.
---
Summary of Answers:
- (a) FB≈4.47kN
- (b) Direction: Upward
- (c) FB≈−2.81kN
- (d) Direction: Downward
- Weight of the car and riders, W=6.0kN=6000N
- Radius of the circle, r=10m
- Speed at the top of the circle for part (a) and (b), v=5.0m/s
- Speed at the top of the circle for part (c) and (d), v=12.0m/s
- Acceleration due to gravity, g=9.8m/s2
m=Wg=6000N9.8m/s2≈612.24kg
The centripetal force Fc needed to keep the car moving in a circle is given by:
Fc=mv2r
Substituting the values:
Fc=612.24×(5.0)210=612.24×2510=1530.6N
At the top of the circle, the net centripetal force is provided by the weight of the car minus the force from the boom:
Fc=W−FB
Rearranging gives:
FB=W−Fc=6000N−1530.6N=4469.4N
FB≈4.47kN
Fc=mv2r=612.24×(12.0)210=612.24×14410=8805.6N
Fc=W−FB
Rearranging gives:
FB=W−Fc=6000N−8805.6N=−2805.6N
FB≈−2.81kN
- (a) FB≈4.47kN
- (b) Direction: Upward
- (c) FB≈−2.81kN
- (d) Direction: Downward
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(SINGLE CORRECT CHOICE TYPE)40videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(MORE THAN ONE CORRECT CHOICE TYPE)6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - CHECK POINTS6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)3videos
CIRCUITS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
Similar Questions
A roller coaster car has mass of 1300 kg when fully loaded with passengers. As the car passes over the top of a circular hil of radius 20m, its speed is not changing. At the top of the hill, what are the (a) magnitude FN and (b) direction (up or down) of the normal force on the car from the track if the car's speed is v=11m/s? What are (C) FN and (d) the direction if v=14m/s?
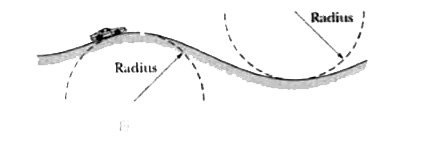
View SolutionIn Fig a car is driven at constant speed over a circular hill and then into a circular valley with the same radius. At the top of the hill, the normal force on the driver from the car seat is 0. The driver's mass is 80.0 kg. what is the magnitude of the normal force on the driver from the seat when the car passes through the bottom of the valley?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In children's park using the part ride the visitors can be made to rotate in a vertical circle. At the top of the circle, a rider has an effective weight of magnitude 1000N. If his actual weight is 500N then what is the effective at eh bottom of the circle?
A1500NB2000NC2500ND1800N - Question 2 - Select One
A car is travelling along a flyover bridge which is a part of vertical circle of radius 10m. At the highest point of it the normal reaction on the car is half of it’s weight, the speed of car is
A7m/sB10m/sC14m/sD20m/s - Question 3 - Select One
A toy car weighing 1 kg tied at the end of a tring 1 m long moves in a circle on the ground. What is the maximum possible speed of the car if the string has a breaking strength of 9 N?
A9m/sB3m/sC1.5m/sD0.75s
A police officer in hot pursuit drives her car through a circular turn of radius 300 m with a constant speed of 75.0km/h. Her mass is 55.0 kg. What are (a) the magnitude and (b) the angle (relative to vertical) of the net force the officer on the car seat?
View SolutionA driver decreases the speed of a car from 25 m/s to 10 m/s is 5 seconds. Find the acceleration of the car.
View SolutionA car is travelling at 30 km/h in a circle of radius 60 m. What is the minimum value of μs for the car to make the turn without skidding ?
View SolutionIn the above example, how is the speed of the image affected if the motor bike comes closer to the car at (a) 30 m from the car (b) 20 m from the car?
View SolutionThe speed limit of a car over a roadways bridge in circle making 2rps If radius of the circle is 1.2cm find the tension in the string at I) top of the circle (ii) bottom of the circle .
View Solution

