An electron dipole of moment →p is placed in a uniform electric field →E . Write the expression for the torque →τ experienced by the dipole. Identify two pairs of perpendicular vectors in the expression . Shown diagrammatically the orientation of the dipole in the field for which the torque is
(i) the maximum , (ii) Half the maximum value ,
(iii) Zero.Solution in Kannada
Let a dipole AB is situated in the uniform electric field →E,

The torque on dipole is ,
→τ=→p×→E, where →p is dipole moment from the line charge . Also E and unit vector n normal to curved suface are in the same direction , So θ=0∘. Contribution of curved suface of cylinder towards electric flux will be zero (null)
Two pairs of perpendicular vectors are →τand→pand→τand→E in the expression .
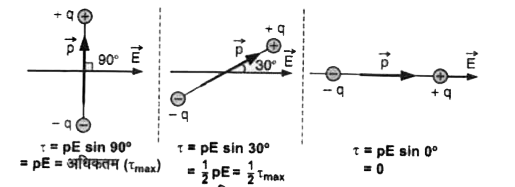
(i) The torque is the maximum , when θ=90∘ which is shown in following diagram :

(ii) The maximum value of torque =pE
The half of maximum value =pE2
So , we have pEsinθ=pE2
or sinθ=12
or θ=30∘
The arrangement is as given below or shown as below :

(iii) For zero value of torque , sinθ=0orθ=0∘ , which is shown as below :

Topper's Solved these Questions
- ELECTRIC CHARGES & FIELDSBOOK - OSWAAL PUBLICATIONCHAPTER - ELECTRIC CHARGES & FIELDSEXERCISE - (TOPIC -1)Long Answer Type Questions
2 Videos
- ELECTRIC CHARGES & FIELDSBOOK - OSWAAL PUBLICATIONCHAPTER - ELECTRIC CHARGES & FIELDSEXERCISE - (TOPIC -1)Numerical Problems
9 Videos
- ELECTRIC CHARGES & FIELDSBOOK - OSWAAL PUBLICATIONCHAPTER - ELECTRIC CHARGES & FIELDSEXERCISE - (TOPIC -2)Numerical Problems
5 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - OSWAAL PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - TOPIC-2 DE-BROGLIE RELATION (NUMERICAL PROBLEMS)
7 Videos
- ELECTROMAGNETIC INDUCTION BOOK - OSWAAL PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - TOPIC-2 (Eddy Current, Self & Mutual Inductance) (Numerical Problems)
3 Videos
Similar Questions
Obtain an expression for the electric field intenstiy at a point on the equatorial line of an electric dipole.
View SolutionPoint charges of 10nC,20nCand10nC are kept at the corners A,B C of a square ABCD of side 3m. Calculate the magnitude of the resultant electric intensity at D.
View SolutionAn atom was earlier assumed to be sphere of radius a having a positively charged point nucleus of charge +Ze at its centre . This nucleus was believed to be surrounded by a uniform desity of negative charge that made the atom neutral as a whole.
Use this theorem to find the electric field of this atom at a distance r(r>a) from the centre of the atom.
View SolutionCalculate de-Broglie wavelength associated with a rifle bullet of mass 2 gram moving with a speed of 400ms−1.
View SolutionA solenoid of length 1 m and 0.05 m diameter has 500 turns. If a current of 2A passes through the coil, calculate (i) the coefficient of self induction of the coil and (ii) the magnetic flux linked with the coil.
Data : l=1m,d=0.05m,r=0.025m,N=5000,I=2A,
(i) L=?(ii)ϕ=?
View SolutionIf an electric dipole of moment →p be placed along a uniform electric field of intensity →E, the torque acting on the dipole is
View SolutionObtain the expression for the torque vectau experienced by an electric dipole of dipole moment vecp in a uniform electric field, vecE . What will happen if the field were not uniform?
View Solutionद्विध्रुव आघूर्ण →p वाला एक वैद्युत द्विध्रुव किसी एकसमान वैद्युत क्षेत्र →E में रखा जाता है। द्विध्रुव द्वारा अनुभव किये जाने वाले बल आघूर्ण →τ के लिए समीकरण लिखें। इसमें दो लम्बवत वेक्टर जोड़े पहचानें। चिन की सहायता से क्षेत्र में द्विध्रुव की स्थिति दिखाएँ जब बल आघूर्ण : (i) अधिकतम (ii) अधिकतम का आधा और (ii) शून्य हो।
View SolutionAn electric dipole is placed in a uniform electric field vecE with its dipole moment vecp parallel to the field . Find (i) the work done in turning the dipole till its dipole moment points in the direction opposite to vecE . (ii) The orientation of the dipole for which the torque acting on it become the maximum.
View SolutionAn electric dipole of dipole moment oversetto P is placed in a uniform electric field oversetto E. Obtain the expression for the torque oversetto tau experienced by the dipole. Identify two pairs of perpendicular vectors in the expression.
View SolutionDeduce the expression for the torque acting on a dipole of dipole moment →P in the presence of uniform electric field →E.
View SolutionAn electric dipole of dipole moment oversetrightarrowP is. placed in a uniform electric field E.The maximum torque experienced by the dipole is :
View SolutionAn electric dipole of dipole moment →p is placed in a uniform electric field →E with its axis inclined to the field. Write an expression for the torquet →τ experienced by the dipole in vector form. Show diagrammatically how the dipole should be kept in the electric field so that the torque acting on it is :
maximum
View SolutionAn electric dipole of dipole moment →p is placed in a uniform electric field →E with its axis inclined to the field. Write an expression for the torquet →τ experienced by the dipole in vector form. Show diagrammatically how the dipole should be kept in the electric field so that the torque acting on it is :
zero
View SolutionIf an electric dipole of moment →p be placed along a uniform electric field of intessity →E, the torque acting on the dipole is
View Solution
