An electron experiences a quasi-elastic force kx and a ''friction force'' yx in the field of electromagnetic radiation. The Ecomponent of the field varies as E=E0cosωt. Neglecting the action of the magnetic component of the field, find,
(a) the motion equation of the electron,
(b) the mean power absored by the electron, the frequency at which that power is maximum and the expression for the maximum mean power.
The equation of the electron can (under the stated conditions) be written as
m..x+γ.x+kx=eE0cosωt
To solve this equation we shell find it convenient to use complex displacements. Consider the equation
m..z+γ.z+kz=eE0e−iωt
z=eE0e−iωt−mω2−iγω+k
(we igone transients)
Writing β=γ2m,ω20=km
we find z=eE0me−iωt/(ω20−ω2−2iβω)
Now x=1 Real part of z
=eE0m.cos(ωt+φ)√(ω20−ω2)2+4β2ω2=acos(ωt+φ)
where tanφ=2βωω2−ω20
⎛⎜
⎜⎝sinφ=−2βω√(ω20−ω20)2+4β2ω2⎞⎟
⎟⎠
(b) We calculate the power absorded as
P=<E.x>=<eE0cosωt(−ωasin(ωt+φ))>
=eE0.eE0m12.2βω(ω20−ω2)2+4β2ω2.ω=(eE0m)2βmω2(ω20−ω2)2+4β2ω2
This is clearly maximum when ω0=ω because P can be written as
P=(eE0m)2βω(ω20ω2−ω)2+4β2
and Pmax=m4β(eE0m)2 for ω=ω0.
P can also be calculated from P=<γ.x..x>
=(γω2a2/2)=βmω2(eE0/m)2(ω20−ω2)2+4β2ω2.
Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Optics Of Moving Sources
22 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Thermal Equation
47 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Polarization Of Light
43 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
Electric field in EM waves is E=E0sin(kz−ωt)(ˆi+ˆj), then equation of magnetic field is:
View SolutionA free electron is located in the field of a plane electromagnetic wave. Neglecting the magnetic component of the wave distrurbing its motion, find the ratio of the mean energy radiated by the oscillating electron per unit time to the mean value of the energy flow density of the incident wave.
View Solution
Knowledge Check
In an electromagnetic wave, electric field E and magnetic field B are
AMutually perpendicular to each otherBbisect to each otherCboth (A) and (B)Dnone of theseElectric field in EM waves is E=E0sin(kz−ωt)(ˆi+ˆj), then equation of magnetic field is:
AB=B0(−ˆi+ˆj)sin(kZ−ωt)BB=Bc(ˆi+ˆj)sin(kZ−ωt)CB=Bc(−ˆj+ˆk)sin(kZ−ωt)DB=B0(ˆi+ˆj)sin(kZ−ωt)An electron is projected in the magnetic field along the lines of force
AThere will be no effect on the motion of the electron.Belectron speed will increase but the direction will not change.Cthe electron speed will decrease but direction will not changeDnone of these
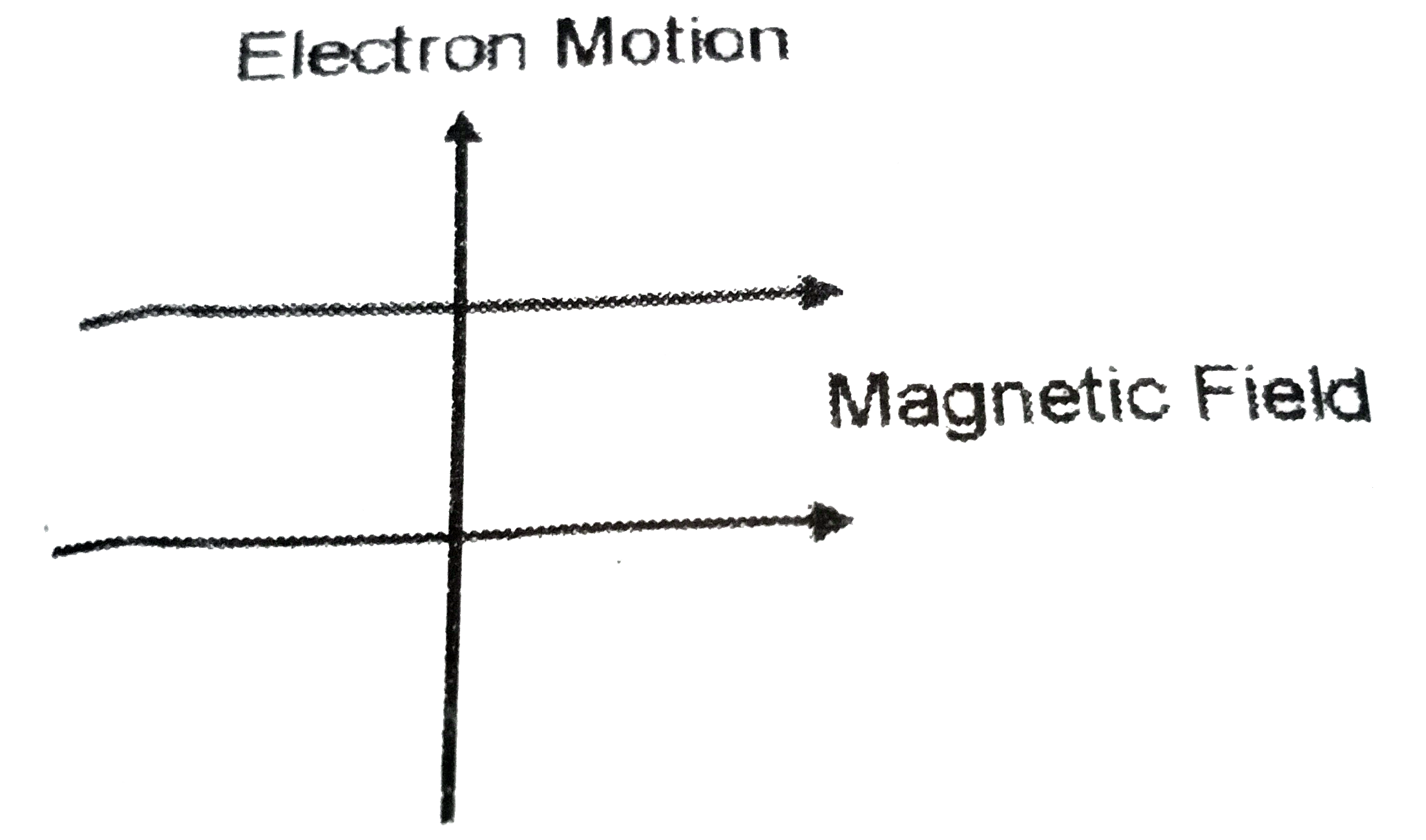
The motion of an electron in presence of a magnetic field is depicted in the figure. Force acting on the electron will be directed
View SolutionAn electromagnetic wave is propagating in vacuum along z-axis, the electric field component is given by Ex=E0sin(kz−ωt). Then magnetic components is
View SolutionThe electric field of an electromagnetic wave travelling through vaccum is given by the equation E=E0sin(kx−ωt) The quantity that is independent of wavelength is
View SolutionAn electromagnetic wave is propagating in vacuum along z-axis, the electric field component is given by Ex=E0sin(kz−ωt). Then magnetic components is
View SolutionThe electric field of an electromagnetic wave travelling through vacuum is given by the equation E=E0sin(kx−ωt) The quantity that is independent of wavelength is
View Solution
