An ideal monatounic gas of mass M is taken through a cyclic process ABC. The process AB is Pp= constant. Totál work done by the gas in the process is −αβMP0P0.
Find (α+β).
'(##CEN_KSR_PHY_JEE_C15_E01_018_Q11##)'
The correct Answer is:5
Topper's Solved these Questions
Similar Questions
One mole of monatomic gas is taken through cyclic process shown below. Temperature at A,TA=300K. The process AB is defined as PT= constant. If the work done in the process AB is (−kR), then find k.(R= Universal gas constant)
'(##CEN_KSR_PHY_JEE_C15_E01_017_Q10##)'
View SolutionIn a cyclic process ABCA then process BC is isothermal. Find work done by gas in cycle ABCA (Given P1=P)
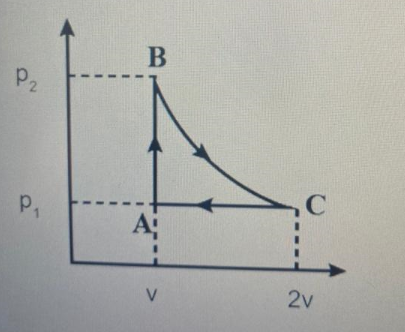
View Solution
Knowledge Check
One mole of an ideal diatomic gas is taken through a process whose P−V diagram is shown in the figure. The work done by the gas is
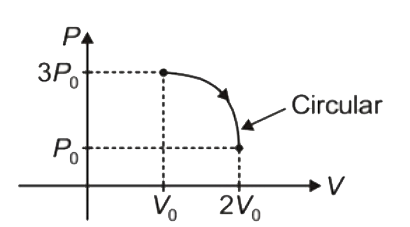 AπP0V0+2P0V0BπP0V02+P0V0C2πP0V0+P0V0DπP0V0√2+P0V0
AπP0V0+2P0V0BπP0V02+P0V0C2πP0V0+P0V0DπP0V0√2+P0V0An ideal gas undergoes cyclic process ABCDA as shown in givend p−V diagram. The amount of work done by the gas is
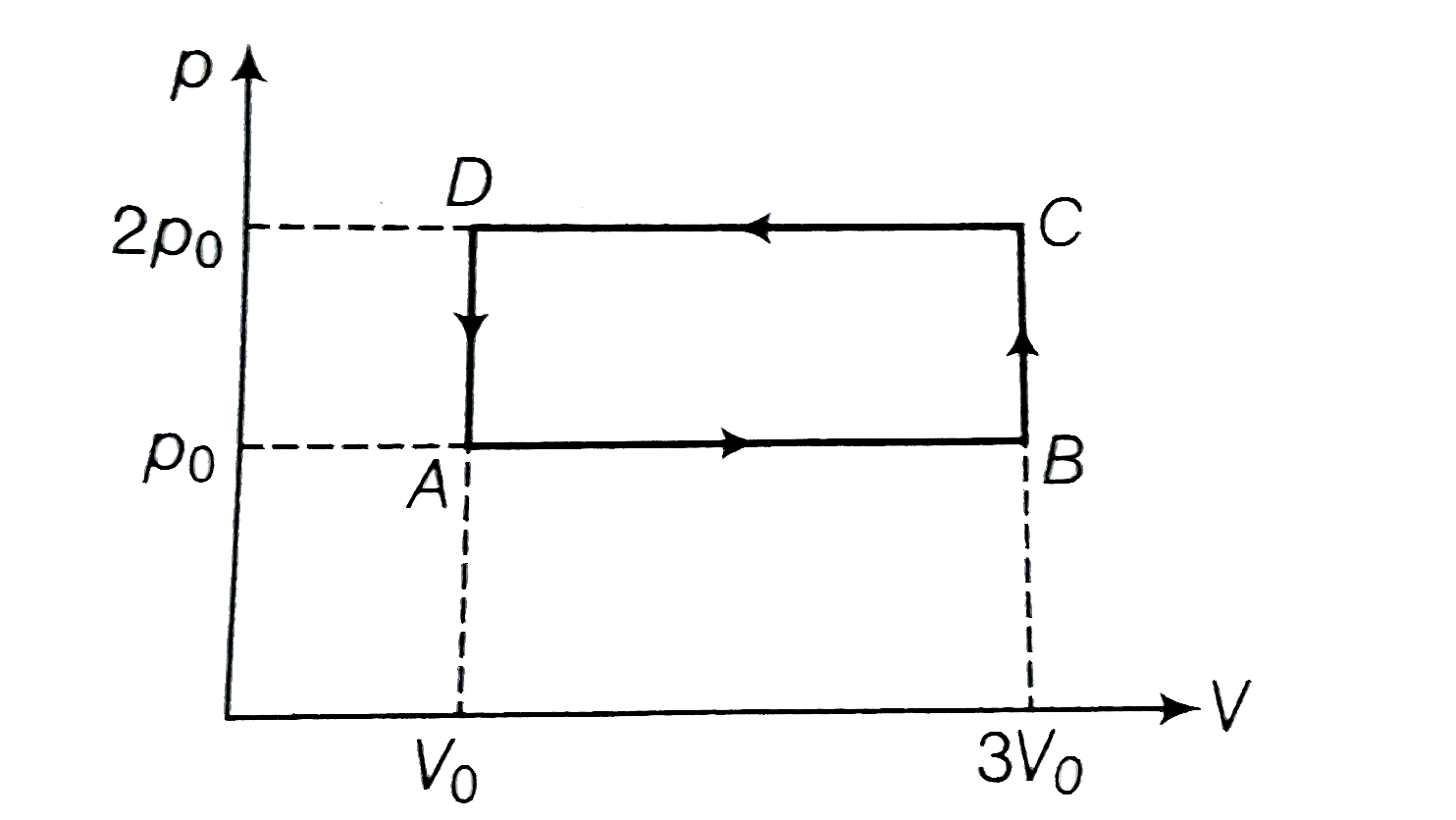 A6p0V0B−2p0V0C+2p0V0D+4p0V0
A6p0V0B−2p0V0C+2p0V0D+4p0V0An ideal gas undergoes cyclic process ABCDA as shown in given p−V diagram. The amount of work done by the gas is
 A6P0V0B−2P0V0C+2P0V0D+4P0V0
A6P0V0B−2P0V0C+2P0V0D+4P0V0
One mole of an ideal diatomic gas is taken through a process whose P−V diagram is shown in the figure. The work done by the gas is

View SolutionAn ideal gas undergoes cyclic process ABCDA as shown in givend p−V diagram. The amount of work done by the gas is

View SolutionPressure and volume of a gas changes from (p0V0) to (p04,2V0) in a process pV2= constant. Find work done by the gas in the given process.
View SolutionPressure and volume of a gas changes from (p0V0) to (p04,2V0) in a process pV2= constant. Find work done by the gas in the given process.
View Solution100 mol of an ideal monatomic gas undergoes the following thermodynamic process as shown in the figure ( P−V or Pressure-Volume plots are shown) A→B: Isothermal expansion B→C Adiabatic expansion
C→D: Isobaric compression
D→A: Isochoric process
The heat transfer along the process AB is 9×104J. The net work done by the gas during the cycle is k×104J. Find k, (Take R=8(~J).(K)−1(~mol)−1 )
'(##CEN_KSR_PHY_JEE_C15_E01_030_Q18##)'
View Solution
