An object of mass 2 kg attached to a spring is moved to a distance x = 10 m from its equilibrium position. The spring constant k=1Nm−1 and assume that the surface is frictionless
(a) When the mass crosses the equilibrium position, what is the speed of the mass?
(b) What is the force that acts on the object when the mass crosses the equilibrium positionSolution in Tamil
(a) Since the spring force is a conservative force, the total energy is constant. At x=10m, the total energy is purely potential
E=U=12KX2=12×(1)×(10)2=50J
When the mass crosses the equilibrium position (x = 0), the potential energy
U=12×1×(0)=0J
The entire energy is purely kinetic energy at this position E=KE=12mv2=50J
The speed v=√2KEm=√2×502=√50ms−1=7.07ms−1
(b) Since the restoring spring force is F lor, when the object crosses the equilibrium position, it experiences no force. Note that at equilibrium position, the object moves very fast. When object is at x=+10m (elongation), the force F=−kx
F=−(1)(10)=−10N. Here the negative sign implies that the force is towards equilibrium ie, towards negative x-axis and when the object is at x=−10 (compression), it experiences a forces F=−(1)(−10)=+10N. Here the positive sign implies that the force points towards positive x-axis.
The object comes to momentary rest at x=10m even though it experiences a maximum force at both these points
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - FULL MARKSCHAPTER - WORK, ENERGY AND POWEREXERCISE - Textual Questions Solved (I. Multiple Choice Question:)
15 Videos
- WORK, ENERGY AND POWERBOOK - FULL MARKSCHAPTER - WORK, ENERGY AND POWEREXERCISE - Textual Questions Solved (II. Short Answer Questions)
5 Videos
- WAVES BOOK - FULL MARKSCHAPTER - WAVES EXERCISE - Additional Questions Solved (Numerical Problems )
10 Videos
Similar Questions
A uniform force of (2ˆi+ˆj) N acts on a particle of mass 1 kg. The particle displaces from position (3ˆj+ˆk) m to (5ˆi+3ˆj) m. The work done by the force on the particle is
View SolutionExplain how the definition of work in physics is different from general perception.
View SolutionThe fundamental frequency of a closed organ pipe is equal to the first overtone of an open organ pipe. If the length of the open pipe is 60 cm, What is the length of the closed pipe?
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
View SolutionThe system is released from rest with both the springs in unstretched positions. Mass of each block is 1 kg and force constant of each spring is 10 N//m . In the equilibrium position, speed of the block placed horizontally is
View SolutionA mass M is attached to a horizontal spring of force constant k fixed one side to a rigid support as shown in figure. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass M is in equilibrium position, another mass m is gently placed on it. When will be the new amplitude of ocillation?
View SolutionA mass of 0.5 kg is hung from a spring. Agradually increasing 0.5 N force is reuired topull the mass downward a distance of 0.25 m from its equilibrium position,if the mass s then released from this position, find
(a) The total energy of the system .
(b) The frequency of the oscillation
(c ) The speed and acceleration of the mass as it passes the equilibrium position.
(d) The speed and acceleration of the mas when the diplacement from equilibrium is 0.25 m
(e) For the initial condition stated, write down the diplacement equation of motion for this mass.
View Solutionस्प्रिंग नियतांक क वाले स्प्रिंग से द्रव्यमान m की एक वस्तु लटके गई है यदि इसे साम्यावस्था से नीचे खींचकर छोड़ जाये तो उसकी गति का आवर्तकाल निकालें
View SolutionA mass 1 kg is attaced to the hook of a spring and the spring is suspended vertically from a ceiling (see Fig. 5.12a). The spring is displaced from its equilibrium position by a distance x. The spring constant of the spring is 2.0×102 N/m. Calculate the displacement x.
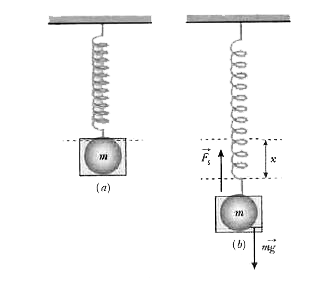
Figure 5-12 (a) Mass of 1 kg attached to a spring is suspended vertically. (b) Spring displace from equilibrium position by a distance x.
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.

View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionIn the given figure, a mass M is attached to a horizontal spring which is fixed on one side to a rigid support. The spring constant of the spring is k. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass is in equilibrium position, as shown in the figure, another mass m is gently fixed upon it. The new amplitude of oscillation will be :

View Solutionएक m द्रव्यमान कि गेंद एक ऊर्ध्वधर स्प्रिंग जिसका बल नियतांक k है के निचले सिरे से जुडी है और स्प्रिंग का ऊपरी सिरा स्थिर छत से जुड़ा है गेंद को विराम अवस्था से छोड़ा जाता है जब स्प्रिंग साम्य लम्बाई में है गेंद x दुरी तय कर दुबारा विराम अव्स्तह में आ जाती है-
View SolutionThe system is released from rest with both the springs in unstretched positions. Mass of each block is 1 kg and force constant of each spring is 10 N//m . In the equilibrium position, speed of the block placed horizontally is
View Solution
