An object of mass 3m splits into three equal fragments. Two fragments have velocities vˆj and vˆi. The velocity of the third fragment is
v(j−i)
v(i−j)
−v(i+j)
v(i+j)√2
The correct Answer is:C
To solve the problem, we need to find the velocity of the third fragment after an object of mass 3m splits into three equal fragments. Given that two fragments have velocities ^vj and ^vi, we can use the principle of conservation of momentum.
1. Identify the Mass of Each Fragment:
Since the total mass of the object is 3m and it splits into three equal fragments, the mass of each fragment is:
m=3m3=m
2. Initial Momentum:
Initially, the object is at rest, so the initial momentum Pinitial is:
Pinitial=0
3. Final Momentum:
After the split, the momentum of each fragment can be expressed as follows:
- For the first fragment (velocity ^vj):
P1=m⋅^vj
- For the second fragment (velocity ^vi):
P2=m⋅^vi
- For the third fragment (velocity →v3):
P3=m⋅→v3
4. Apply Conservation of Momentum:
According to the conservation of momentum:
Pinitial=P1+P2+P3
Substituting the values:
0=m⋅^vj+m⋅^vi+m⋅→v3
5. Simplify the Equation:
Dividing the entire equation by m (since m≠0):
0=^vj+^vi+→v3
6. Solve for →v3:
Rearranging the equation to solve for →v3:
→v3=−(^vi+^vj)
7. Final Expression:
Thus, the velocity of the third fragment is:
→v3=−^vi−^vj
Conclusion:
The velocity of the third fragment is:
→v3=−^vi−^vj
Since the total mass of the object is 3m and it splits into three equal fragments, the mass of each fragment is:
m=3m3=m
Initially, the object is at rest, so the initial momentum Pinitial is:
Pinitial=0
After the split, the momentum of each fragment can be expressed as follows:
- For the first fragment (velocity ^vj):
P1=m⋅^vj
- For the second fragment (velocity ^vi):
P2=m⋅^vi
- For the third fragment (velocity →v3):
P3=m⋅→v3
According to the conservation of momentum:
Pinitial=P1+P2+P3
Substituting the values:
0=m⋅^vj+m⋅^vi+m⋅→v3
Dividing the entire equation by m (since m≠0):
0=^vj+^vi+→v3
Rearranging the equation to solve for →v3:
→v3=−(^vi+^vj)
Thus, the velocity of the third fragment is:
→v3=−^vi−^vj
The velocity of the third fragment is:
→v3=−^vi−^vj
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Taking it together
73 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason
21 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
A bomb initially at rest explodes by it self into three equal mass fragments. The velocities of two fragments are (3ˆi+2ˆj) m/s and (–ˆi–4ˆj) m/s. The velocity of the third fragment is (in m/s)-
View SolutionA grenade having mass of 10 kg flying horizontally with a velocity of 10 m/s explodes into two fragments. The larger fragment has a velocity of 25 m/s in the direction of the intial velocity of the grenade. The smaller fragment has a velocity of 12.5 m/s in the opposite dirction. The masses of the fragments are
View SolutionA projectile is fired with velocity.v0 at an angle 60∘ with horizontal . At top of its trajectory it explodes into two fragments of equal mass. If one fragment retraces the path then the speed of the other fragment is
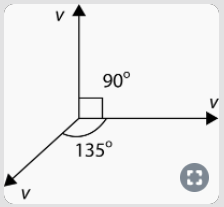
View SolutionA particle of mass 4m which is at rest explodes into four equal fragments. All four fragments scattered in the same horizontal plane. Three fragments are found to move with velocity v as shown in the figure. The total energy released in the process is
View SolutionAt a certain height a shell at rest explodes into two equal fragments. One of the fragments receives a horizontal velocity u. The time interval after which, the velocity vectors will be inclined at 120∘ to each other is
View SolutionAt a certain height a body at rest explodes into two equal fragments with one fragment receiving a horizontal velocity of 10ms^−1. The time interval after the explosion for which the velocity vectors of the two fragments become perpendicular to each other is
View SolutionAt a certain height a body at rest explodes into two equal fragments with one fragment receiving a horizontal velocity of 10ms−1. The time interval after the explosion for which the velocity vectors of the two fragments become perpendicular to each other is (g=10ms−2)
View SolutionA shell in flight explodes into n equal fragments k of the fragments reach the ground earlier than the other fragments. The acceleration of their centre of mass subsequently will be
View SolutionA bomb falling freely bursts after 10 sec. (g=10ms2) into two fragments of masses in the ratio of 2:1. The velocity of heavier fragment immediately after the explosion is 200ms−1 vertically downwards. The velocity of the lighter fragment immediately after the explosion is
View SolutionAn object initially at rest explodes into three equal fragments A,B and C.The momentum of A is pˆi and that of B is √3pˆj
where p is a+ve number. The momentum of C will be
View Solution
