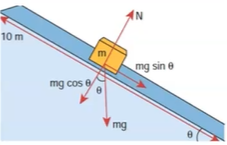
An object of mass $m = 1 k g$ is sliding from top to bottom in the frictionless inclined plane of inclination angle $θ = 30^{\circ}$ and the length of inclined plane is 10m as shown in the figure. Calculate the work done by gravitational force and normal force on the object Assume acceleration due to gravity, $g = 10 m s^{- 2}$
Solution in Tamil

Video Solution

Text Solution

Verified by Experts

We calculated in the previous chapter that the acceleration experienced by the object in the inclined plane as $g sin θ$
According to Newton.s second law, the force acting on the mass along the inclined plane $F = m g sin θ$ . Note that this force is constant throughout the motion of the mass The work done by the parallel component of gravitational force $(m g sin θ)$ is given by $w = \to F . d \to r = F d r cos ϕ$
where is the angle between the force $(m g sin θ)$ and the direction of motion $(d \to r)$ . In this case, force $(m g sin θ)$ and the displacement $(d \to r)$ are in the same direction. Hence and $cos ϕ = 1$
$w F d r = (m g sin θ) (d r)$ (dr=lenght of the inclined plane)
$w = 1 \times 10 \times sin (30^{\circ}) \times 10 = 100 \times \frac{1}{2} = 50 J$ The component ing cos 6 and the normal force N are perpendicular to the direction of motion of the object, so they do not perform any work.

|

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

FULL MARKS-WORK, ENERGY AND POWER-Additional Question Solved (Numercials)

Home
Ask Doubt
Profile