As shown in Fig. the two sides of a step ladder BA and CA are 1.6m long and hinged at A. A rope DE,0.5m is tied half way up. A weight 40kg is suspended from a point F,1.2m from B along the ladderBA. Assuming the floor to be fricionless and neglecting the weight of the ladder, find the tension in the rope and forces exerted by the floor on the ladder. (Take g=9.8m/s2)
(Hint. Consider the eqilibrium of each side of the ladder separately.)
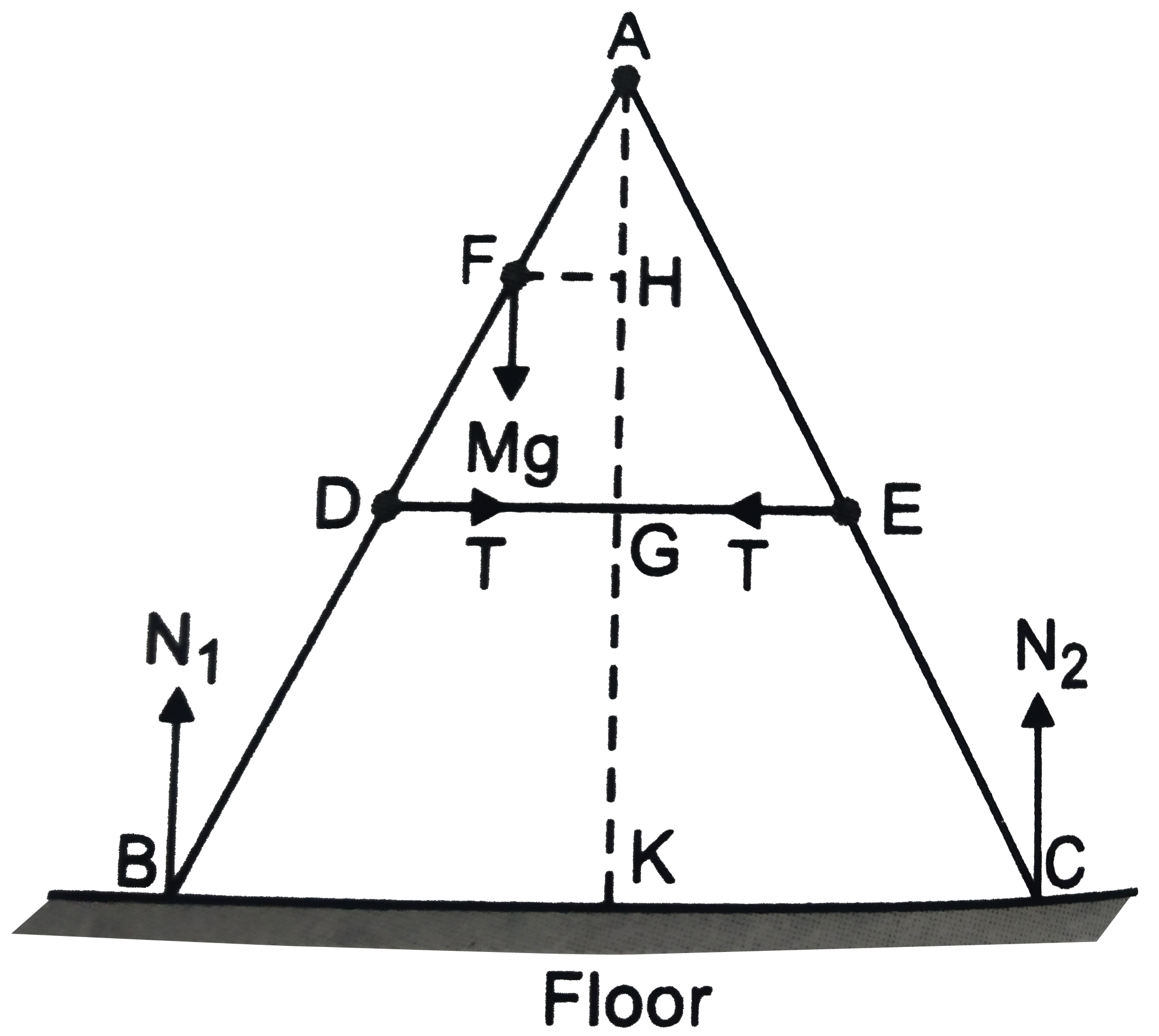
The forces acting on the ladder are shown in Fig. 7.14. Here, IV=40kg=40×9.8N=392N,AB=AC=1.6m,BD=1/2×1.6m=0.8m,BF=1.2m andDE0.5m
In the Fig. ΔADE and ΔABC are similar triangles, hence
BC=De×ABAD=0.5×1.60.8=1.0m
Now, considering equilibrium at point B ∑τ=0
∴ W×(MB)=NC×(CB)
But MB=KB×BFBA=0.5×1.21.6=0.375m
Substituting this value in (i), we get
∴ NC=W×(MB)(CB)=392×0.3751=147N
Again considering equilibrium at point C in similar manner, we have
W×(MC)=NB×(BC)
∴ NB=W×(MC)(BC)=W×(BC−BM)(BC),
(392×(1−0.375))/1=245N
Now, it can be easily shown that tension in the string T=NB−NC=245−147=98N.
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - NCERT ENGLISHCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISE
33 Videos
- PHYSICAL WORLD BOOK - NCERT ENGLISHCHAPTER - PHYSICAL WORLD EXERCISE - EXERCISE
16 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXERCISE
22 Videos
Similar Questions
The step-ladder shown in figure 6.11 is 8 m long and hinged at C.BD is a tie rod 2.5 m long half way up. A man of 86 kg climbs 6 m along the ladder . Find the tension in the tie rod the forces exerted on the ladder by the assuming the floor to frictionless and the ladder weightless.

[Hints: Consider the free-body diagram of the sides of the ladder.]
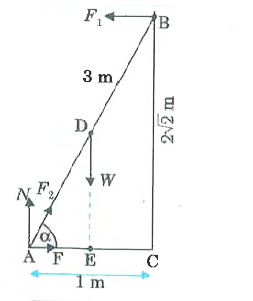
View SolutionA 3 m long ladder weighing 20 kg leans on a frictionless wall. Its feet rest on the floor 1 m from the wall as shown in the following figure, Find the reaction forces of the wall and the floor.
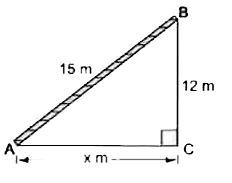
View SolutionA 15-m long ladder is placed against a wall to reach a window 12 m high.Find the distance of the ladder from the wall.
View SolutionA ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from the base of the wall.
View SolutionA 6.5 m long ladder rests against as vertical wall reaching a height of 6.0 m. A 60 kg man stands hlf way up the ladder. A. Find the torque of the force exerted by the man on the ladder bout the upper end of the ladder. b.Assuming the weight of the ladder of be negligible as compared to the man and assuming the wall to be smooth find the force exerted by the ground on the ladder.
View SolutionA ladder 3.7 m long is placed against a wall in such a way that the foot of the ladder is 1.2 m away from the wall. Find the height of the wall to which the ladder reaches.
View SolutionA ladder makes an angle of 30∘ with a wall. If the foot of the ladder is 5 m away from the wall, find the length of the ladder.
View SolutionA ladder 13 m long rests against a vertical wall. If the foot of the ladder is 5 m from the foot of the wall, find the distance of the other end of the ladder from the ground.
View SolutionA ladder 13 m long rests against a vertical wall. If the foot of the ladder is 5 m from the foot of the wall, find the distance of the other end of the ladder from the ground.
View SolutionA ladder 10m long reaches a window 8m above the ground. Find the distance of foot of the ladder from base of the wall.
View Solution
