As shown schematically in the figure , two vessels contain water solutions (at temperature T) of potassium permanganate (KMnO4) of different concentrations n1andn2(n1>n2) molecules per unit volume with Δn=(n1−n2<<n1. When they are connected by a tube of small length and cross - sectional area s, KMnO4 starts to diffuse from the left to the right vassel through the tibe Consider the two collection of molecules to between as dilute ideal gases and the difference in their partial pressure in the two vassels causing the diffusion . The speed v of the molecules is limited by the viscous force −βv on each molecule, where β is a constant . neglecting all terms of the order (Δn)2 which of the following is / are correct ? (kB is the Boltzmann constant)

the force causing the molecules to move across the tube is ΔnkaTS
force balance implies n1βvl=ΔnkBT
total number of molecues going across the tube per sec is (Δnl)(kBTβ)S
rate of molecules getting transferred through the tube does not change with time
The correct Answer is:A, B, C
(A) 
F=ΔP.A=(P1−P2)A
=n1×RTA−n2RT.A
F=Δn.RT.A
(B) βv×(n1×A×l)=Δ.T.A
β.v.n1.l=Δn.k.T
(C ) dNdt=s×v×n1
=s.n1×Δn,K,Tβ.n1l=S×Δn.K.TSβ.l
(D) Δn→ decreasing then dNdt→ decreasing
Topper's Solved these Questions
- BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCED 2020EXERCISE - SECTION-36videos
JEE ADVANCED 2020
- BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCED 2020EXERCISE - SECTION-36videos
JEE ADVANCED 2020
- BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCEDEXERCISE - PHYSICS SECTION - IV Matrix Match Type2videos
JEE ADVANCED
- BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCED 2021EXERCISE - QUESTION38videos
JEE ADVANCED 2021
Similar Questions
Put a unifrom meter scale horizontally on your extended index fingers with the left one at 0.0 cm and the right one at 90.00 cm when you attempt to move both the fingers slowly towards the center , initially only the left finger slips with respect to to the scale and the right finger does not . after some distance, the left finger stops and the right one starts slipping . Then the right finger stops at a distance XR from the center (50.00 cm ) of the scale and the left one starts slopping again . This happens because to the difference in the fiictional forces on the two fingers . it the coefficients of static and dynamic between the fingers and the scale are 0.4 and 0.32 respectively , the value of XR (in cm is ...........
06:15View SolutionPut a unifrom meter scale horizontally on your extended index fingers with the left one at 0.0 cm and the right one at 90.00 cm when you attempt to move both the fingers slowly towards the center , initially only the left finger slips with respect to to the scale and the right finger does not . after some distance, the left finger stops and the right one starts slipping . Then the right finger stops at a distance XR from the center (50.00 cm ) of the scale and the left one starts slopping again . This happens because to the difference in the fiictional forces on the two fingers . it the coefficients of static and dynamic between the fingers and the scale are 0.4 and 0.32 respectively , the value of XR (in cm is ...........
02:45View SolutionOne mole of a monatomic ideal gas is taken through a cycle ABCDA as shown in the P-V diagram. Column II gives the characteristics involved in the cycle. Match them with each of the processes given in Column I.
 04:53View Solution
04:53View SolutionThe smallest division on the main scale of a vernier calipers is 0.1cm. Ten divisions of the vernier scale correspond to nine divisions of the main scale. The figure below on the left shows the reading of this calipers with no gap between in two jaws. The figure on the right shows the reading with a solid sphere held between the jaws. The correct diameter of the sphere is.
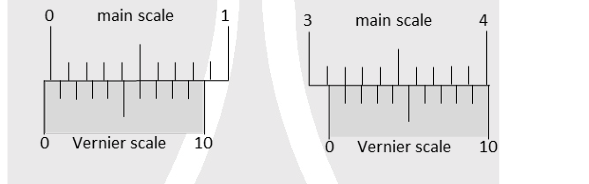 03:33View Solution
03:33View SolutionLet n1<n2<n3<n4<n5 be positive integers such that n1+n2+n3+n4+n5=20. then the number of distinct arrangements n1,n2,n3,n4,n5 is
01:22View SolutionTwo perfect gases at absolute temperature T_(1) and T_(2) are mixed. There is no loss of energy. The masses of the molecules are m_(1) and m_(2) . The number of molecules in the gases are n_(1) and n_(2) . The temperature of the mixture is
03:41View SolutionTwo perifect gases at absolute temperatures T1andT2 are mixed. The absolute temperature of the mixture is T. there is no loss of energy.If m1andm2 are masses of molecules and n1andn2 are number of molecules, then
02:03View SolutionIf n2−11n+24=0 is satisfied by n1&n2 where n2>n1 then (A) n21+n2 is prime number (B) n1&n2−n1 are co-prime (C) n1&n2−n1 are twin primes (D) n1+n2+n1n2 has 2 prime divisors
11:32View SolutionTwo gases A and B at same pressure contain number of moles n1andn2. Their volume temperature graphs are shown in figure. Then the ratio
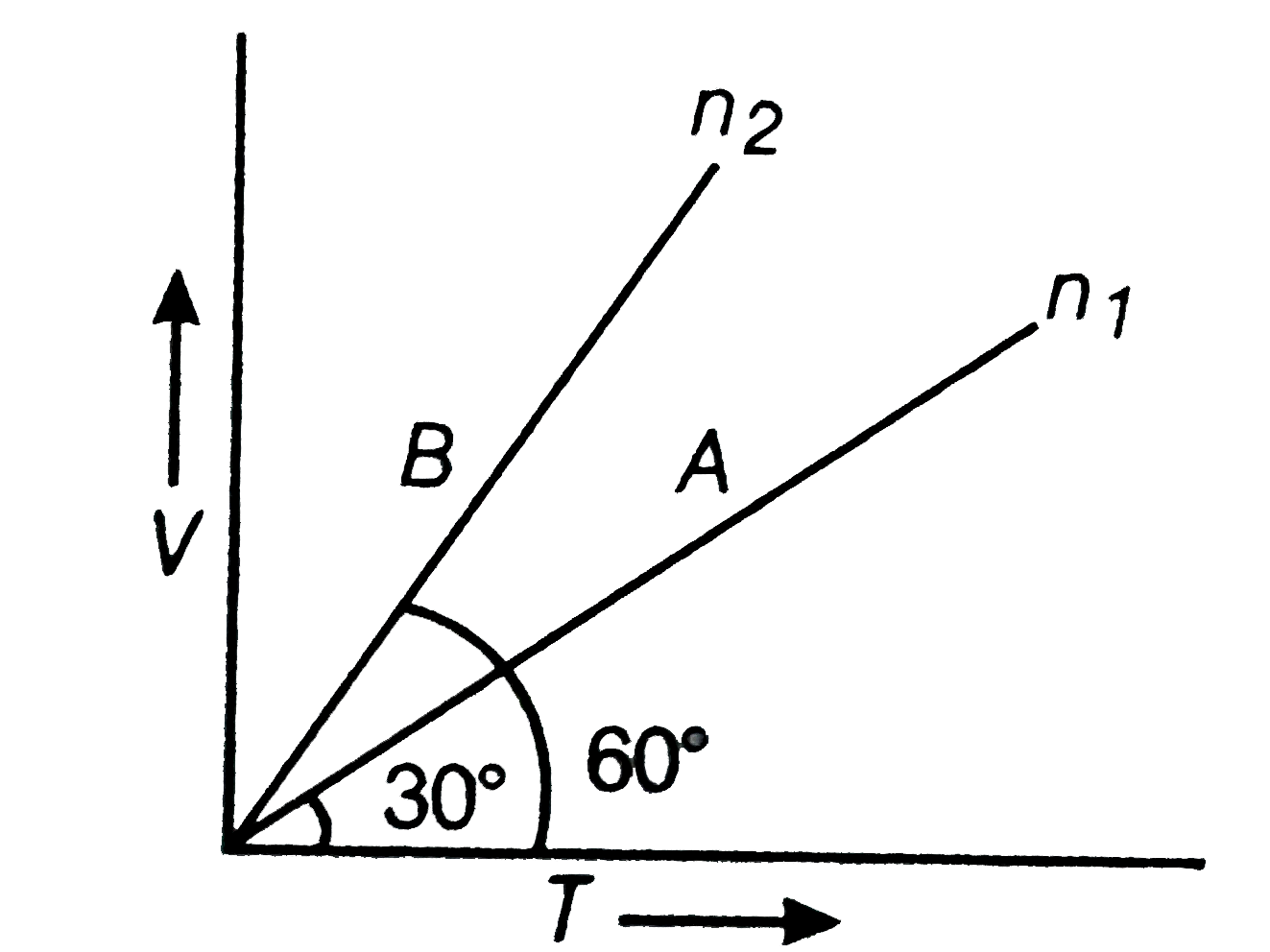 03:56View Solution
03:56View SolutionFind the mutual inductance of two long concentric solenoids for a length I. The area of cross-section of inner solenoid is A and number of turns per unit length are n1 and n2.
03:12View SolutionWhen a transition of electron in He+ takes place from n2 to n1 then wave number in terms of Rydberg constant R will be
(Given n1+n2=4,n2−n1=2)06:22View Solutionएक पतला उतल लेंस दो प्रदार्थों से मिलकर बना हैं, जिनके अपर्वतनाक (Refractive index) क्रमशः n1 और n2 है| लेंश के बांये और दांये पृष्ठों की वक्रता त्रिज्याए समान है n1=n2=n के लिए लेंस की फोकस दूरी f है| जब n1=n और n2=n+Δn है, तब फोकस दूरी f=Δf है| यह मानते हुए कि Δn<(n−1) और 1<n<2 है निम्नलिखित कथानो में से कौन-सा(से) सही है(है)
02:43View SolutionTwo frequencies whose frequencies n_(1) and n_(2) Huh, n_(1), 15cm With COP and n_(2) 30.5 cm If resonated with OOP n_(1), n_(2) will be honoured. n_(1) and n_(2) There is a relation of two lashes per second.
05:55View SolutionIn this spectrum of Li2+ the difference of two energy level is 2 and sum is 4. Find the wavelength of photon for difference of these two energy state.
(note n1+n2=4 and n2=2 so take n1=1 and n2=301:53View Solutionयदि n2−11n+24=0,n1 और n2 जहाँ n2>n1 से संतुष्ट होता है तब
03:05View Solution

